Updated: December 28th, 2024
Jun Sato Laboratory + Jun Sato Structural Engineers Co., Ltd.
������w�����~������ + �����~�\���v������
Transparent Structures providing Komorebi Naturalness
�X�ɐ��ތ��ɐ��� /�u������сv�ޓ��ߐ�����\���`��
Essay Contents
�� Overview
�� Simple Optimization / Simple Form Finding
�� Structural Optimization Algorithm
�� Similarity between Form Optimization and Deep Learning
�� Sakura Dimples: Local Optimization Applicable to Lunar / Martian Base Camp
�� Energy Distribution as an Objective Function
�� Contraction Method in Buckling Eigenvalue Equation
�� Timber Joinery for Free Aggregated Form
�� 1/f fluctuation / 1D Spectrum Analysis
�� 2D Power Spectrum in Creating Komorebi Naturalness
�� Geometry to Provide Natural Forms / Flexible Angle Kigumi Joinery
�� Geometry to Provide Natural Forms / Free 3D Metal Form
�� Geometry to Provide Natural Forms / Fuzzy Node Algorithm
�� Multi-objective Optimization
�� Super Lightweight Paper & Fabrics / Quick Establishment
�� Lightweight and Ductile Structure: Little by little, learning Great Nature
�� Super Lightweight Structures
�� Membrane Tensegrity / Omission Technique of Engineering
�� Glass Shop
�� Lattice3 = Lattice�~Lattice�~Lattice
�� Concealed Structural Design
�� Metal Shop
�� Wood Shop
�� Free Form Using Excavated Ground
�� Structural Analysis Model Tune-up
�� 3D Tensegrity / Pop-up Tectonics
�� International Seminar & Workshop
�� Lectures
�� Publications
Transparent Structures providing Komorebi Naturalness
�X�ɐ��ތ��ɐ��� /�u������сv�ޓ��ߐ�����\���`��
Jun Sato
Jun Sato Structural Engineers Co., Ltd.
Associate Professor, University of Tokyo
http://junsato.k.u-tokyo.ac.jp/
���� �~ / �����~�\���v������, ������w�y����






�� Overview
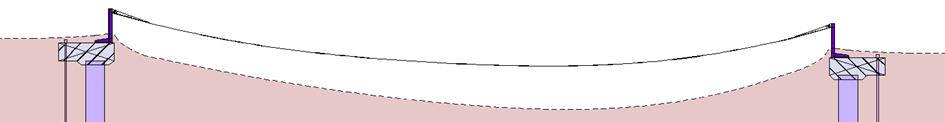
Architectural structures composed with slender elements let us notice that transparent structures have potential to serve as environmental filters to create Komorebi space.
Using multi-objective optimization, those structures prevent death in the event of collapse while providing natural space.
���ׂ��ނŌ`�����ꂽ���ߐ�����\���`�Ԃ͊��𐧌䂷���u�t�B���^�[�v�Ƃ��Ă̖����������A�u������сv�̂悤�ȃi�`�������ȋ�Ԃݏo�����̂ƂȂ邱�Ƃ��ł��܂��B
�͊w�ƌ������ɍœK�������u���ړI�œK���v�ł���Ȍ`�Ԃ����ݏo����܂��B
���܂����u���ړI�œK���v�̎��オ�K��Ă��܂��B
�������u���Ă����ȂȂ��\���v�ݏo�����Ƃ��ł������ł��B



Komorebi : Sunlight comes through leaves in the woods.
Sazanami : Ocean ripple, containing acoustics.
Seseragi : River stream, containing acoustics, cool breeze, humidity, smell of moss, and Komorebi.
���{�ɂ͑f�G�Ȍ��t������܂��B
�u������сv�u�����Ȃ݁v�u�����炬�v
�u�����炬�v�́A�������łȂ����A���x�A�C���A���C���D��Ȃ���Ԃł��B
We should compose a morphogenetic operation for those targets through these practices:
Dynamical Operations / Geometrical Operations / Workshop Scale Experiments
Using these operations, we would be able to develop more morphogenetic forms based on geometry, materials, dynamics, craftsmanship, site situations, and energy consumption.
Engineering is a technique of omission. We still confront unidentified phenomena. We never have enough time to try all the calculations we desire. We try to suppose a simplified model with fewer calculations and figure out if the structure is feasible. This aspect has to be acknowledged by society.
������u�����^�������v�ȍ\���`�Ԃݏo�����߂ɁA
�u�͊w�`�Ԃ̐���v�u�w�`�Ԃ̐���v�u���[�N�V���b�v�X�P�[���ł̎��H�v
��ʂ��Č`�Ԑ������u�v�@�v���\�z���邱�Ƃ�ڎw���܂��B
���̎�@��p���āA�w�A�ޗ��A�͊w�A�E�l�Z�A������A����G�l���M�[�A�Ɋ�Â��`�Ԃݏo�����Ƃ��ł��܂��B
�G���W�j�A�����O���u�ȗ��̋Z�v�ł��B
�����𖾂���Ȃ����ۂ����钆�ŁA����ꂽ���ԂƔ�p�̒��ŁA���f���Ă����̂��ƎЉ��F�������K�v������܂��B
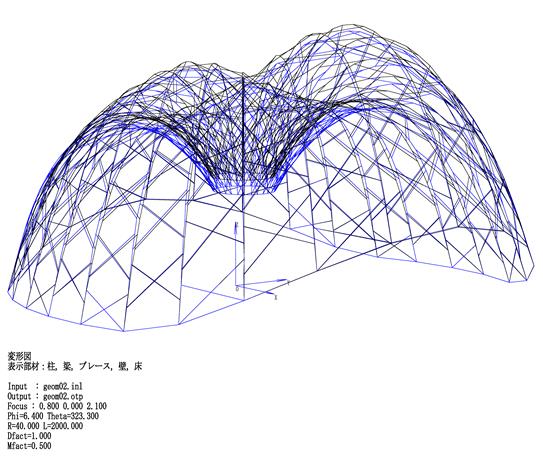
�� Simple Optimization / Simple Form Finding
Park Groot Vijversburg, Netherlands, 2017
Visitor Center in Vijversburg
�t�@�C�o�[�X�u���O�r�W�^�[�Z���^�[
Architect: Junya Ishigami, Marieke Kums / studio MAKS
Structural advisor: Jun Sato
Engineering: ABT
Published: MARK #54, 2015 / Shinkenchiku, September 2017
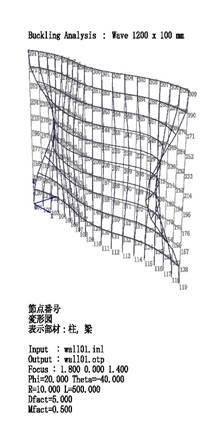
A curved glass wall structure with a thickness of 1cm is provided by manipulating the buckling phenomenon and load distribution.
�Ȗʏ�̃K���X�ǂ̍\���B
�������x�Ɖd�z�����œK�����Č���1cm�łł��Ă��܂��B



Buckling strength is manipulated by curvature. (Photo: Jun Sato)
Glass walls work as shear walls against wind load.
���ɋȗ���^����ƍ����ɋ����Ȃ邱�Ƃ����p���Ă��܂��B
�K���X�ǂ͕��ɂ�鐅���͂ɂ���R���܂��B
(No Image)
Junya Ishigami�fs sketch
http://www.designboom.com/architecture/junya-ishigami-associates-maks-park-groot-vijversburg/
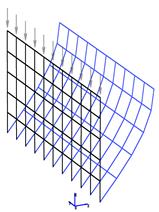

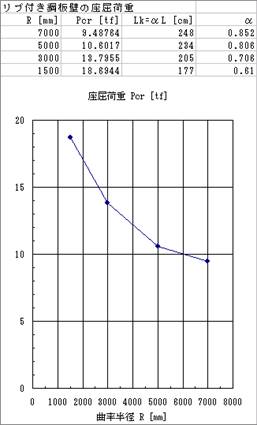
3D buckling analysis on curved thin wall
Radius – Buckling Strength Diagram
���ɋȗ���^����ƁA���{���������邱�Ƃ��ł��܂��B
The brainstorming with Junya Ishigami takes 5 to 8 hours on each day.
Through detailed thinking against each phenomenon, a new structural idea sometimes comes up.
�Ώコ��ƈĂ����Ƃ��́A5�`8���Ԃ��̃u���[���X�g�[�~���O�����x���J��Ԃ��܂��B
�������Č��ۂJ�ɕ��͂��Ă��邤���ɁA�A�C�f�A�����܂��Ƃ�������܂��B
Glass walls and beams construction (Photo: Marieke Kums)
Screenshot of form optimization software to manipulate load distribution
The load distribution is manipulated by arrangement of beams.
�d�z���́A���̔z�u�ɂ���ăR���g���[���ł��܂��B
�u�ǂ̋ȗ��v�u���̔z�u�v�Ƃ���2���u�w�p�����[�^�[�v���������čœK�����܂����B
Construction of curved glass walls (Photo: Marieke Kums)
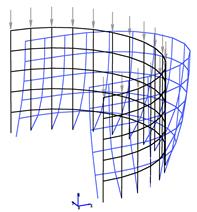
KAIT Plaza, 2020
KAIT�L��
Architect: Junya Ishigami
Structure: Yasutaka Konishi + Jun Sato + Kazuyuki Ohara
Contractor: Kajima Co., Ltd.
Steel Roof Construction: Shiraishi Steel Co., Ltd.
Steel Wall Construction: Nichinan Steel Co., Ltd.
2-way catenary thin steel sheet with a thickness of 12mm is spans 50�~80m while sagging 4m.
Allowing a bit of compression, the concaved shape on the corners could become curved in low curvature which provides higher space and simple manufacturing.
12mm�̍|��80�~50m�̌����������ł��܂����B
�䂪�l�ӌ`�̃G�b�W����2�����ȗ��������Đ��ꉺ����܂��B

Rain water as well as sunlight comes in from the openings.
�g�b�v���C�g�ɂ̓K���X���Ȃ��̂ʼnJ�������Ă��܂��B

���̂ق��ł͏��ʂ����炩�ɂ���オ���Ă��܂��B

�����}�^�������}
�����̉���3m�ɂ͍����~�߂̃��u����ׂĂ��܂��B
�Z��50m�̎��g�}
������4m����Ă��܂��B
�����͋���ɕǂ�������܂��B
��b��傫�����A�[�X�A���J�[�ŗ}�����݂܂��B
�|�T���h�C�b�`�p�l���̕ǂ̑����̓A���J�[�{���g����߂�ƈ����߂���悤�ɂ��Ă���܂��B

�|�T���h�C�b�`�p�l���̕ǂ̊O��
�����ɂ̓g�b�v���C�g���U�݂��Ă��܂��B

The catenary shape was determined using the Co-rotation method,
which is one of the nonlinear analysis algorithms.
�l�ӌ`�̌����Ȗʂ͎l���ł��Ȃ�~���`�ɍi��K�v������ƕ�����܂����B
����ł͓V�䂪�Ⴍ�Ȃ�̂Ɖ��H���ɂ����̂ŋȗ����ɂ߂܂��B
����ƈ��k���������Ă��܂����Ƃ�������܂����B

�F�����^�ԁF���k

����������������ɒ����Ɉ��k���������܂��B

�~�G�Ɏ��k����Ƃ��͋����ł��̈��k�����債�Č������Ȃ�܂��B

Buckling mode in summer
In summer, the compression stress increases in the middle of the edges.
�ċG�̉��͏�Ԃɑ���������[�h
�ċG�ɂ͎l�ӂ̒����ň��k�����傷��̂��������Ȃ�܂��B


Steel roof construction
Around 200 sheets of steel are assembled and full-penetrated.
�|��~�����I����������^�v�H��

�ǂ̑�����}�����ޑ�ʂ̃A���J�[�{���g�^�|�T���h�C�b�`�ǂ̗n��


�����̉����̃��u�^�g�b�v���C�g�̊J���⋭�̗n��


�J���⋭�^�����̕����I�Ȗc��݂����������A�u��̐�
�J���̉��̓t���b�g�o�[�ŕ⋭���܂����B
�X�J���b�v��r=35mm�Ȃ̂ɏK���ē�����r=50mm�Ƃ��܂����B
�K�X�o�[�i�[�ŃA�u����@�͍�������ɋ����Ă��������������ł��B


�W���b�L�_�E������

�� Structural Optimization Algorithm
�͊w�I�œK���̃A���S���Y��
Community Centre in Kawatana Onsen, Shimonoseki, Japan, 2010
Architect: Kengo Kuma
Structure: Jun Sato
��I����𗬃Z���^�[
���z�ƁF�G ����
�\���F���� �~


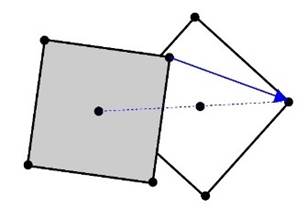
Multifaceted form optimized by adjusted position of nodes.
��I����𗬃Z���^�[ (���z�ƁF�G����) �̎R���݂̂悤�ȑ��ʑ�
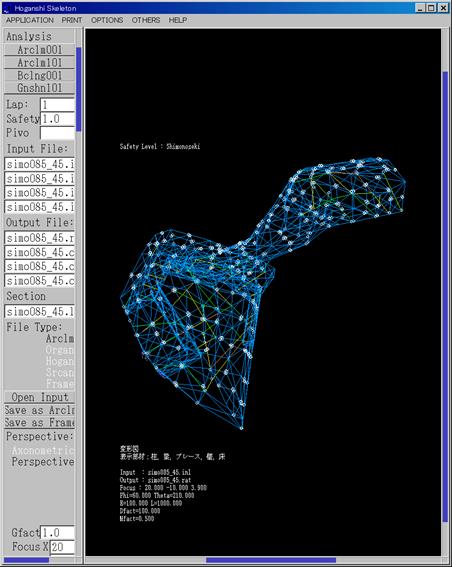
�u�͊w�I�`��œK���v�̓T�^�I�ȑΏۂ̈�����ʑ̂ł��B
���ʑ̂̓ʉ��̋���œK�����܂��B
Development of algorithm for form finding under several load scenarios:
Gravity
Gravity + Seismic load X
Gravity + Seismic load Y
Gravity + Wind load X
Gravity + Wind load Y
Self-weight + Snow
The structural performances as the objective functions:
Safety ratio due to material strength
Safety ratio due to buckling phenomenon
Distribution of energy absorption
This software has automatic execution option which will substitute for Macro or Plug-in of some specific 3D modeling software.
�u�d�͂̂݁v�u�d�́{X�����n�k�v�u�d�́{Y�����n�k�v�Ȃ��u�����̉d�p�^�[���v�ɑ��Č`�Ԃ��œK������A���S���Y���̒�Ăł��B
���S���A�������x�A�z���G�l���M�[�Ȃǂ��͊w����u�ړI���i�]���w�W�j�v�ƂȂ�܂��B
�������s�@�\����������ƁA�`���ʂ̃\�t�g�E�F�A�Ɉˑ����āA���̃\�t�g�E�F�A������s���邱�Ƃɂ�����u�}�N���v���u�v���O�C���v�Ɍ�������̂Ƃ��Ďg�p���邱�Ƃ��ł��܂��B
Polyhedral form for Community Centre in Kawatana Onsen, Architect: Kengo Kuma
Safety ratio color chart is displayed while morphing the shape by mouse.
�܂��`�����ʏ�Łu�}�E�X�ɂ��蓮����v�łł����u�蓮�`�ԉ�́v�̃\�t�g�E�F�A������Ă݂܂����B
���e���͓x�ɑ����u���S���i�����j�v���w�W�ɂ��āA�e�d�p�^�[������Z�o����錟���̂����A�ޖ��ɍő�l�݂̂�\�����܂��B�S�Ă̕��ނŌ���䂪 1.0 ���Ȃ��`��������邱�Ƃ��ڕW�ɂȂ�܂��B�d�͂�n�k�A�ϐ�A���ȂǕ����̉d���u�����]���v���Č`����œK�����邱�Ƃɑ������܂��B
Any geometrical parameters from any shape, such as Free Surface, Stacked Clusters, Branched Tree, Randomly Located Columns, can be selected to transform the shape.
�u���R�Ȗʁv�u�ϑw�v�u����v�u���i�X���u�v�u�ї����钌�v�Ȃǂǂ̂悤�Ȍ`����w�I�p�����[�^�[��I��œK�p�ł��܂��B

Polyhedral mesh for Naoshima Pavilion, Kagawa, 2015, Architect: Sou Fujimoto
�����p�r���I�� (���z�ƁF���{�s��) �̗n�ڋ��Ԃɂ�铧�������鑽�ʑ�
Free surface for House of Pease HOPE, Copenhagen, Architect: Junya Ishigami
�œK���̓T�^�I�ȑΏۂ̈�Ɏ��R�Ȗʂ�����܂��B

Twisted ribbon for Cloud Arch, Sydney, 2014~, Architect: Junya Ishigami
Geometrical Parameters: winding shape, twisting angle
���{�����ɕ����悤�ȃ^���[�ł��B
�u3�����Ȑ��v�ƃ��{���́u�Ђ˂�p�v���w�I�p�����[�^�[�ɂ��čœK�����܂����B
�p�����[�^�[�����Ȃ����肷��̂��r�̌����ǂ���ł��B
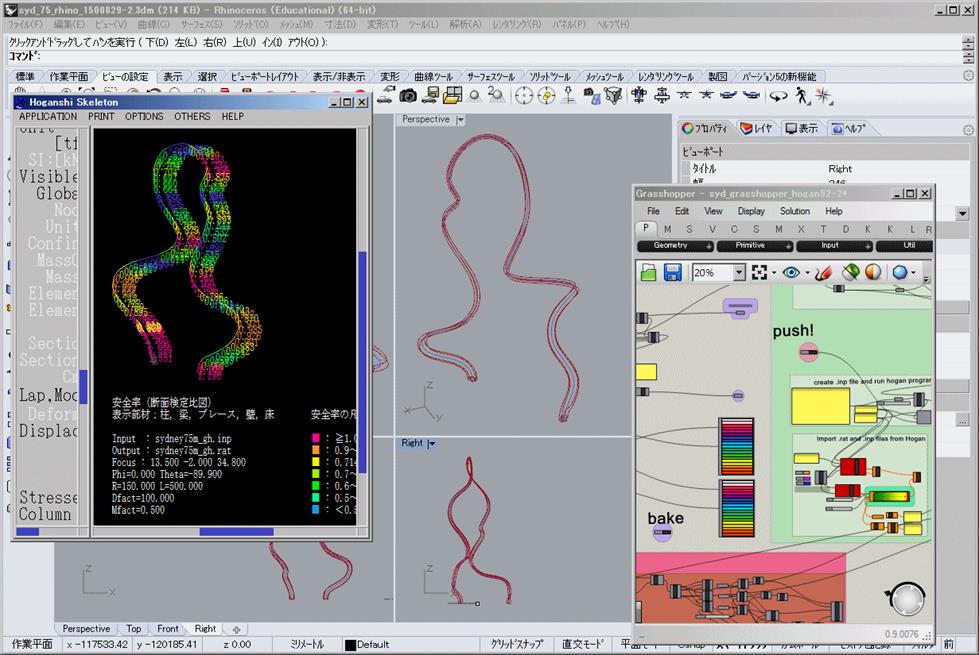
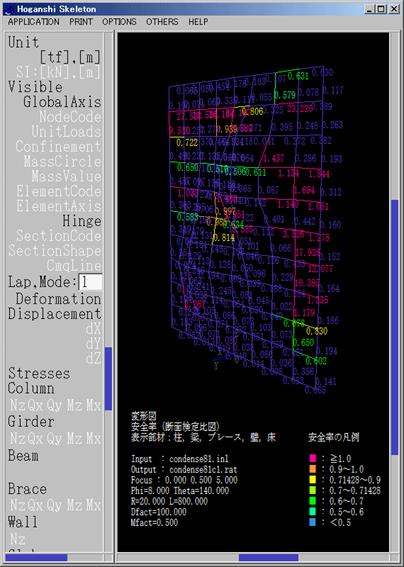
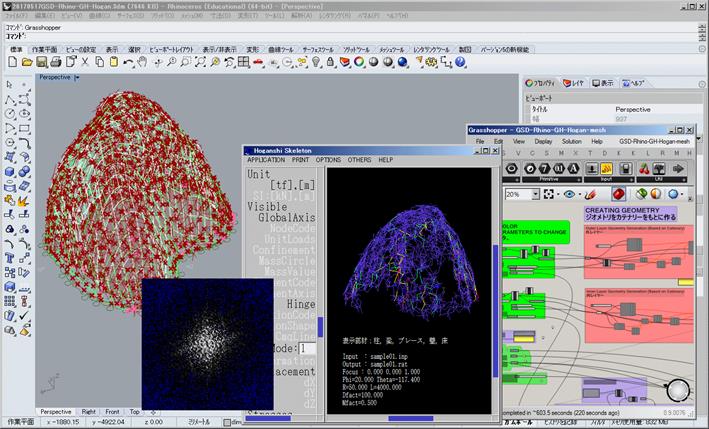
Form Optimization Software Component: Hogan + Rhinoceros + Grasshopper
Rhinoceros + Grasshopper + Python + Hogan �ɂ��\�t�g�E�F�A�R���|�[�l���g
�`���ʂ̃\�t�g�E�F�A�Ɉˑ����ăv���O�C���̂悤�Ɏg�p���邱�Ƃ��ł��܂��B
����̃\�t�g�E�F�A�̃v���O�C���Ƃ��ăv���O���~���O���Ȃ��Ă悢�\���@�Ƃ��Ē�Ă��Ă��܂��B
Stacking free curved wall, Architect: Sou Fujimoto
Genus of topology is also optimized in this case
�A���[�o�`�{�����[���̐ϑw�`�� (���z�ƁF���{�s��)


Free level floors for House NA, Architect: Sou Fujimoto
House NA (���z�ƁF���{�s��) �̑��i�X���u

Branched tree, Architect: Sou Fujimoto
Randomly located columns for Extreme Nature, Architect: Junya Ishigami
����`�� (���z�ƁF���{�s��)
Extreme Nature �̗ї����钌 (���z�ƁF�Ώ㏃��)
�� Automated Optimization
�œK���̎�����
�`�ԉ�́i�œK���j�����������邱�Ƃ��l���܂��B
���ʑ̂̐ߓ_��Z���W�i�����j�����𑀍삵�ĒT������c�[��������Ă݂܂����B
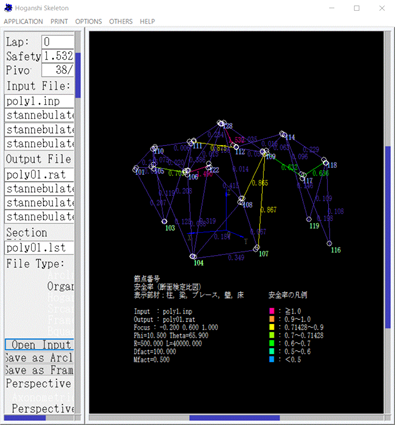 ��
�� 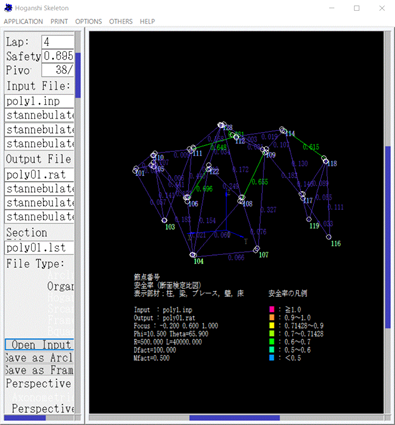
�������z�@�ɂ�鎩�����̃T���v�����f��
�ߓ_��Z���W�݂̂��p�����[�^�[�ɂ��Ď����œK�������
�u�������z�@�v�ɂ��4��̎����v�Z�ň��S���� 1.532 �� 0.695 �ɉ������܂��B
�����̕�����Ȃ����̌��z�����߂邽���u���x��́v�ɂ͎��Ԃ�������܂��B
��������ߓ_��1�Ɍ��肷���1�����̒T���ɂȂ�܂��B
���S��f�̃O���t�����։��ւ��ǂ�T���ɂȂ�܂��B
��������ړ_��2�ɂ����2�����̋Ȗʂ������Ă䂭�T���ɂȂ�܂��B


Gradient Method
Single dimension / 2 dimensions
���z�@�̒T���C���[�W
1���� �^ 2����
�ł��}�ȕ����։����Ă䂭�̂��u�ŋ}�~���@�v�ł��B
�Ȗʂ�ȉ~�̂̈ꕔ�ƌ��Ȃ��Ƒ��������������u�������z�@�v�Ƃ����܂��B
�u��`�I�A���S���Y��(GA)�v�u�i���I�A���S���Y���^�����I�v�@(Evolutionary Algorithm / Generative Design)�v�uESO�@�v�uForce Density�@�v�Ȃǂ̎�@����Ă���Ă��܂��B
�u�������z�@�v�̌v�Z���@�𗝉�����͍̂����܂�܂����v���O�����͂��������ꂾ���ł��B�� �\�[�X�R�[�h
�� Similarity between Form Optimization and Deep Learning
�`�ԉ�͂Ƌ@�B�w�K
�u�`�ԉ�́v���u�@�B�w�K�v�͎��Ă��܂��B
�u�œK���v���uAI�v���T���Ƃ����Ӗ��ł͂���܂���B
����ȕ��������������Ƃ��܂��B
��x +��y = z
�u�`�ԉ�́v�͍œK�� x, y ��T�����ł��B
�uDeep Learning�v�͍œK�� ��, �� ��T�����ł��B
�Ȃ̂Ŏ����A���S���Y���œ�����������ꂻ���ł��B
�u�`�ԉ�́v�́u���͒l�v�̍œK���ł��B
��x +��y = z
���̌W�� ��, �� �͗^�����Ă��܂��B
���̂Ƃ��ڕW�� z �ɋ߂��Ȃ� x, y ��T���܂��B
��F4x + 2y = 1
z���u1�ɋ߂��Ȃ�v�u1�������v�Ȃǂ̖ڕW��ݒ肵�܂��B
�����ł� z=1 �ɋ߂��Ȃ� x, y ��T���Ƃ��܂��B
x=0.10, y=0.10 �������Ă݂�� 4�~0.10 + 2�~0.10 = 0.6
x=0.15, y=0.05 �������Ă݂�� 4�~0.15 + 2�~0.05 = 0.7
�ƂȂ��ď����ǂ��Ȃ�܂����B
������J��Ԃ��Ă��ǂ����ɋ߂Â��܂��B
x, y ���u���W�v�ƍl����Ό`����œK���������ƂɂȂ�܂��B
�uDeep Learning�v�́u���v�̍œK���ł��B
��x +��y = z
���̌W�� ��, �� ��������܂���B
�ł������� x, y �̒l�ɑ��铚�� z �����Ⴉ�������Ă��܂��B
�u���t�f�[�^�v�ƌĂ�܂��B
�����Ȃ�悤�ȌW�� ��, �� ��T���A�܂�K�������������܂��B
��Fx=1, y=4 ��^����� z=2
�@�@x=2, y=2 ��^����� z=1
�ƂȂ�ƕ������Ă���Ƃ��܂��B
�����Ȃ�悤�� ��, �� ���������Ƃ������Ƃł��B
��=0.8, ��=0.1 �Ƃ��Ă݂��
0.8�~1 + 0.1�~4 = 1.2
0.8�~2 + 0.1�~2 = 1.8
��=0.1, ��=0.5 �Ƃ��Ă݂��
0.1�~1 + 0.5�~4 = 2.1
0.1�~2 + 0.5�~2 = 1.2
�ƂȂ��Ă����ԗǂ��Ȃ�܂����B
�u�w�K�v�����Ƃ������Ƃł��B
����Ɏ��ۂ����Ă͂߂܂��B
x=1 �́u��ށv�Ax=2 �́u�M���ށv�Ƃ��Ă݂܂��B
y �́u�r�̐��v�Ƃ��Ă݂܂��B
x=1, y=4 �́u��ށv�Łu�r��4�{�v�Ȃ̂� z=2 �́u���j�v��\���Ƃ��܂��B
x=2, y=2 �́u�M���ށv�Łu�r��2�{�v�Ȃ̂� z=1 �́u�S�����v��\���Ƃ��܂��B
�����ʂ��鎮�� 0.1x + 0.5y ���ƌ�����܂����B
����ł� x=2, y=3�i�M���ނŋr��3�{�j�Ƃ��������ʂ��Ă݂܂��B
0.1�~2 + 0.5�~3 = 1.7
z=1.7 �ƂȂ��� z=2 �ɋ߂��̂Łu���j�v�ɋ߂��Ɣ��ʂ���܂����B
�u���j�x�v��1.7�ƕ\�����Ă������ł����ǂ��炩�Ɩ�������AI�́u���j�v���Ɠ�����Ƃ������Ƃł��B
Deep Learning �̊�{�v���O�����͂��������ꂾ���ł��B�� �\�[�X�R�[�h
�F�ʂ�����v���O����������Ă݂܂����B
�w�K�f�[�^�i���t�f�[�^�j
�ECyan �� {0, 255, 255} �� 1 �܂� f(x,y,z) = f(0,255,255) ={1 0 0 0}
�EYellow �� {255, 255, 0} �� 2 �܂� f(x,y,z) = f(255,255,0) ={0 1 0 0}
�EMagenta �� {255, 0, 255} �� 3 �܂� f(x,y,z) = f(255,0,255) ={0 0 1 0}
�EOrange �� {255, 100, 0} �� 4 �܂� f(x,y,z) = f(255,100,0) ={0 0 0 1}
�ƂȂ��f�������Ă����܂��B
���̏�ŁA
�E�� {180, 140, 40} ���ǂ�ɋ߂������肵�Ă݂܂����B
Yellow �Ƃ̈�v�x62%�COrange�Ƃ̈�v�x27%�ƎZ�o����܂����B
Beginning of computer programming �v���O���~���O�̑�P��

Windows �v���O���~���O�̑�P��
�u�E�B���h�E���J���}�E�X���������v
���ꂪ�ł�����Ƃ̓v���O�����B���Ă䂭�̂͊ȒP�ł��B
�u�`�ԉ�́v�uDeep Learning�v�u�X�y�N�g����́v�Ȃǂ��������{�v���O�����͒Z�����̂ł��B
���C�u�����Ȃǎg�킸���삵�Ă݂�Ƃǂ�ǂB���Ďg���܂��B
�� Local Optimization �Ǐ��œK��
Global Optimization �S�̑��� : Optimization of global shape.
Local Optimization �Ǐ����� : Optimization of local shape using drapes, wrinkle, dimples, or grooves.
���ɉ��ʂ��uDimple�������ځv��݂���Ƌ��x�𑝂����Ƃ��ł��܂��B
�u�f�B���v���v�u�t����̍a�v�u�J�[�e����̃q�_�v�u���̃V���v�Ȃǂ��Ǐ��œK���Ɏg���܂��B
Dimple / Groove / Wrinkle to increase buckling strength of thin plate
Water Pavilion, World Expo in Aichi, 2005, Architect: Naomi Sakuragi + Students, University of Tokyo
Pulp Mold Screen, Tokyo University of Science, 20
COCOON, Seminar in Stanford University, 2016


COCOON, Seminar in Stanford University, 2016
A catenary arch or a dome is globally optimized due to gravity.
In addition, wrinkles and dimples can be attached as local optimization.
���炩�ȃ��H�[���g��h�[�����S�̑����ɂ��œK�����ꂽ�`��ƕ߂炦���܂��B
������u�����ځv��u���˂�v��^�����Ǐ������ɂ���Ă���ɐ��\���グ���܂��B
�Ǐ�������u�t���N�^���v�̂悤�Ɋ�d�ɂ��J��Ԃ����Ƃ��ł��܂��B
�t���N�^���Ɠ��l�ɍœK���ɂ���Ă��i�`�������Ȗ͗l�����ݏo���ꂻ���ł��B


Floral dimples / Crescent dimples
�ԕ��͗l�ƌ��^�͗l�̃G���{�X���H���{��������
��w�@�����u�ԕ��͗l�v�����ʂ��������Ƃ������܂����B
�~����s�^�C������s�^��͗l�ŃR���g���[�����邱�Ƃ��ł��܂��B
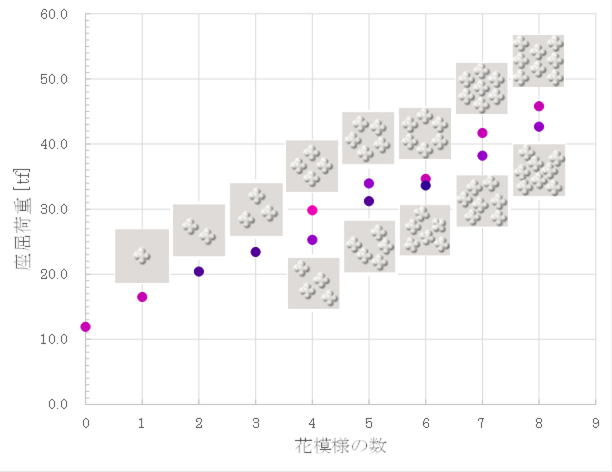

Buckling strength is proportional to the number of dimples
�ԕ��f�B���v���̖��x�𑝂��Ɣ��I�ɍ������x�������܂��B
�u�Ȃ����� = �ʊO�Ȃ��v���d���u������ = �ʓ��L�k�v���_�炩���ł��邱�Ƃ��w�@���������܂����B
����͑��ʑ̂̓W�J�\���ɑ������������ł��B
Scattering floral dimples onto stressful area.
���͂̑傫�ȕ����ɉԕ����U��߂܂��B

Sakura dimples pressed on a copper sheet.
Double-slumped glass sheet with floral dimples.
�^���������� / �K���X�h�[���ɎU��߂�ꂽ�ԕ��͗l
�u�_�u���X�����s���O�v�ɂ��f�B���v�����U��߂��K���X�h�[�������̂ɐ������܂����B
��1�X�����s���O�ʼnԕ������H���A��2�X�����s���O�Ńh�[���^�ɂ��܂��B
���͂̑傫�ȕ����ɉԕ����U��߂��u�Ǐ��œK���v�ɂ���ăh�[���`��Ɏ��R�x�������܂��B
�����炫�ւ�悤�ȃi�`���������������R�Ȗʂ̃h�[�����f�U�C���ł���悤�ɂȂ肻���ł��B
���ꂪ�u���ʊ�n�v�̑��ʑ̂ɂ��g����Ƒ�w�@�����C�Â��܂����B
�� Sakura Dimples: Local Optimization Applicable to Lunar / Martian Base Camp
���ʊ�n�^�ΐ���n
Detailed page �� Quick Setup Lunar Base Camp on the Pole / in the Pit
�T���F2021�N�x
JAXA�F���T���n�u���������u�O��Ə��������W�J�����x�[�X�L�����v(�\����)�Ƃ��̓����Ή���Ԃ̍\�z�v
������w + �v�����c���{���g���[������� + ������Ж + �F���q���J���@�\(JAXA)
�U���F2022�`2025�N�x
���y��ʏȁ{�����Ȋw�ȁ{���t�{�u�F�����݊v�V�v���W�F�N�g�v
�u���̋Ɉ您��яc�E�ł̑؍݊J�n�p�x�[�X�L�����v�̍ŏ��`�ԂƓW�J�����@�\�v
������w + ��B��w + �|���H���X + �F���q���J���@�\(JAXA)
STARDUST PROGRAM�̂ЂƂƂ��Č��^�ΐ���n�̊J�����n�܂�܂����B
�܂������Ȏ��{�v���W�F�N�g�ł͂Ȃ����̂̍\����̂̊J���ɒ��肵�Ă��܂��B
����܂Ō������Ă����\���̃A�C�f�A�̑�������������܂��B
�E�O��ƍ������g���u�����W�J�v
�E���������u�ԕ��f�B���v���v���u�Ȑ��܂�v�ɂ�鑽�ʑ̂̓W�J�\��
�E�u�R�n�v�ւ̒����@�\
�E�P�[�u�����t�g��݂��u�����\���v
�E�u�n�T�~���V�v�̌�����͂����\�[���[�p�l��

Lunar Pole Setup �� Movie
The minimal composition of a base camp on the moon or Mars.
Permanent sunny region on the lunar pole is suitable for photovoltaics.
�u�Ɉ恁��Ɂv�ւ̃Z�b�g�A�b�v
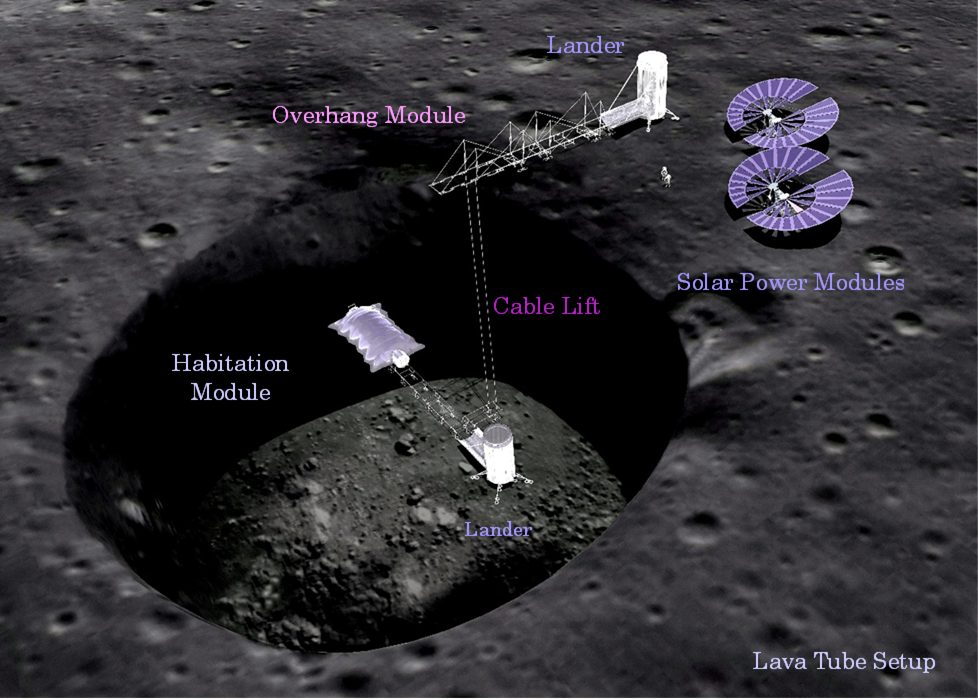
Lava Tube Setup
Lava tube is protected from temperature gaps, radiations, and meteorites.
Habitat Module 3D printed, Scale=1:100 / Aluminum mock up, Scale=1:10
���^�ΐ���n �؍݃��W���[��
�A���@�ɉ^�ꂽ�R���e�i����̃����[�X �^ ��C���œW�J���Ȃ���R�n�ɉ��낷�X���C�h���[���Ƌr��
�Ƃ������@�\�������J�����Ă��܂��B


�؍݃��W���[���̊i�[��� �^ �؍݃��W���[���̃����[�X �� Movie
Deployment test of the body of Habitat Module
Embedded floor deploys simultaneously when the pillow-shaped envelope deploys by the air.
�X�P�[�� 1:5 �̃��b�N�A�b�v�̓W�J���������{�ł��܂����B
�u�؍݃��W���[���v�͓��C���ɂ���u�O��{���������{�r���v���u�����ɑ����Ɂv�W�J���܂��B
�A���~���̊O����u�ԕ��f�B���v���v���U����u�Ȑ��܂�v�Ő܂��݂܂��B

The Sakura dimples enable the multifaceted thin plate to snap-through readily.
�l�p���^�̃��b�N�A�b�v�ɂ���шڂ莎��
�u�ԕ��f�B���v���v�ɂ���āu�ʊO�������Ȃ������v�͑傫���A�u�ʓ��������������v�͏������Ȃ�܂��B
����͓W�J�\���ɕK�v���u��шڂ茻�ہv���N�����₷���ł��邱�Ƃɑ�w�@�����C�t���܂����B
Double fold using curved crease
Curved crease prevents the fatigue failure.
�u�Ȑ��܂�v�ɂ��2��Ȃ�
��������܂��ނƂ�������J�������ɂ��u�Ȑ��܂�v�ɂ���Ċɂ��ȗ��Ő܂�悢�Ɣ���܂����B
1���Ȃ��̒��a�����10�{�A2���Ȃ���100�{�Ƃ���ƐL�ѐ��\10%�ȏ�̃A���~�j�E�������ł͔j�f���܂���B
�����œ����W�J���鍂�� �^�@�R�n�ւ̐ڒn�r

�u��шڂ�v���ɂ����������m�F�ł��镔�����ʑ̂���肵���僂�b�N�A�b�v�삵�܂����B


���^�O��̓W�J���� �� Movie �^ �Ȑ��܂�ɒǏ]����q���W
�������僂�b�N�A�b�v����C���Ŗc��܂��邱�Ƃɐ������܂����B
�܂��ԕ��f�B���v�����{���Ă��Ȃ����̂̊T�˂Ђ��Ⴐ���W�J���܂����B
�u�Ȑ��܂�v�ɒǏ]�ł��钚�Ԃ��J�����܂����B
Dense vegetation in the cramped spaces
Provide oxygen (O2) and food, absorb carbon dioxide (CO2).
��襕����u�����x�Ή��v���Ď_�f�CCO2�C�H���̏z��}��܂��B
The overhang module comprises a tensioning structure to cantilever from the cliff.
The cable lift is suspended from the 15m cantilever.
�I�[�o�[�n���O���W���[���̃��b�N�A�b�v Scale=1:10
�N���[�^�[��c�E�։����ɂ�15m���x�I�[�o�[�n���O������悳�����ł��B


�I�[�o�[�n���O���W���[���̃��j�b�g�������Ő��삵�Ă݂܂����B
The deployable fan panel, referring to the hind wings of earwigs,
was developed by Saito K. et al., Kyushu University.
�\�[���[�p�l���͋�B��w�̐ē���Ɛ搶�ɂ��u�n�T�~���V�v�̌�����͂����W�J�@�\���J������Ă��܂��B
�n�T�~���V�̌����͍����ōő��15�{�Ƃ����W�J�����ւ邻���ł��B


�������僂�b�N�A�b�v�S�̑�
�� Energy Distribution as an Objective Function
�z���G�l���M�[���z��ړI���Ƃ���œK��
�u���S���v�̑����u�z���G�l���M�[�v�̕��z��ړI���ɂ��邱�Ƃ��ł��܂��B

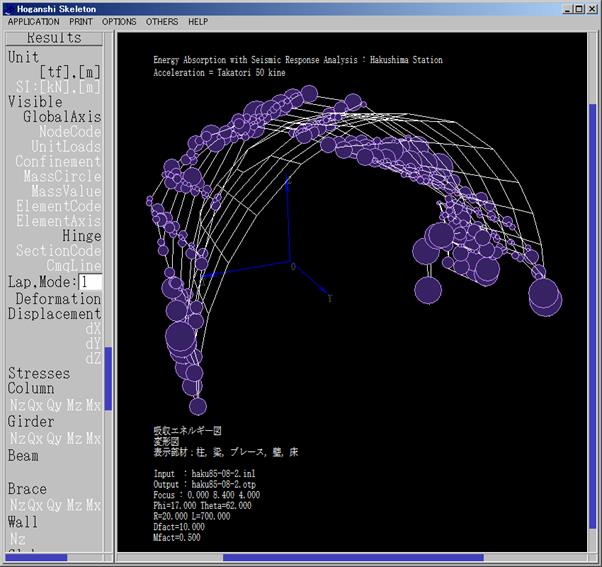
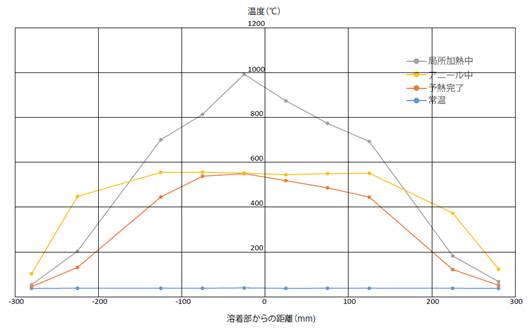
Energy absorption diagram for New Hakushima Station, 2015, Architect : Kazuhiro Kojima / CAt
�����V�w (���z�ƁF������_�{�ԏ�����q)
�n�k���ɋz�������G�l���M�[�����������u�G�l���M�[�c�q�}�v
�u������́v�ɂ��n�k���̐U�����V�~�����[�V�������e�ނ��z�������G�l���M�[���u�c�q�v�ŕ`���܂��B
���̃G�l���M�[�c�q�̕��z���œK�����鎎�݂ł��B


���炩�ȃA�[�`�́u�����v�ƍ��E�́u�����v�ɑ������������܂��B
4��������������͕̂s����x�̍�������`�ł��B
�u���˂�v���{���Ƒ��������u�����v�Ɋ���3�q���W��Ԃň���x�̍�������`�ƂȂ�܂��B
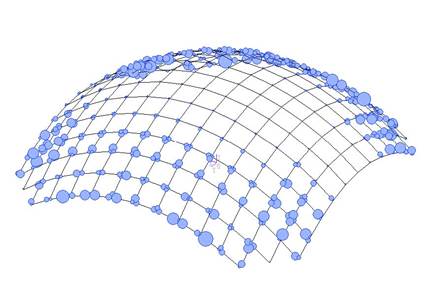
���炩�ȃh�[���͑S�ʓI�ɑ������ĕs�����x�̍�������`�ƂȂ�܂��B
�������𑫌��ƒ����Ɋ���ƈ���x�̍�������`�ɂł��܂��B
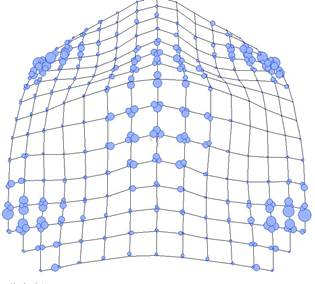
�ԕ����U��߂��i���L����������܂���B
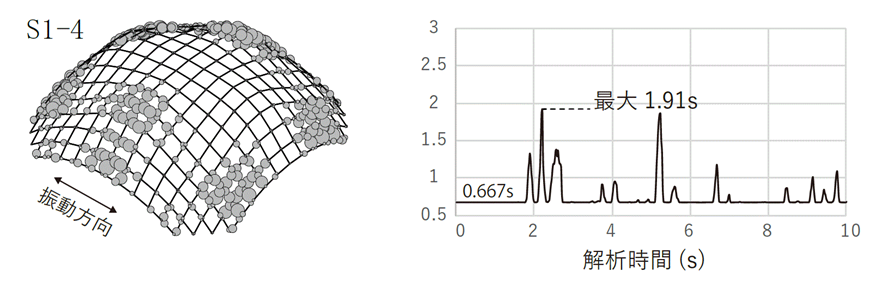
�U���̉ߒ��Ŋώ@�����ŗL�����̕ϓ�
�����̋���u�ŗL�����v���ώ@���邱�ƂŌ��o���邱�Ƃ��w�@������Ă��܂����B
�ŗL�����������Ȃ�`��̓G�l���M�[�c�q�̕��z���ǂ��Ȃ����ƂɂȂ�܂��B
������͂�4000��قǂ̌v�Z�Ŗ��x�ŗL�������v�Z����͖̂c��Ȍv�Z�ʂł���
����Ȃ��Ƃ��ł��鎞��ɂȂ�܂����B
�� Contraction Method in Buckling Eigenvalue Equation
�����ŗL�l���̏k��
When we could know buckling strength of each element, we can manipulate buckling phenomenon more precisely and compose the structure with slim elements.
Contraction method seems applicable to extract buckling strength of each element.
���̍��g�S�̂̍����ŗL�l�����u�k��v�����u�ނ̍������x�v�����o���邱�Ƃ����݂܂��B
������u�ړI���v�ɂ��Č`����œK�����邱�Ƃ��ł��܂��B
�����̍ނ��琬��\���̂̏I�Nj��x��͂��s���ہA�u�Y���i�I�ǁj�߁v�̐ݒ�̂��߂ɂ��u�ނ̍������x�v���K�v�ƂȂ�܂��B
Buckling analysis for 3D frame
Buckling strength distribution of dimpled wall calculated by contraction method
Published in JIA paper, 2017
�������[�h���S�̓I�ȕό`�ƂȂ闧�̍��g�^�u�f�B���v���i�����ځj�v��݂����ǂ̍������x���z
�L���v�f�@�iFEM�j�ɂ����Đߓ_��N����S�̍��g�̍����ŗL�l���́A���̂悤�ɕ\�����B
[KE] {Ui} = ��i [KG] {Ui} �� 6N�s�̕�����
�����ŁA[KE]�F�e�������}�g���N�X
[KG]�F�w�I�����}�g���N�X
��i�F�����ŗL�l
{Ui}�F�����ŗL�x�N�g���i�������[�h�j
�K�E�X�̏����@�Ɠ��l�̎菇�� k �Ԗڂ̍s�Ɨ����������k������̂悤�ɕ\������B
�e�����}�g���N�X�̗����ɓ����W���s�� [C]�C[D]���|���邱�Ƃ��l����B
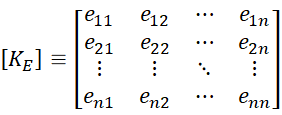
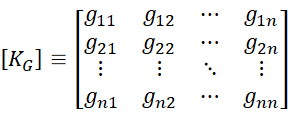
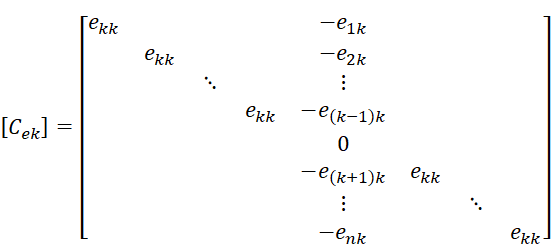
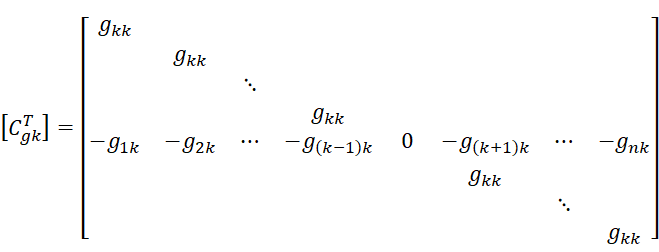

�����p���Ċe�����}�g���N�X [KE]�A[KG] �Ɏ{���Ǝ��̂悤�ɂȂ�B
![]()
![]()
����ƌŗL�l���́A
![]()
[KE'] {Ui'} = ��i' [KG'] {Ui'} �� 6N - 1 �s�̕�����
�ƂȂ�B��������X�J��Ԃ��āA�ނɊ֘A���镔���������c���B
�ŏI�I�Ɏc�鍄���}�g���N�X��[KE'']�A�����}�g���N�X��[KE''] �Ə����ƁA
[KE''] {Ui''} = ��i'' [KG''] {Ui''} �� ���ނł�12�s�̕�����
�Ƃ��Čނ̌ŗL�l�����߂邱�Ƃ��\�ł��邱�Ƃ���������܂����B
���̌ŗL�l���ނ̍������x��\�����Ƃ����҂���܂��B
�P���Ȗ�^���g�̎����ł͑Ó�����������܂����B

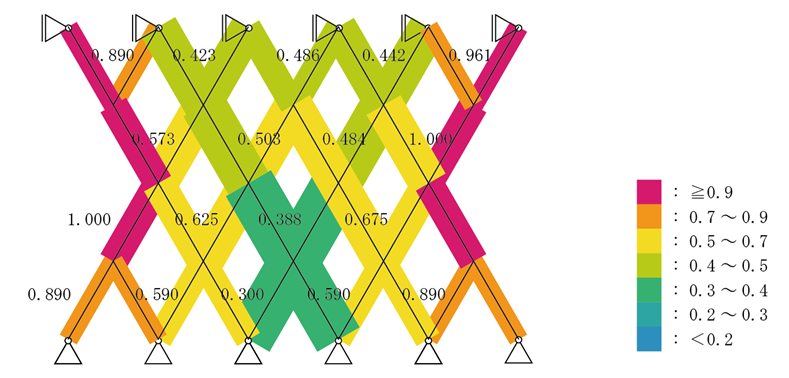
�ł��d�𑽂����������̂̎����C�d�ό`�Ȑ�
�{��@�̑Ó����̌���
��������2�̒��̋��x�̓O���[�͈̔͂ɂ���ƕ�����B
����ɑ��A�{��@�Ōv�Z����鋭�x�͐ۂȂ̂ŁA�����������l���悭�\���Ă���B
�`��œK���ւ̉��p��
�ߊi�q�ǂ̗v�f�̑������œK������
�� Updating Optimization �����œK��
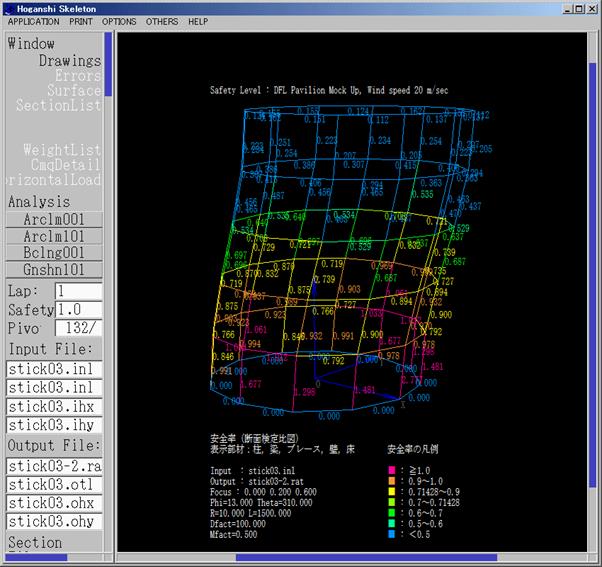
PAFF, Digital Fabrication Laboratory (DFL) Pavilion, 2017
�v��F������w�����S�����
����A�W�A�ł̓R�R�i�b�c�̑@�ۂ���ʂɗ]���Ă��܂��B
�����S������ł�����ۂ߂Ēe�ɂ��ĉ����ɕ~���l�߂悤�Ƃ����A�C�f�A�����܂�܂����B

���ꂽ�l�b�g�ɏd�ʂ��ڂ���ƁA���ׂ����������ɋ����Ɖ��߂ł��邱�ƂɋC�t���܂����B

�������čl����ƁA�l�b�g�������ł���Ƃ��́A�d�����������ق�������Ȃ悤�Ɍ����܂��B

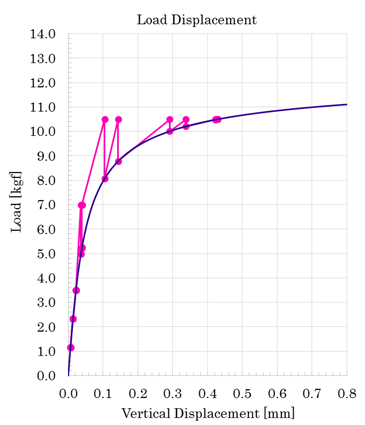
�v���g�^�C�v�ɂ���̓��f���^����`��͂ɂ������v�Z�̗l�q
���܂ł��s����ɋ߂��\���Ȃ̂ŁA���ɂ͔���`��͂��K�v�ł��B
�������̌Îs���ɂ��ANewton Raphson �@�̈��ł��� Co-rotation �@�����ǂ���
��̓A���S���Y���̃v���O�������ғ����A�������Ƃ��ł��܂����B



�R�R�i�b�c�̑@�ۂ̒e�͉����̌������̌����Ȃ��Ƃ���փG�A�o�Y�[�J�Ō������܂�܂��B
�������ފp�x�̓w�b�h�z���̉����m�点�Ă���邻���ł��B


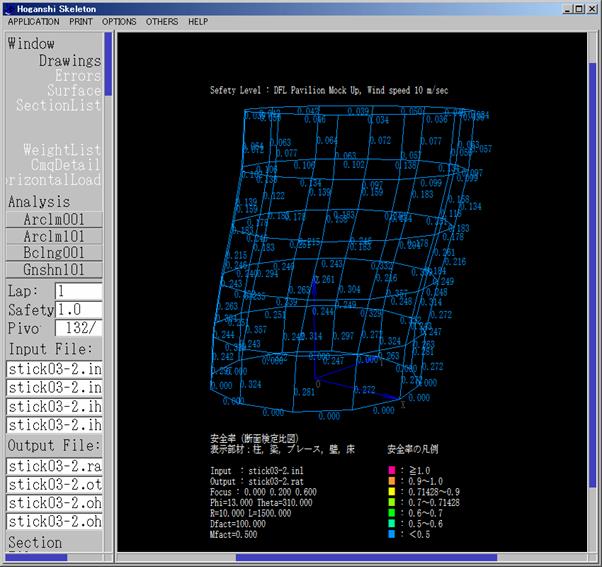
Day 6 (final day) : Target weight / Deformation
6���ځi�ŏI���j�̖ڕW�d / �ό`
�� : ���̓��̏����l�i�O���̌���̕ό`�v���l�j
�� : ���̓��̕ό`�\��
�� : ���̓��̕ό`����
�ǂ��ւǂ̗ʂ̑@�ۂ�ł����ނׂ������肷��A���S���Y�����\�z�ł��܂����B
�ł����܂ꂽ�e���ڕW�n�_�ɒ��e���������v������̂łȂ��A1���̍�Ƃ��I������璌�̕ό`���v�����܂��B
����Ɨ\���Ƃ̈Ⴂ�ŏd���̕��z�̌덷��������܂��B
��������ƂɎ��̓��Ɍ������ޖڕW�ʒu�����܂��B
������u�����œK���v�ł��B
Wetland, Venice Biennale 2021, National Pavilion UAE
Wetland, ���F�l�`�A�r�G���i�[��2021, UAE�p�r���I��
Awarded: Golden Lion
Management: The Salama bint Hamdan Al Nahyan Foundation
Organizer: Ministry of Culture and Knowledge Development
Curator: Kenichi Teramoto, Wael Al Awar
Structural Advisor: Jun Sato
Navigation System Developer: Yusuke Obuchi
��ÁFThe Salama bint Hamdan Al Nahyan Foundation
���� : Ministry of Culture and Knowledge Development
�L�����[�^�[�F���{����, Wael Al Awar
�i�r�Q�[�V�����V�X�e���F������w�����S�����
In UAE, pure water is extracted from seawater, and a huge amount of salt is left.
Magnesium can be extracted from that salt, and it is useful as an ingredient of cement.
Coral-shaped pieces with a dimension of 40cm, made of magnesium cement, are stacked up to 2.7m.


UAE National pavilion in Arsenale
�C�݂ɗ��ꒅ�����T���S�̂悤�ɁA�}�O�l�V�E���Z�����g�����u�T���S�v��2.7m�ɐϑw���܂����B


Leftover salt / Salted surface of the coral unit
�\�ʂɉ������������Ă��܂��B
�A���u���A�MUAE�ł͊C������^�������Ă��܂��B
��ʂɗ]�鉖����}�O�l�V�E�����̎�ł��܂��B
���̃}�O�l�V�E���ŃZ�����g����邱�Ƃ��ł��܂��B

Stacking test uo tp 2.3m / Loading test / Casting test using ground as a mold
To provide around 2500 pieces of the free-shaped brick in 2 months,
Jun Sato recommended using the ground as a mould.
The grooves could be drawn quickly on the ground, and the brick became coral-shaped.
2.3m�ɐϑw�������b�N�A�b�v �^ �ϑw��Ԃ̈��k���� �^ �T���S���W���[���̐���
�u�}�O�l�V�E���Z�����g�v�Ŏ��R�`��̃��W���[��������Đϑw�ł��Ȃ����Ǝ��{���l���Ă��܂����B
2000�`6000���̃��W���[����2�����قǂō��Ȃ���Ȃ�܂���B
�y�ɍa���@��Z�����g�𗬂�����Ōł߂��u�T���S�v���W���[���Ȃ����ƍl���܂����B
Navigation tools
To manipulate the huge number of free-shaped pieces, navigation tools could be developed by Yusuke Obuchi.
A positioning device on the arm tells us the area to put the piece,
and the pointer tells us the location of each piece.
�ϑw�ʒu���w������i�r�Q�[�V�����V�X�e�� �^ �ϑw������Ԃ̈ʒu���̎擾
�����S��搶�̌������Ńi�r�Q�[�V�����V�X�e�����\�z����܂����B
�T���S���ǂ��ɐς߂悢���r�ɂ����������v���_���܂��B
�ʂ����Ăǂ��ɐς܂ꂽ���X�L�������܂��B
�T���S�̌`�ƕ����܂Œm��K�v�͂Ȃ��ƍl���܂��B
�T���S1��1�_�ł悢�̂Ŗ_�̐�̐ԋʂŃT���S�̒������`�����ƐG�邾���ł��B
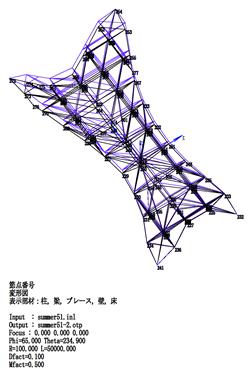
Simplified structural analysis model for updating optimization
Through the loading test, the structural performance could be related to just the density of the wall,
so the shape and orientation of each piece do not need to be detected.
The overall shape was stacked layer by layer, day by day.
When a layer is stacked, a simplified structural analysis model can be updated using the coordinates
announced from the pointer, and the form of the upper unbuilt part can be optimized again.
�œK���c�[��
�T���S�̈ʒu����ς܂ꂽ�u���x�v��������܂��B
�\����̓��f���̓T���S�̖��x��������Ή�����ƍl���܂��B
����Ȃ�f������̓��f�����\�z�ł��܂��B
6�w�ɕ�����1�w�ł��閈�ɍ\����͂��܂��B
�ڕW�̖��x�Ƃ͈Ⴄ�������ł���̂ł��������̌`����œK�����ĕ���܂��B
�傫�ȁu�덷�v����e��������ɂ�����œK����i�Ƃ��āu�����œK���v�̒�ĂɂȂ�܂����B
�� Timber Joinery for Free Aggregated Form
Sunny Hills in Aoyama Tokyo, 2013
Architect: Kengo Kuma
Structure: Jun Sato
Published: MARK #54
���z�ƁF�G����
�\���F�����~�\���v������
Shaggy free-form timber structure provides an inner space filled with Komorebi – sunlight through leaves.
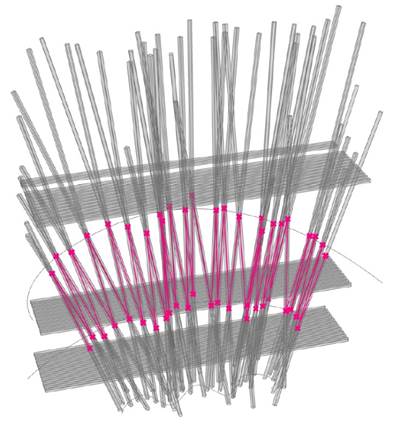
Timber joinery for 4 timbers to cross could be developed, referring to Japanese traditional joinery.
����́A���{�̓`���I���u�ؑg�v�̋Z�p�����p���āA�ׂ��ȍނ����V�����V���ɑg�܂ꂽ�\���ł��B
���ꂪ���������Ƃ��ɂ���ȓ��ߐ�����\���f�U�C�������⎋�����R���g���[�����u������сv�̂悤�ȓ�����Ԃݏo���u�t�B���^�[�v�Ƃ��Ă������������Ɗ����܂����B

Sunny Hills in Aoyama
Timbers are interlocked using unique Kigumi – timber joints without metal fixings.
A mesh structure can serve as a filter of light, sight, air, heat, sound, water and ecosystem.
�u�n���g�݁v�����p���Đ��܂ꂽ�u�ؑg�v�̍\��
Discussion with Kengo Kuma is always just a brief moment of 10 minutes.
It is necessary to develop an innovative imagination from his few words such as �gscattering tiny particles�c�h
�G����Ƃ̑ł����킹�͋ɒ[�ɒZ����10�����炢�B�u�����ȍށv�������_���Ɂu�p���p�����Ɓv�W�ς����C���[�W�A�Ƃ������t�𗊂�ɁA�ؑg������Ă݂悤�Ǝv�����A�C���[�W��c��܂��Ă����܂��B

Chidori / Jigoku Grid developed by traditional carpenters in Hida district.
�u�n���g�݁v�u�璹�i�q�v�ȂǂƌĂ�镽�ʊi�q�B
���Ĕ�˂̑�H�ɂ���čl�Ă���܂����B
�d���ނ����݂ɑg�܂�Ă���s�v�c�Ȋi�q�B


Crossing timbers / Processed timbers / 2D projection of overlapping timbers
We should develop a suitable way of projection onto a 2D display.
�؍ނ̍��ݕ��́u���ߕ\���v�u���@���̗��̕\���v�Ȃ�2�����ł̕\���ɍH�v���K�v�ł��B
���ꂪ�ł����BIM�ɂ����p�ł������ł��B
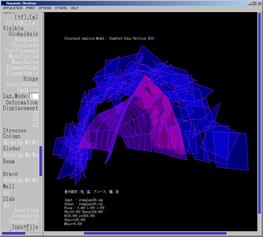
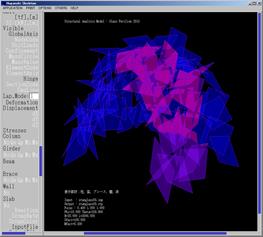
Structural analysis model / Detailed buckling analysis model
��̓��f���S�̐}�^�������f���ɂ��������
6cm�̍ނ�3�w���x������͈̂�ؓ�ł͂����܂���B
�q�m�L�ōd�߂�E110���g�p���܂��B
������͉͂������ׂ͍��ނƂ����ڍׂȃ��f���ʼn����K�v������܂����B

Component Growth Process
The timbers are continuous, but the global form can be understood as stacked voxels geometrically.
�؍ނ͌q�����Ă��܂����S�̌`�̊w�I�ȍ\���͒P�Ȃ��u�{�N�Z���`���v�ł��B
���B�͒P����voxel�̂悤�ɕ��ׂĂ䂭�����ŁA�ڍ��Ƀp�����[�^�[�͂���܂���B

Random Operation
The simple voxel system enables the quick provision of random global shapes.
It is helpful in the Genetic Algorithm of the optimization.
��`�I�A���S���Y���̂悤�Ƀ����_���ɑS�̌`�������@�ɂ��K�p���₷���V�X�e���ł��B

�@�@�@�@�@�@�@�@�@�@�@�@�@�@�@�@�@�@�@�@Target Global Shape
Topological Operation
This system also enables quick feedback from the topological optimization.
The thickness of the structure provides a gradual pattern under a condition of constant porosity.
�t�B�[�h�o�b�N���e�Ղ� Topological Optimization ���K�p���₷���B
���̋ψ�ȍ\�����A�{�����[���̈Ⴂ�ɂ���ăO���f�[�V�����ݏo���܂��B
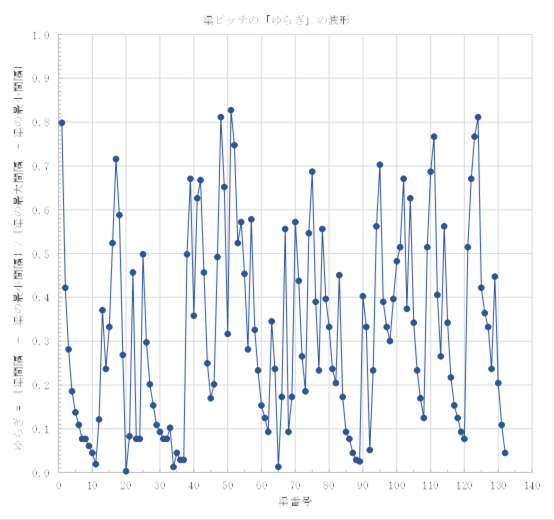
�� 1/f fluctuation / 1D Spectrum Analysis
1/f ��炬�^�P�����X�y�N�g�����
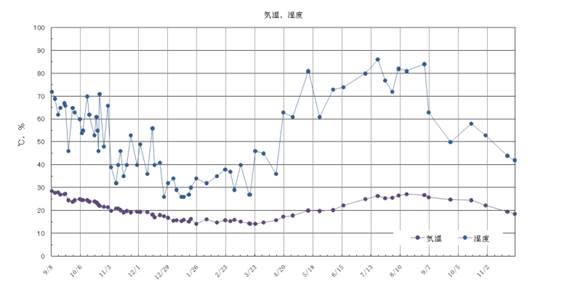
Cafeteria in Chiba University of Commerce, 2015
Architect: Kazumi Kudo + Hiroshi Horiba / Coelacanth K&H
Structure: Jun Sato
Geometry Advisor: Takashi Chiba
��t���ȑ�w�J�t�F�e���A
���z�ƁF�H���a���{�x��O�^�V�[���J���XK&H
�A�h�o�C�U�[�F��t�M�j�^���z�s�{�b�g
The 1/f fluctuation (pink noise) makes music or visual patterns to be comfortable and natural.
For this roof, thin LVL beams are assembled in a 1/f fluctuation pattern of spacings.
�ؖڂ≹�y��S�n�悢�Ɗ�����̂�1/f��炬�̌��ʂ��Ƃ����܂��B
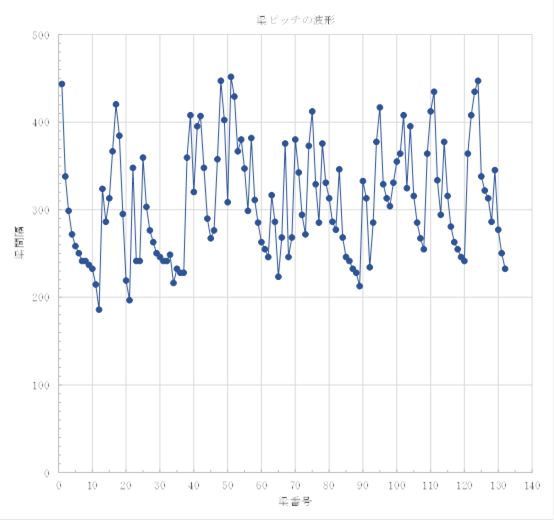
�����炢�k�u�k�����ߊi�q��ɕ��ׁA���̃s�b�`���u1/f ��炬�v�̃��Y�����������邱�Ƃ���t������Ă���܂����B
���R�̕�����������A�g�b�v���C�g����ؘ͖R����̂悤�Ȍ����������݂܂��B

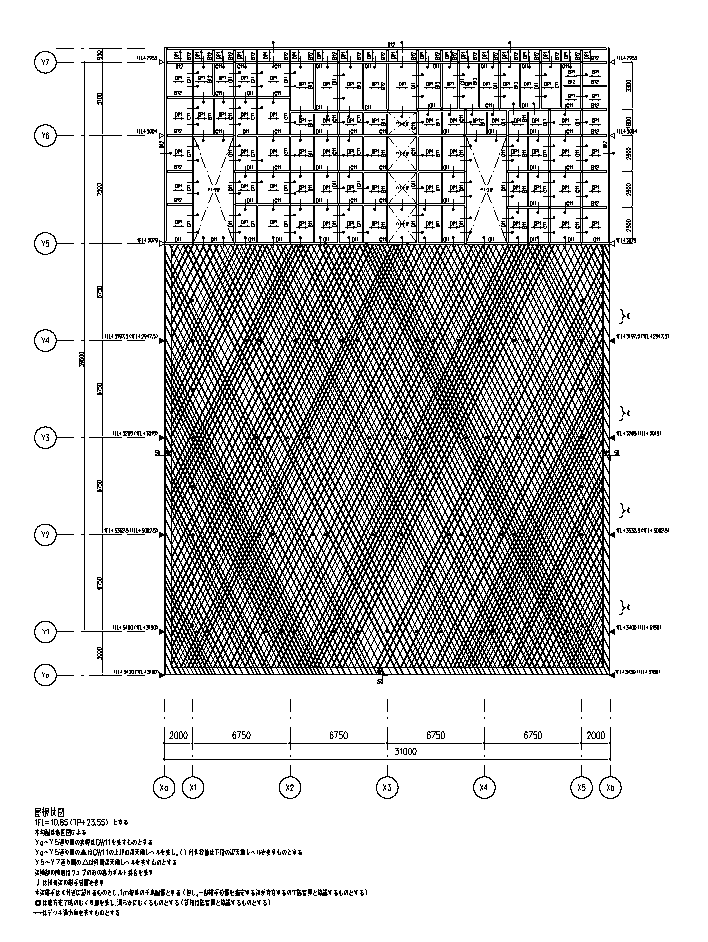
Roof pattern �������}

Section ����̎��g�}
LVL�������x�����钌�� ��141.3x30 �Œ����s���ڍ��B
�S�����[�����\�������̒��� H-125x125x6.5x9 �������ڍ��B
�d���̃o�����X������ĕΐS����}����B
Safety ratio diagram ���S���̐F�\���}
The location of columns was optimized due to the pattern of beams.
���[�o�[���LVL���̃s�b�`����ׂ������g�`�ƌ��Ȃ��� 1/f ��炬�̖͗l��`���B
���̗��z�u�ɑ��Ē��̈ʒu���蓮�`�ԉ�͂ɂ��œK������B
Original wave Fluctuation
Progression of spacings
Assume the number of data = N.
�g�̃f�[�^�� N ����Ƃ���B�iN �͋����Ƃ���̂��悢�j
am = a0, a1, a2,�c aN-1 (m = 0�`N-1)
Assume the interval of data = ��t, total period Td results in as follows.
�f�[�^�擾�̊Ԋu�� ��t �Ƃ���ƁA�p������ Td �́A
Td = N ��t
Assume Ck (k = 0�`N-1) as the factors of Complex Fourier Transform, Ck and the amplitudes Xk are expressed as follows.
���f�t�[���G�W���� Ck (k = 0�`N-1)�Ƃ���ƁA�t�[���G�ϊ��̎��́A

�Ƃ����ƁA

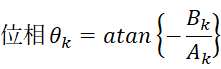
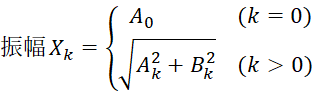
![]()
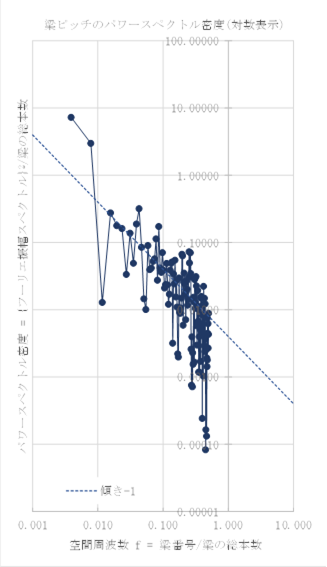
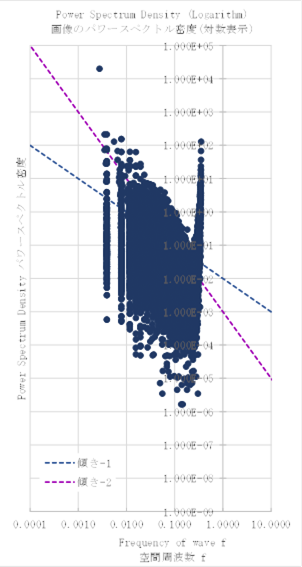
Power Spectrum by Fourier Transform, logarithm scale
Horizontal axis : f = frequensy of wave
�gPower�h can be understood similar as �gamplitude.�g
When the logarithm scale graph with the horizontal axis �g f �h shows a distribution of -1 gradient, it indicates 1/f distribution.
���s�b�`�̐����g�`�ƌ��Ȃ��A���́u��炬�v���������o�����g�`���t�[���G�ϊ����āA�p���[�X�y�N�g�����x�i�U���̂悤�Ȃ��́j�̕��z��`���܂��B���������g�����Ƃ����ΐ��O���t�ŕ`���Ɛ}�̂悤��-1�̌X�������̂�f��-1��܂�1/f�̌`�ɕ��z���Ă��܂��B�����Ƃ���̏��Ȃ�����ɂ��邱�Ƃ��ł��܂��B
Spectrum analysis is expected to be useful for manipulating environmental factors. There might be some other formulae or parameters existent which are related to environment elements.
�u1/f ��炬�v�͑��ɂ����l�Ȍ`�ԂɓK�p�ł������ł��B
�� 2D Power Spectrum in Creating Komorebi Naturalness / Multi-objective Optimization
�u������сv�ݏo���Q�����X�y�N�g����� / ���ړI�œK��
The 1/f fluctuation (Pink Noise) of 1D spectrum makes musics or visual patterns to be comfortable and natural.
Spectrum analysis is applicable to 2D phenomena.
1�����̔g���u1/f ��炬�v�̓��������ƁA�u�i�`�������v�u���K�v�Ɋ�����ƌ����܂��B
�����2�����Ɋg�����ĉ摜�ɓK�p���邱�Ƃ����݂܂��B



Komorebi : Sunlight comes through leaves in the woods.
Sazanami : Ocean ripple, containing acoustics.
Seseragi : River stream, containing acoustics, cool breeze, humidity, smell of moss, and Komorebi.
���{�ɂ͑f�G�Ȍ��t������܂��B
�u������сv�u�����Ȃ݁v�u�����炬�v
�u�����炬�v�́A�������łȂ����A���x�A�C���A���C���D��Ȃ���Ԃł��B
�摜�����̎�@���u�Q�����X�y�N�g����́v������܂��B�摜�̃s�N�Z���̐F�̒l�́AX, Y�����ɕ��Ԑ���ƌ��邱�Ƃ��ł��܂����A�����g�`�ƌ��Ȃ��A���́u��炬�����v���t�[���G�ϊ����A�u�p���[�X�y�N�g���v��`���܂��B
������g���āA�u�i�`�������v�u���K�v�Ƃ��������]�������ۂƂ��ĕ߂炦�邱�Ƃ����݂܂��B
 ��
�� 
Original image / 2D power spectrum image converted from grayscale image
White = high power, Dark gray = low power, Navy = 0.0
�u�ؘR����v�̉摜�́u��炬�v�̂Q�����p���[�X�y�N�g��


Sample image : 64x64 pixels
2D spectrum is a top view of the 3D diagram
�Q�����̃t�[���G�ϊ��̎��́A�P�����ƂقƂ�Ǔ��l�ł��B
�g�̃f�[�^�� M�~N ����Ƃ���B�iM, N �͋����Ƃ���̂��悢�j
amn = a00, a01, a02,�c a(M-1)(N-1) (m = 0�`M-1, n = 0�`N-1)
���f�t�[���G�W���� Ckl (k = 0�`M-1, l = 0�`N-1)�Ƃ���ƁA
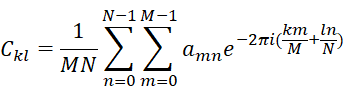

�Ƃ����ƁA
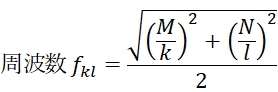

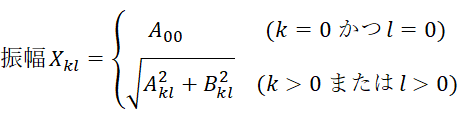
![]()
Original image / Monochrome / 2D power spectrum / 1D power spectrum



Komorebi (sunlight through leaves), Full wave
�ؘR����C�摜���̂܂܂̃p���[�X�y�N�g��
�@�@�@�@�@�@�@�@�@�@�@�@�@ 

Komorebi (sunlight through leaves), Fluctuation wave
�ؘR����C�u���ϒl�v����́u��炬�v�̃p���[�X�y�N�g��




Komorebi (Sunlight through leaves) / Japanese Pampas Grass / Weird Ground
Weird Ground is showing similarity with White Noise.
You can list up 2D spectra of some images, and the list works as a catalogue of your perceptual expression for the patterns which you composed.
���̑��A���R�̕��i�̃X�y�N�g���͂���ȕ��ł��B
�����Ń��X�g�A�b�v�����ʐ^�̃X�y�N�g����p�ӂ��邱�Ƃ́A�����̊��o�̕\���@���u�J�^���O�v�Ƃ��Ď����Ƃɑ������܂��B










2D Spectrum Catalogue
Ocean Ripple / Komorebi / Fleecy Cloud / Autumn Woods / Pampas Grass
2�����p���[�X�y�N�g���̃J�^���O
�����Ȃ� �^ ������� �^ �킽�_ �^ �g�t�̐X �^ �������쌴
������\�z���Ɣ�r���Ă݂܂��B
�\�z�������ݏo���i�F�͂���A�܂��͖ڕW�̃X�y�N�g������������悤�ɍ\�z���̖͗l���œK������A�Ƃ��������p���l�����܂��B



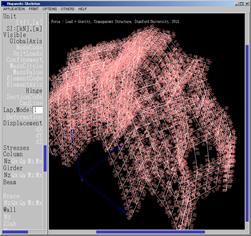
Transparent Structure as Perceptual Filter, Workshop at Stanford University



Sunny Hills in Aoyama



Veiled Tectonics 2018 : Thistle Leaves, Structural Design Studio, The University of Tokyo
Overlapping glass structure shows a spectrum quite similar to Komorebi scene.
Shaggy Kigumi structure shows a spectrum similar to Autumn Woods scene.
Veiled Tectonics shows a spectrum that has some elements of Autumn Woods and Pampas Grass.
�X�^���t�H�[�h��w���[�N�V���b�v�ł̃K���X�\���́A�u�ؘR����v�Ƃقړ����B
Sunny Hills �̖ؑg�́u�g�t�̐X�v�ɋ߂��B
���E�Ŕ��̕z�u�V���̉H�߁v�̖��e���Z�O���e�B�́u�g�t�̐X�v�u�������̌��v�̗v�f�����B
����́u�\���v�f�v�Ɓu���v�f�v�̗������`��œK�����u�]���w�W�i�ړI���j�v�ɂ��Ă����u���ړI�œK���v�����Ă��邱�ƂɂȂ�܂��B
�^�Ă̊C�݂œǏ�����̂��S�n�悢�A����Ȋ����ʓI�ɔF���ł��邩������܂���B









Creating Komorebi using only circles
�w�͗l�̑g�ݍ����ŖڕW�̃i�`�������x�ݏo�����Ƃ��ł������ł��B
�� Geometry to Provide Natural Forms / Flexible Angle Kigumi Joinery
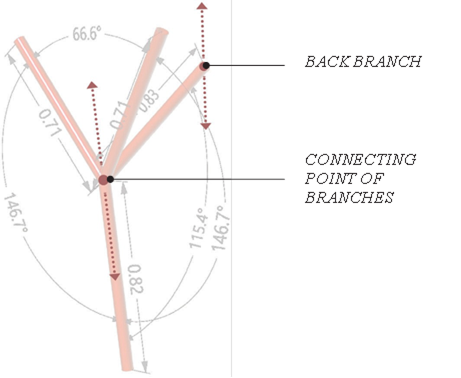
Pergola at the bottom of Carved Tower, Vancouver, 2017
Carved Tower �̃p�[�S��
���z�ƁF�G���ጚ�z�s�s�v������
�\���@�F�����~�\���v������
Published: Lixil eye 13, 2017
Kigumi pergola of 15m tall, 40m long, located at the bottom of a skyscraper.
�����w�r���̑����Ōv�悳��Ă���A����15m�A�S��40m�̃p�[�S���̌v��ł��B


Partial model
Taking this flexible angle joinery into account, the shaggy shape could be provided using parametric algorithm.
Fanning 5-column modules are located on waved lines.
Column modules are connected with fanning elements.
Number and location of crossing points can be structurally optimized by the parameters of this geometry.
�ؑg�V���[�Y��T�e�F�o���N�[�o�[�̃p�[�S���̕����͌^
�g�`�ɕ������ނŐ��ɖa���A�u�ˉe�ʑ��v�ɂ���čނ̖��x�𑀍삵�܂��B
�ڍ��p�x���p�����[�^�[�ƂȂ��Ă���w����Ō`�Ԃ��œK�����邱�ƂɂȂ�܂��B

Flexible Angle Kigumi Joinery
To interlock the timbers in a free 3D-angle, this carved shape could be detected.
It can be carved by CNC roughly, and carpenters will finish them.
���̂��߂Ɂu�����p�v�Ɓu�Ђ˂�p�v�����R�ȑ����̍��ݕ����l�Ă��܂����B
CNC�ł��̂悤�ȓ��������߂�悤�ɂȂ�Ɩؑg�݂̎��R�x���i�i�ɑ������Ƃ����҂���܂��B
Susuki / Pampas Grass: Shelf using Flexible Angle Joinery
���R�����ؑg�̒I�u�������v, 2021
�v�FMariya Stoycheva + Ruta Stankeviciute�^������w�����~������
�؍މ��H�F�������돺�^�͂��H�[
�g��w���B�F�������b�{���䂳���^littlemedia
�ޗnj��_�ѕ��ޗǂ̖u�����h��
�g��w���ށF�g�c���ފ������
Carved Tower �����̂�҂�����Ȃ��̂��u���R�����ؑg�v���g���ĉƋ������Ă݂܂����B

���R�����ؑg�̒I�u�������v �� ���L�����p�X�����ɂď�ݓW����
�c��48�{�A����42�{�A�c�ނ��u�������v�̂悤�ɉ��ނ�a���܂��B
�u�g��w�i�Ђ̂��j�v��2cm�p�ɐ��ނ��Ă��炦�܂����B
�ؖڂ̐^�������ʂ����ނȂ̂ŁA�ׂ����ނ��Ă��߂Ɋ����܂���B


�v�r����3D���f�� �^ �ŏ��̖͌^


Flexible angle joinery to cross in narrow angle
�p�x�̎��R������3D�v�����g �^ ���܂ꂽ2cm�̊p��
�p�x�́u���R�����v�͂���Ȃɉs�p�ȕ������c���Ȃ���Ȃ�܂���B
����͂܂��uCNC���H�@�v�ł͍��߂܂���B

������Ƌ��H�̔������돺����ɂ�����1�l�ō���ł��炦�܂����B
�u�w�i�̂݁j�v�Ɓu�����v�̋Z���Ⴆ�܂��B

�u�`�a�v��h���Ėh���d�グ���܂��B
���������߂�u�g�v��`���A��Ɍ�_�����߂�u���˂�v��2�{�`���܂��B
������R���g���[���|�C���g�Ƃ��ďc�ނ���ׂ܂��B
���ނ����ꂼ��K��2�_�ȏ�Ŏx����ʒu�������܂��B

��������A�ނ̐c���m��5mm�قǗ���Ă���ƒ��悭�f
�ʂ��c���ċ��x���m�ۂł���̂ŁA���������ʒu���u����
�T���v�����āu�œK���v���܂����B
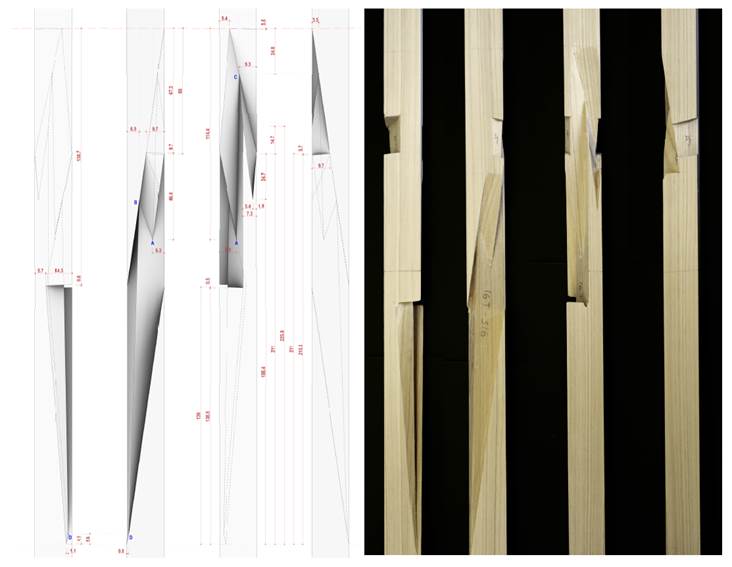
�ނ̈ʒu�����܂�ƌ������̍��`����u�����������v�ł��܂��B
�`���̑�H�Z�p�ƍŐV�̃f�W�^���Z�p���Z�������`�Ԃ����܂�܂����B



A tool to carve this shape is under development in Jun Sato Lab, The University of Tokyo.
���R�����ؑg��5��CNC���H�@�ō��߂�悤�ɂ��錤���J��
���������������g���Ă���̂����Ċw����5��CNC���H�@�ɏ����̓����������Ă�������ތ����J�������܂����B
�܂����t�ō��ނ̂Ɏ��Ԃ�������܂������Ҋ�������x�ɂ͂ł��܂����B
CNC���H�@�łł��邱�Ƃ�������ƁA��H������đւ���̂ł͂Ȃ��A��H�̋Z������Ɏ䂫������Ɗ����܂��B
Digital Fabrication ����g���� Parametric Design �����{����ɂ͂܂�������Ԃ������Ȃ���Ȃ�Ȃ�����ǁA�r�����Ȃ����̂ł͂Ȃ��Ȃ��Ă��܂����B


�p�v�A����Korowai���̃c���[�n�E�X
http://www.natgeocreative.com/ngs/photography/about/index.jsf
�C���h��Meghalaya�̃��[�g�u���b�W
https://amritmahotsav.nic.in/blogdetail.htm?82
�p�v�A����Korowai���͒n��30m�̏��Ƀc���[�n�E�X��݉c���ĕ�炵�Ă��܂��B�C���h��Meghalaya�ɂ͐��������������ċ�����閯�������܂��B��X�ɂ���Ȃ��Ƃ��ł���ł��傤���B���������B������u�ł��Ȃ��Ȃ�v���Ƃ�����悤�ł��B
�ł�������͂��A����`��͂��A�f�W�^�����H�@���A�{��H�̐E�l�Z����g���āA�������߂���u�������v�ȍ\����n�邱�Ƃ��ł���悤�ɂȂ�܂����B�������Ď��R�ɖ�������悤�ɂȂ�ƁA�u������сv�̂悤�ȃi�`�������Ȍ��̈��鎩�R�Ȍ`�Ԃ����ݏo�������ł��B
���Ă����ȂȂ��\��
�ɍׂ̍ނł��{�����[�������̂��`������t���X�P�[���̌��z�����邩������܂���B
���ׂ��ނłł������ߐ�����\�����u�i�`�������v�ȋ�Ԃݏo���Ȃ���A�ЊQ���u���Ă����ȂȂ��v�A����ȍ\�������܂ꂻ���ł��B
Treehouse in Setagaya, 2019
�v��n�F�����s
�c���[�n�E�X�N���G�C�^�[�F���� ��
���z�ƁF���c �i
�\���@�F�����~�\���v������
ShopBot��������+CNC���H���́F�H�g�_�C�^VUILD
Treehouse creator: Takashi Kobayashi
Architect: Osamu Nishida
Structure: Jun Sato
�����ݍ��킹�ċȖʂ����ؑg�ł��B


Wood siding pattern of Kigumi could be developed using wooden boards of 60mm thick.
�J�}�L���̗��̂悤�Ȕ�Ώ̂ȋȖʂɁA����60mm�̔ނ��u��������v�����悤�ɕ��ׂ�ؑg�̍\��

��{�̖ؑg�����삵�Ă݂�
CNC���H�@�Ő؍킵�����̂��H�Ɏd�グ�Ă��炢�܂��B
Geometry of Kigumi / 3D printed mock up / Rough carving by CNC
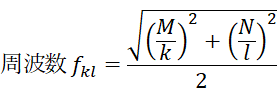


�p�l���`��̓p�����g���b�N�ɐ�������B
3D �v�����g���đg�߂邱�Ƃ��m�F�B
��������188���AVUILD�Ȃǎ蕪�����Ă������200���A���킹��388�������܂ꂽ�B
Panel distribution and carved shapes were generated paremetrically on asymmetric Mantis�f egg surface.
More the digital fabrication technology could be developed,
more we can try collaboration with carpenters to develop precise Kigumi.
CNC���H�@�łł��邱�Ƃ�������ƁA��H������đւ���̂ł͂Ȃ��A��H�̋Z������Ɏ䂫������Ɗ����܂��B
Digital Fabrication ����g���� Parametric Design �����{����ɂ͂܂�������Ԃ������Ȃ���Ȃ�Ȃ�����ǁA�r�����Ȃ����̂ł͂Ȃ��Ȃ��Ă��܂����B
�� Geometry to Provide Natural Forms / Free 3D Metal Form
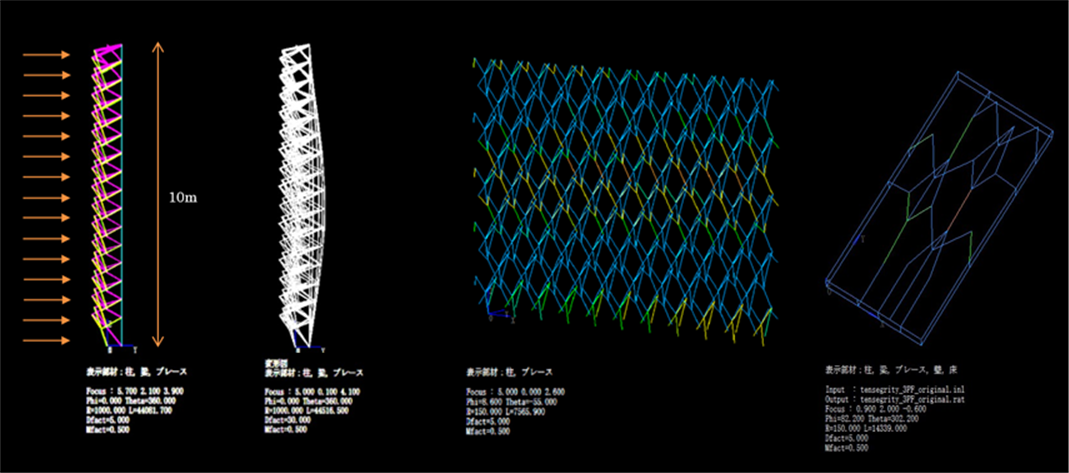
Tensegrity Façade Project �e���Z�O���e�B�t�@�T�[�h
���W���[���{�ڍ���������FKINZI �������
�t���[���{�ڍ���������F������� Heartland Engineering
���������F���j�I�����c
���̃��W���[�����e���V�������b�h�ŕ��V�������悤�ȁu�e���Z�O���e�B�\���v�̃t�@�T�[�h�̒�Ăł��B
���z�Ƃ̕����F�[����Ƃ̃v���W�F�N�g�ɂ��K�p����\��ł��B
���V���W���[���́A�͊w�I�œK�����{���A3D�v�����^�[����g���āu������сv�̂悤�ȃi�`�������Ȍ���ʂ��`����X�^�f�B���܂��B


Partial model
4�[�����}�^���W���[�����P�[�u���łȂ����e���Z�O���e�B�\��
���F���W���[�������T��
�E�F���W���[�������W��
�_�◧�̃��W���[�������݂��ɐڐG�������C���[�łȂ���ĕ������悤�Ɍ�����e���Z�O���e�B�\���ł��B



Module options designed by Tucky
���̃��W���[���́A�P�[�u�����ڑ�����4�_�������ʒu�ɂ���A���R�Ƀf�U�C���ł��܂��B
�^�C�̌��z�� Tucky ������Ȃɒ�Ă��Ă���܂����B



Full scale branch module made of casted stainless steel
Module height = 1.5m
Equivalent cross section
Ellipse pipe 150x90mm, t=6mm at the middle of the branch
Ellipse pipe 180x100mm, t=6mm at the bottom of the branch
3D�v�����g�p���f�� �^ �X�e�����X�ŃL���X�e�B���O���ꂽ����i
����3D�v�����g�͂܂����Ԃ������肷���܂��B
�}�^���W���[����3D�v�����g�����v���X�`�b�N�������b�N�A�b�v�����^���N����
�X�e�����X�𗬂�����Œ�������܂����B
�S12�p�[�c��n�ڂ��Ĉ�̉����Ă��܂��B
���W���[���͐A����A����v�����^�[�ɂ��Ȃ��Ă��܂��B

�\���v�f
Supposed Wind Load = 980 Pa = 100 kgf/m2
Supposed Wind Speed = 66 knot = 34 m/sec
Horizontal deformation due to wind �� around 4cm out of 10m span
Material
Compression Module
Yield stress 325MPa = 3.3tf/cm2
Tension element
Stainless cable 8mm diameter
![]()
![]()
�t�@�T�[�h�S�̂̍\����� �^ 3���W���[���̃��b�N�A�b�v�̍\�����
�ό`�ƌ����i���S���j�̕\���i�ό`�\���{��=30�{�j
���S���i�����j���F�ŕ\������܂��B
���̐}�ł̓I�����W�F���ł����������Ƃ�\���Ă��܂��B
�g�p�\�t�g�E�F�A�FHogan�i�����~�\���v���������j

Multi-objective Optimization using Generative Design
�w�p�����[�^�[ / �����_���Ɍ`���������l�q
�g�p�\�t�g�E�F�A�FRhinoceros, Grasshopper, Bio-morpher, Millipede
��w�@���ɂ��A�}�^���W���[����P����������̓��f�����g���āA
�}���W�܂�u�W���_���W�v
��}�́u��[�����v
��2�������w�p�����[�^�[�ɂ��ė͊w�I�œK�����{����܂����B

Transition of objective functions
�i���^���ړI�œK���̐i�s�ƖړI���̒l
�����ɑ���u����݁v�Ǝ}�^���W���[���́u�d�ʁv���ɏ��������鑽�ړI�œK�����{���܂��B
�ԐF���u����݁v�A���F���u�d�ʁv��\���܂��B
30����̎����v�Z�Łu����݁v�u�d�ʁv�Ƃ������ȉ��Ɏ������܂����B

3 branch modules produced in Thailand
�^�C��3�̎}�^���W���[��������Ă��炦�܂����B


3���W���[���̃��b�N�A�b�v���g�݂�����܂����B
���{���n�ł�150���W���[���قǂ���ׂ�\��ł��B
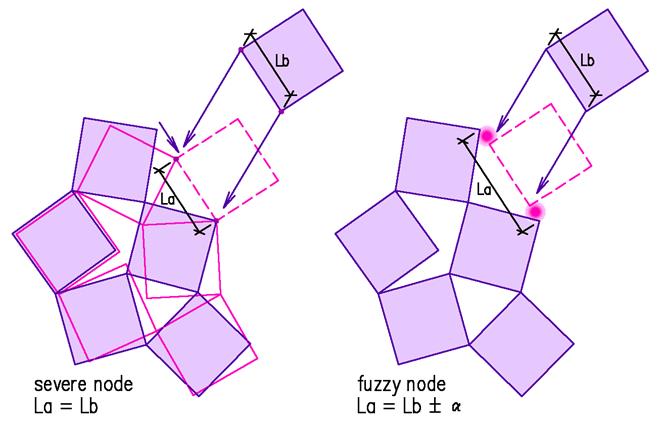
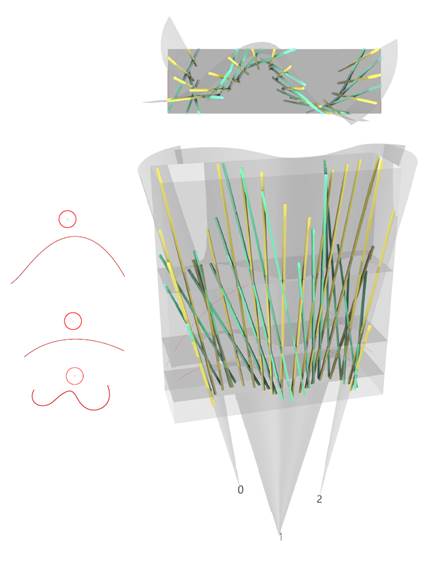
�� Geometry to Provide Natural Forms / Fuzzy Node Algorithm
Transparent Structure as Perceptual Filter
Stanford University seminar and workshop : Winter semester January ~ March, 2015
Tokyo session : June 13 ~ 14th, 2015
Lecturer : Beverly Choe, Jun Sato
Published in GA JAPAN, 2015
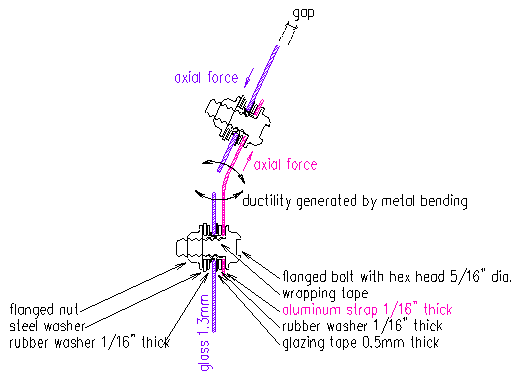
Using 1.3 mm thick engineered, high strength glass panels, connected by an aluminum straps, the installation was formed into a triangulated matrix resembling a 3 dimensional truss and reciprocal compositions.
����́A1.3mm�Ƃ��������������K���X Leoflex, Dragontrail ���g�p�����K���X�\���ł��BReciprocal�CLamellendach �ȂǂƌĂ�鑊�݈ˑ��ň��肷��W�ύ\���ɂ��߂��`���ł��B

���i�ɗn�����ނ悤�Ɍ������߂���\��
Glass: Leoflex and Dragontrail, 1.3mm thick, with holes, with safety film,
size = 600x600mm, 600x440mm, 300x300mm
Fastener: Aluminium straps, rubber washers, metal washers, glazing tape, bolts & nuts


Local geometry to reduce buckling length into 40cm out of 60cm panel




Structural analysis was resulted in the manipulation of buckling length to be less than 40cm.
�ŏI�`��z��������͂ɂ�����������40cm�ɍS���ł���悢���Ƃ���������
These forms are generated by controlling the buckling phenomenon through the geometrical configuration and optimization method.
�E�����͎��Ԃ�������̂ł��炩���ߊJ�������̂���{���狟���B
���[�N�V���b�v�X�P�[���ł��A1.3mm�Ƃ��������̃K���X�ō\�z���邽�߂ɂ͍����̃R���g���[�����K�{�ƂȂ�B
���̔����Ő���������ɂ́A����������40cm�ȉ��ɂ���K�v������A��߂�ꂽ�E���g���Ȃ��烉���_���ɔz�u����ق����A�u���������v��Z���S�����₷�����Ƃ�������܂����B


Vault shape with buttress was developed in Stanford University.
�X�^���t�H�[�h�ł̓��H�[���g�{�Б��o�b�g���X�ō\�����ꂽ

Branching dome was developed in Tokyo.
�����Z�b�V�����ł͐�`�̃h�[���`�\�z���ꂽ


�A���~�X�g���b�v�ɂ��t���L�V�u���Ȑڍ�
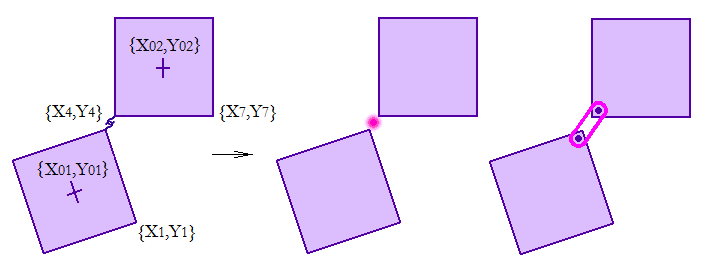
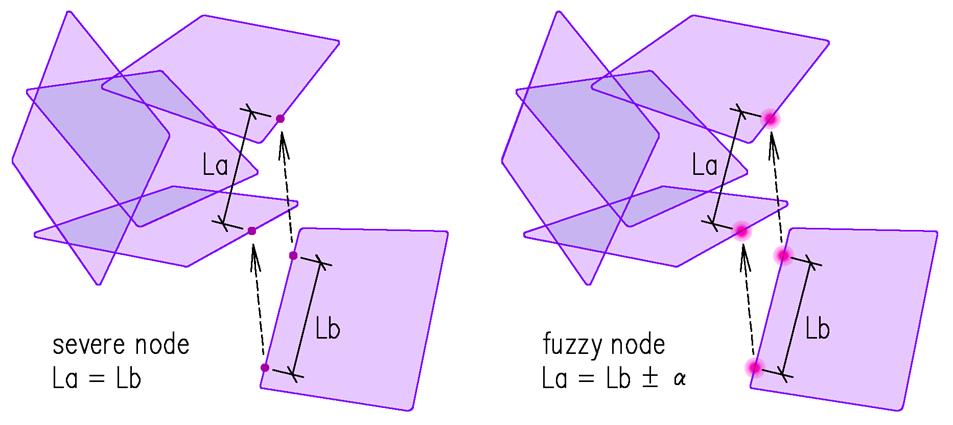
In this system, the Fuzzy Node geometry enables the overall shape to become free form.
���Ђ��W�ς����\����v����Ƃ��A�ڍ��ɋ͂��Ɏ��R�x������fuzzy�Ȑߓ_�ƌ��Ȃ��Đv�ł���ƑS�̌`�̎��R�x�������i�`�������Ȗ͗l�ɂ��₷���Ȃ�܂��B���̂ڂ���Ƃ����ߓ_�� Fuzzy Node �ƌĂׂ܂��B

Fuzzy Node �̃C���[�W
���炩���ߍE���J�������̂����������̂ŁA��߂�ꂽ�E���g���Ȃ��烉���_���ɔz�u���Ă䂭�w��T���Ă䂭���ƂɂȂ�܂����B1���̃K���X�́A���Ȃ��Ƃ�3�����ŗ��߂��Ȃ���Ȃ�܂��A�E���_���ƕ߂炦��Ƃ���قǂ̐�����v����Ƃ͓���z���ł��܂���B
�ł����A�A���~�X�g���b�v���Ȃ��A�Ђ˂��čE�ɓ���܂���ڍ���̂������Őړ_���ڂ���Ƃ����ߓ_ Fuzzy Node �ƂȂ�A���̂������Ŋi�i�ɑS�̌`���`�����₷���Ȃ�܂����B
���܂�ʒu�ɂ���ĈقȂ�`��̐ڍ��������̂͂����s�o�ςłȂ��Ȃ����܂��B���̐ڍ��͂�����\���Ă���ƌ����܂��B



PENTA �̐ڍ����� Fuzzy Node �Ƃ��Đ��삷��ƑS�̌`�̃o���G�[�V���������������ł�
���́A���̊w�������ʏ�ŕ`����悤�ɂȂ邱�Ƃł��B
���ɍ\�z���ꂽ�{�̂ɍނ�lj����邱�Ƃ��l���A�P�_�����Ɗe���Ђ��ǂ��ɗ��������ׂ��������@�\��͂��K�v�ł��B
�Q�̏��Ђ��q��������Ԃ͉��L�̂悤�Ɂu�@�\��́v�̎�@�ōs��\���ł��܂��B
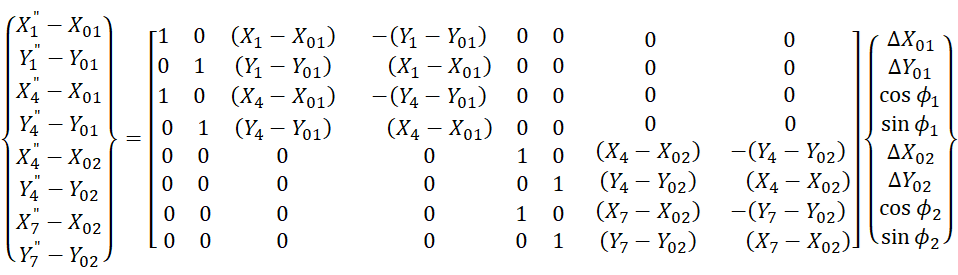
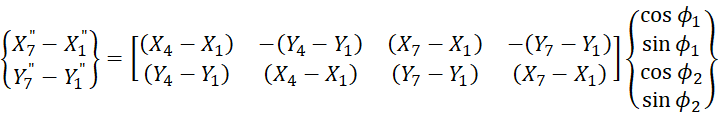
�ڍ��_�� Fuzzy Node �ɂȂ�ƁA���̗v�f���lj�����܂��B����������̓I�ɉ������ɑ��̒l�����߂邱�Ƃ��ł��Ȃ����A�͍����ł��B

������������߂ɁA�P���ɏ����𑝂₷��@�͍l�����܂��B
�u�d�S�̒Ǐ]�v��u���Г��m�̉�]�p�ɊW����lj��v�Ƃ��������@������Ɣ������Ă��܂��B


�d�S�Ǐ]�@�F���钸�_����������ƁA���̒��_�̍s��ւƏd�S���ړ�����Ƃ�������������
����m�F���Ă݂�Ǝv���`�������ɋ߂�


�����v�f����ׂđS�̌`���f�U�C������c�[��
Electron Cloud, Uncertainty Principal or Soft Computing might have relationship with this algorithm.
���̒l����肹���Ɂu�ŗL�l��́^���ْl��́v�ʼn�����@�����肻���ł��B
�ʎq�͊w�̓d�q�_�A�s�m�萫���_�A�s��\���A�܂��� Soft Computing ���Q�l�ɂȂ邩������܂���B
��w�@���ɂ��u��ʋt�s��v���g���ĉ��͈̔͂���肷����@����Ă���܂����B
����́A���j�b�g�̑��B�ɓK�p�ł����@��Nj����܂��B
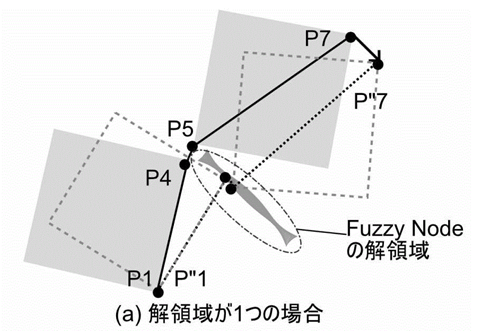
Fuzzy Node P4-P5 �����݂�����̈�
¿ - cube, 2013
Design & Construction : Ken Yokogawa Laboratory, Nihon University
Structural Adviser : Jun Sato
�v��F���{��w���͌�������
�\���A�h�o�C�X�F�����~
Like particles gathering into a protein molecule, 60 mm cubes made of hemlock spruce are connected by �g ¿ - inverted question�h mark shaped eye bolts.
The structure gradually changes from a hard structure at the base to a soft membrane-like structure on the roof.
The distance between nodes should be the
dimension of the cube with factors of x 1, x ![]() ,
x
,
x ![]() .
.
�c�K����60mm�L���[�u���H�`�̃q�[�g���ɂ��A�Ȃ��ɂ����ɂ��Ȃ�B
�L���[�u���R�����Ɏ��͂�`���邽�߁A��{�I�ɂ͗��̃g���X���Ɨ����ł��Ă������ڂŒǂ����Ƃ�����B
1, ![]() ,
, ![]() �̒����݂̂Ō`�������Ƃ������ł���B
�̒����݂̂Ō`�������Ƃ������ł���B



It seems we don�ft have so many choices to compose the global / whole shape with only the 3 distances, but we could feel at the construction site, it is not so hard to add a cube.
It has been found because of the flexibility of the connection which can be called Fuzzy Node.
���_���q���ł����̂ŁA1, ![]() ,
, ![]() �̒����݂̂Ō`�������ƍl���Ă��܂��ƂȂ��������肳��܂����q�[�g���̋͂��ȁu���R�x�v�ɂ���Ċi�i�ɑg�݂₷���Ȃ邱�Ƃ��̊��ł��܂����B
�̒����݂̂Ō`�������ƍl���Ă��܂��ƂȂ��������肳��܂����q�[�g���̋͂��ȁu���R�x�v�ɂ���Ċi�i�ɑg�݂₷���Ȃ邱�Ƃ��̊��ł��܂����B
���Ђ��W�ς����\����v����Ƃ��A�ڍ��ɋ͂��Ɏ��R�x������fuzzy�Ȑߓ_�ƌ��Ȃ��Đv�ł���ƑS�̌`�̎��R�x�������i�`�������Ȗ͗l�ɂ��₷���Ȃ�܂��B
����� Fuzzy Node �̓T�^�I�ȗ�ƌ����܂��B
�ڂ���Ƃ����ߓ_ Fuzzy node

Growth Process with Fuzzy Node
�����̂�lj�����v���Z�X
�� Multi-objective Optimization
���ړI�œK��
�͊w�I�œK�����u�������v�Ȍ`�Ԃݏo���Ȃ���������ɍœK�������u���ړI�œK���v���l���܂��B
Komorebi Workshop in Harvard GSD, 2016-2017
Lecturers: Mark Mulligan, Jun Sato
Published in AXIS, 2017
�u�t : Mark Mulligan(�n�[�o�[�h��w����), ���� �~
PET�������E�H�[�^�[�W�F�b�g�ł��蔲������̌����̂悤�ȃs�[�X��g�ݏグ�܂����B
�u������сv�̂Q�����X�y�N�g������������͗l��ڕW�ɂ��Ă��܂��B


Komorebi Pavilion in Gund Hall, May 2017
Komorebi Pavilion in Autodesk Build Space, January 2017



����̌i�F�˂��ĐF�ǂ�L���ȁu�����X�v�̂悤�ɂȂ�܂����B
�t�̂̂悤�ɂ������܂��B


Final shape of the snow flakes and configuration example
�w���������l�Ă����O���̐�̌����̂悤�ȃp�l��
 ��
�� ��
�� ��
�� 
Algorithm to convert the configuration typology into structural analysis model
����Ȑڍ��p�^�[�����\����̓��f���ɕϊ�����A���S���Y�����l�Ă��܂����B
2�̃h�[�����N�l�N�l�Ƃ��˂��Č�������`����œK�����܂����B

Software component Hogan + Spectrum + Grasshopper + Python
�\�t�g�E�F�A�̃R���|�[�l���g���\�z�����@�̒��
����̃\�t�g�E�F�A�̃v���O�C���Ƃ��ăv���O�������Ȃ��Ń����N������

Software component Hogan + Excel
�`�������c�[���Ƃ���Excel���֗��ł��B





2D power spectrum, provided by the students, similar to Komorebi spectrum
�����̌`��Ɣz�u�ɂ��u������сv�̂Q�����X�y�N�g������������X�^�f�B
�w���������u������сv�ɋ߂��X�y�N�g�������̂ɐ������܂����B


2D power spectrum of Komorebi




���̋��܂Ɣ��˂��D��Ȃ��i�F
�� Super Lightweight Paper & Fabrics / Quick Establishment
Hanafubuki / Ballet de Pétales, 2020
Workshop ENSA Paris Val de Seine + The University of Tokyo
February 17th – 20th, 2020
In 4 days workshop, the students could design and build the tower with a height of 10m, using world-thinnest Washi paper and 3mm slender timbers.
4���Ԃ̃��[�N�V���b�v�Łu�ɔ��a���̑��v�Ɠ����ޗ��Ń^���[�����܂����B


10m tower built in Kashiwa campus, The University of Tokyo
���E�Ŕ��̘a����3mm�̖؍ނɂ�鍂��10m�̃^���[



Colors extracted from plants / Frame form study


3 teams worked on Footing / Body / Head

Lift-up construction process
Veiled Tectonics 2018 : Thistle Leaves – preventing death in the event of collapse
Structural Design Studio, IEDP Integrated Environmental Design Program, the University of Tokyo, 2018
IEDP�u���z�\���f�U�C���X�^�W�I�v�͖��N12���O�オ���C���F�ō\�z�����\�z���܂��B



Semi Tensegrity structure composed of
Super Organza, the world thinnest and lightest fabrics, and 4mm carbon rods.



This structural design could show similarity with the 2D spectrum of autumn woods and pampas grass.
���̃X�y�N�g���́u�g�t�̐X�v�u�������̌��v�̗v�f�������Ă������ł��B
Lightweight and ductile structure will prevent death in the event of collapse.
When we think of natural space, we can think of saving people at the same time.
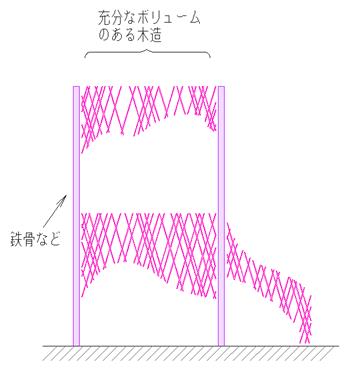
�ׂ₩�ȍނ��D�萬���u�y�ʁv�ȍ\���f�U�C���͍ЊQ�ʼn��Ă����ɗv��l�����ȂȂ��\���ɂȂ肻���ł��B
�����⏰�ɏ[���ɐ[���f�v�X���̂萔�����̍ނŌ`������Ό��z�X�P�[���ł��ł������ł��B
���R�Ȍ`����u�i�`�������v�ȋ�Ԃ�Nj����邱�Ƃ��������u���Ă����ȂȂ��v�\���̒Nj��ɂ��Ȃ��Ă��܂��B
�ɔ��a���́u���v
21 21 Design Sight �ł́u���W�v�W����i

��Y�����̂悤�əR�����u�g�r�P���v��D������ݍ����u���v�ł��B
�u�Ђ����a���v�ł����Ă���A�ꕽ�Ăł킸��1.6g�����Ȃ��A���E�ōł������a�����s���ƒ����āA
�g��ł���ꂽ�킸��3mm���u�q�m�L�v���u�P���L�v�̍��g���������߂܂��B
�₩���u���ؐ��߁v���ꂽ�a���͐܂�d�Ȃ��ĖؘR������u�l���v�ݏo���܂��B
�u�`�a�v���h��ꂽ���g�͘a���̐F�ʂ��������Ă܂��B
���Ȃ₩�ɔ��������g�́A���炩���߈������߂���ƍd�������܂��B
�A�U�~�̗t�̂悤�ȃ��W���[�����W�߂���l�q�͗͊w�I���u�œK���v����Ă��܂��B
�����̌`�ԂɏK����@�� Biomimetics �ƌĂ�܂��B



���m�����Ђ����a�����u���E�Ŕ��̘a���v�������Ă��܂��B
�T����Ƃ�����ނ��u�y���a���v�ł��B
�ɔ��œ����ł������͍ނƂ��Ďg�������ł��B
1mm�̃q�m�L�ō��g�������Ē����Ă݂܂����B
�ނ̒�����s�ϓ��ɂ��āA�[�����łȂ��r�������˂�ƁA�s�v�c�ȏ�Ԃň��肵�܂��B
�Ɍy�ʂȁu�A�U�~�̗t�v�̂悤�Ƀg�Q�g�Q�������W���[�����ł������ł��B
�������������W�߂��g�r�P���́u���v�����邱�Ƃɂ��܂����B
�A�U�~ : https://plumkiw948.at.webry.info/201307/article_9.html


�ɔ��̘a�����u���ؐ��߁v���Ă݂܂����B
�a���͐A���̑@�ۂ�����ݍ����u���f�����v�ł������Ă��܂��B
�G�炷�Ɛ��f�������O��Ă��܂��܂��B
���߂�ɂ͉t�ɐZ���Ȃ���Ȃ�܂���B
�Ȃ̂������獁�q����ɑ��ؐ��߂ɐ������Ă��炦�܂����B

����ȂɑN�₩�ɐ��܂�܂����B

�u���v��傫�����đS��9m�A����3.2m�ɂ��܂����B
���̑傫���ł�3mm�Ƃ����ׂ��̖؍ނłł��܂���3mm�ł͖ؖڂ��ʂ�܂���B
�Ȃ̂ɓޗnj����g�c�������u�g��q�m�L�v���u�P���L�v���ׂ�3mm�Œ���2m�ɔ҂����Ƃɐ������Ă��炦�܂����B
�u�`�a�v�ɂ͖h�����ʂ�����܂��B
�؍ނɓh���Ă������F�ɂ���Ƒ��ؐ��߂̉₩�ȐF�����������܂��B
�؍ނ𑩂˂�1.5mm�̖Ȏ����`�a�Ő��߂Ă����܂��B

�a�����u�f���v���Ёv�Ŗ؍ނɐڒ����܂��B
�؍ނ������������߂Ȃ��璣���Ă䂫�܂��B

�������Ă��炩���ߒe���͈͂Ŕ��点�邱�Ƃ��u�x���f�B���O�A�N�e�B�u�v�ƌ����܂��B
���Ƃ͏_�炩�������؍ނɏd����������Ă��A�s���ƒ������a�����ɂނ܂ł͂��܂肽��݂܂���B
����ƃ��W���[���͍d�����̂Ɍ�����悤�ɂȂ�܂��B



SCI-Arc�Ɠ�����w�̊F�Ń��W���[���̌`�ݏo���܂��B
��������1/2�T�C�Y�̃��W���[����54���ł��܂����B
�F���u�[�_�̐��v�u�ނ̐��v�u�[�_�ɏW�܂�ނ̐��v����ׂ�ƃ^�C�v�����ł��邱�ƂɋC�t���܂����B
�����Ƒ��˕��ł����ԈقȂ�`��Ɍ����Ă��A�Ȃ�������������̂��u�g�|���W�[�v�Ƃ������w�ł͓����ƌ��Ȃ��܂��B
��������15�^�C�v�ɕ��ނ���܂����B


���W���[�����W�߂�1/2�T�C�Y�̑S�̖͌^���ł��܂����B
�Ȃ������l�����ɊF�����R�ɍ�������W���[���ł������܂��Ȃ��邱�Ƃ��ł��܂����B
�ʂ蔲������ʘH���J���܂����B



����̐��삪�n�܂�܂��B
���W���[��1��100g��������܂���B
54���ł�������6kg�ł��B
�P���L�̓q�m�L����2�{���炢�d���ċ����̂ʼn��̂ق��̃��W���[���Ɏg���܂��B


���悢�挻�n�Ő݉c�ł��B
�Ȃ��Ȃ����苭���`���T��܂��B
 ��
�� 
���s���č\����͂ɂ���Č`���͊w�I���u�œK���v���܂��B
�u�`�ԉ�́v�Ƃ��Ă�܂��B
�œK������O�͉�̓��f���ɐԂ��ނ�����シ���邱�Ƃ�\���܂����œK�����ĐԐF���Ȃ����܂����B


���W���[����1.2mm���u���j���v�łȂ��܂��B
�F��������Y��Ȍ��ѕ��������܂����B
�����ł͔����ɗ��߂���̂��g�����Ƃɂ��܂����B
1.2mm�̓��j���͖؍ނɊ�������Ɩ؍ނ����悭����Ŕ����Ȃ��Ȃ�܂��B
�r���L����Ȃ��悤�ɃI�������K�v�ł��B
�u��v���Ђ���Ă��܂����B
��������j�����Y��Ɍ��т��܂��B



�₩�Șa���͐܂�d�Ȃ��āu������сv�̃i�`���������Ɠ����悤�ȃX�y�N�g��������܂��B
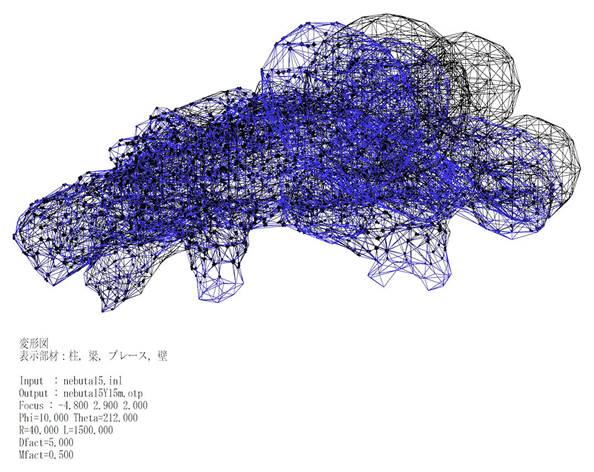
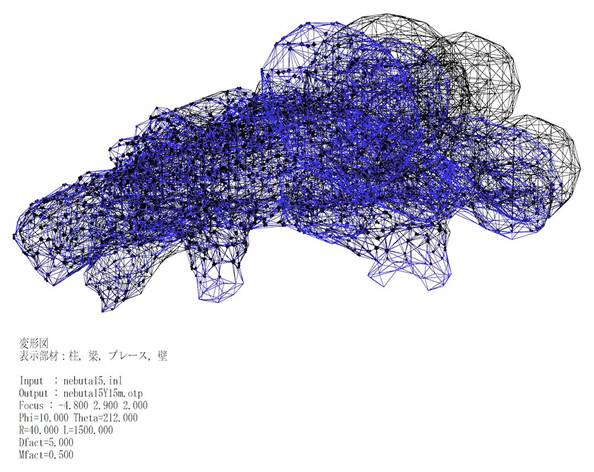
Nebuta Tectonic 2014, 2015 – preventing death in the event of collapse
Structural Design Studio, IEDP Integrated Environmental Design Program, The University of Tokyo, 2014 & 2015
Operations will also result in developing a lightweight and ductile structure which will prevent death in the event of collapse.
From this studio we proposed Nebuta Tectonics composed of steel wire frame covered with Washi paper.
Published in GA JAPAN, 2015 / Shinkenchiku, 2015
�g�˂Ԃ��\���h�| ���Ă����ȂȂ��\��
���z�\���f�U�C���X�^�W�I, ������w���f�U�C�������v���O���� 2014 & 2015
����́A�j���̍��g��a���ł���A�X�́u�˂Ԃ��v�̕\��̂悤�ȉˍ\�ł��B
3mm�̐j���Ń��`�X��̍��g�����A�a���������Ɍ������܂��B
�y���ď_�炩���āA�ЊQ�ʼn��Ă��l�����ȂȂ��A����ȍ\���̒�Ăł��B


Nebuta Tree House, 2015 (Photo by Ying Xu)
Referring to Nebuta, the floats for the festival in Aomori,
this structure is composed of Washi paper and 3mm steel wires.
When the Washi papers are coated with oil, they turn into translucent material. They will work not only for bracing but also serve as Filters for environmental elements.
�˂Ԃ��\���C2015�N�x�́u�c���[�n�E�X�v
�a���ɖ���h��Ɣ������ɂȂ�A����M�ɑ����u�t�B���^�[�v�Ƃ��Ă̖��������悤�ɂȂ�܂��B
�j���́u�����v�Ɓu�Y�����v�𑀂邱�Ƃɂ���āA����Ƃ��̓��j�����ƕό`�����܂��B
Japanese traditional Washi papers are made of fibers of Kozo or Mitsumata plants. It is an organic material, made of only the fibers of plants, without chemical glue. It is strong as the fibers are longer than other papers.
���{�`���̘a���͞��i�������j��O���i�݂܂��j�̑@�ۂō���A�@�ۂ������̂ŋ������ł��B�@�ۓ��m�͐��f���������ł������Ă��鎩�R�f�ނł��B�a���ō������̂ɂ́A�u�˂Ԃ��v�u�a�P�v�u�v�u�����D�v�Ȃǂ��������܂��B
(No Photo) (No Photo)
Nebuta �˂Ԃ�
http://chao01.cocolog-nifty.com/blog/2007/10/post_b822.html
http://tuproduce.blog32.fc2.com/blog-entry-593.html
Nebuta floats in Nebuta Festival, Aomori, Japan are made of Washi paper, steel strings.
�X�́u�˂Ԃ��Ղ�v�ŏ��q����u�˂Ԃ��v�̕\��́A�j���Ō`�����A�a���ŕ����Ă��܂��B�����ɂ͖؍ނ̍��g������܂����A����̍\���͖؍ނ͎g���Ă��܂���B

�a�P
Japanese traditional umbrella Wagasa, representing a lightweight structure,
made of Washi paper coated with linseed oil or perilla oil for waterproof.
The frame is slight and woven with colorful string to prevent buckling.
�a�P�͌y�ʂŏ_�炩���\���̑�\�i�ł��B
�a���ŕ����A�h���̂��߈����m���i���܂ɂ�j��`���i���̂��Ԃ�j���h���Ă��܂��B
���ׂ����g�͐F�ʖL���Ȏ��ŕ҂܂�A���̎��������~�߂̖��������Ă��܂��B


To resist against the first blow in spring (February or March) called Haru Ichi-ban, imagining the wind speed 20 m/sec, we practiced a materially nonlinear analysis, concerning the Washi papers as tension elements and controlling the buckling phenomenon and plastic state of 3 mm steel wires.
In this case the buckling length was found to be manipulated to less than 40cm.
The shape was decided through structural analyses, material strength tests, drag coefficient tests, anchor strength tests.
�u�t��ԁv��z�肵������ 20 m/sec �ɑς���`�Ԃ�ڎw���܂��B3 mm �̐j�����u���������v�� 40cm �ȉ��ɐ��䂷��K�v������܂����B�a�����ޗ������A�R�͌W���̉�͂Ɩ͌^�ɂ��v���A�A���J�[�p�X�N�����[�y�O�̈��������A���d�ɑ����ޗ�����`������o�āA��C��R�̏��Ȃ��`������肵�܂����B
Captured model

Drag coefficient analysis by Flow Design (Autodesk), experimentation
�R�͌W�����v�����AFlow Desgin (Autodesk) �̉�͂Ɣ�r����


Washi paper tensile test / Screw peg plucking test
�a���̈��������^�A���J�[�̈�����������
Materially Nonlinear Analysis
�������ʂf���čޗ�����`��͂��s�Ȃ�



Nebuta Tree House, 2015 (Photo by Ying Xu)
�˂Ԃ��\���C2015�N�x�̃c���[�n�E�X
When the Washi papers are coated with oil, they turn into translucent material. They will work not only for bracing but also serve as Filters for environmental elements.
Lightweight and ductile structure will be applicable also for Lunar Base and Mars Base.
�a���ɖ���h��Ɣ������ɂȂ�A����M�ɑ����u�t�B���^�[�v�Ƃ��Ă̖��������悤�ɂȂ�܂��B
�j���́u�����v�Ɓu�Y�����v�𑀂邱�Ƃɂ���āA����Ƃ��̓��j�����ƕό`�����܂��B
���̂悤�ɁA���l�ȍޗ��łł����ʍނ�b�V����̍\�����u�i�`�������v�ȋ�Ԃݏo���Ȃ���A�ׂ��ȍނłł��Ă��邨�����ōЊQ���u���Ă����ȂȂ��\���v�ɔ��W����\���������Ă��܂��B
�� Lightweight and Ductile Structure: Little by little, learning Great Nature



Lightweight and ductile structure will prevent death in the event of collapse.
When we think of natural space, we are thinking of saving people at the same time.
Although it is impossible to know everything of great nature, it is impossible to control great nature, when we learn the great nature a little more, we can save people a little more.
���ׂ��ނłł������ߐ�����\�������ݏo���u�i�`�������v�ȋ�Ԃ�Nj����邱�Ƃ��A�ЊQ���u���Ă����ȂȂ��\���v��Nj����邱�Ƃɂ��Ȃ�܂��B
���Ə������R��m���Ă�����A���Ə��������l����������ɈႢ����܂���B
�� Technical Aid Projects
�x������
Handmade Retaining Walls in Barrio Cantera, Argentina, 2018
Architectural assistance : Akiko Okabe Lab, The University of Tokyo
Technical assistance : Jun Sato Lab, The University of Tokyo
Support project in Barrio Cantera, San Martin de los Andes, Argentina, where is exposed to landslide every year. We could build handmade retaining walls, collaborating with local people using local materials, to resist soil pressure when the cliffs fell down.
�A���[���`���� San Martin de los Andes �ɂ���X�����uBarrio Cantera�v�̎x��, 2018�N5��
������w�����������̃X�����x�����͂��܂����B
���N�������n���肪�N����Ζʒn�ɏZ�ݒ������X�����̂��߂Ɂu�e�Y����v�̎R���ǂ��\�z���܂����B


Retaining Walls in Barrio Cantera, San Martin de los Andes, Argentina, 2017
(Photos : Claudia Sakai, Akiko Okabe Lab, University of Tokyo)
http://www.viviendaneuquen.gov.ar/site/web/site/detalle?id=21
The wall is composed of small I beams inserted into the ground, and wooden sidings in between.
The walls were painted by the kids fascinatingly.
�n�ʂ�H�`�|��˂��h���A�ؐ��̉�������荞�܂��܂��B
���Ƃ͒n�������Ƃ��ɓy������Ȃ��悤�ɂ��邽�߂̍H�@�ł��B
�q�������̃y�C���g�ɂ��₩�ɂȂ�܂����B
House for All in Rikuzentakata, Venice Biennale 2012
Awarded: Golden Lion
Architect: Toyo Ito, Kumiko Inui, Sou Fujimoto, Akihisa Hirata
Structure: Jun Sato
For the recovery project from the Tohoku Earthquake 2011,
the logs of the cedar trees that were exposed to Tsunami could be composed into the meeting house for the people.
This project was exhibited in Venice Biennale 2012.

House for All in Rikuzentakata / Exhibition in Venice Biennale
https://www.inuiuni.com/projects/482
House for All in Toyono, 2018
�F�{�������x���Z�� �{ �݂�Ȃ̉�
�v��@�@�F2018�N
�v��n�@�F�F�{��
�ӏ��v�F�H���a���{�x��O�^�V�[���J���X�j���g
�\���v�F�����~�\���v������


�b�����̕����Z��
�c�i�q�̑ϐk�v�f�̃��b�N�A�b�v
���̍\���ł͕ǔ{��1.5���x�̐��\������ƕ��������B
�F�{�n�k�̕����Z��̃v���W�F�N�g�ł́A�c�i�q�̑ϐk�v�f���Ă��Ă���B���V�̐��╝�𑝂��ƌ��ʂ��傫���B����Ɏ�Ԃ�������̂Ő����Ȃ߂ɂ����g�p�ł��Ȃ����A�ׂ��ȍނō\�����ꂽ����������ؑ��ϐk�v�f�̉\����Nj�������̂ƂȂ��Ă���B


�L�쒬�̋��������Z��u�݂�Ȃ̉Ɓv�̘a�P�\��
Community Week 2014
Dhillon Marty Foundation international workshop in Punjab, India
Schools : The University of Tokyo, Stanford University, The University of Oregon, Rhode Island School of Design, Guru Nanak Dev University
Students from : Japan, U.S.A., India, China, Greece, Columbia, Indonesia
Public Toilet Design Competition in 3 days
5 clusters of students proposed the public toilet design.
Public toilet represents the social problems in India as follows,
Sanitation on water, foods, streets
Gender problem such as safety against crimes for ladies
Gap between rich and poor

Design Build Workshop in 2.5 days
Design build team was composed with 2 or 3 spies from each 5 clusters of students.
A kind of private space, also imagining the public toilet, was designed with some elements extracted from those design proposal of 5 clusters. The spies had to bring those informations from each clusters.
We can design structural elements which also work as environmental elements by designing filter for light, heat, air, water, sight, insects, person.
Keywords Delivered: water filtering / air ventilation / use waste for fertilizing / natural material / lift up the floor



Shopping for materials and tools
Materials : local fabrics, bamboo, metal wire, strings, metal bars, plywoods, screws
Tools : saws, pliers, hand drills, hammers, needles, screwdrivers



Studies on bamboo frames
Brick and timber for lifted platform.



Instable frames stabilized by fabrics
Mesh structure with semi-transparent fabrics for filtering light and sight
Cellular spaces by branching membrane



Final shape with 15m length, indicating a gate, lifted private room covered with layered filters,
rest space, air ventilater.
�� Super Lightweight Structures
Balloon, 2007
Architect: Junya Ishigami
Structure: Jun Sato
Aluminium �gballoon�h of 14m height, weighing roughly 1 tonne.
The balloon with aluminium lattice endoskeleton, filled with helium gas.



Architecture as air, Venice Biennale 2010
Awarded: Golden Lion
Architect: Junya Ishigami
Structure: Jun Sato
Rigid frame structure composed of 0.9 mm CFRP columns, 1.2 mm CFRP beams could be stabilized
by invisible bracings made of 0.02mm polyalyrate fibers.
Architecture as air in Arsenale / Buckling analysis model
http://contessanally.blogspot.jp/2010/08/venice-12th-biennale-of-architecture_26.html
�� Membrane Tensegrity / Omission Technique of Engineering
���e���Z�O���e�B / �G���W�j�A�����O�͏ȗ��̋Z
Big Art, Exhibition Archi-neering, 2008
Designed and constructed by students
Structure: Jun Sato et al.
Tensegrity structure composed of a membrane supported by carbon (CFRP) pipes.
�A�[�L�j�A�����O�W �g Big Art �h, 2008�N
�J�[�{���̖_�̒��ɓ˂����Ă邱�Ƃɂ���Ĉ��肷��S��12m���u���e���Z�O���e�B�v�\���B
�����ނɂ���ƒ��͂̓`�B�ɍL���肪���܂�A�Ǝ��̌`�Ԃ����܂��B
�J�[�{���́A���e���������x��I���ł���B���e���̂��͓̂S�̂Q�{�قǍd���A�����ɋ����̂ň��k�ނɑ��������B
���̓c�[�E�F�C�\�t�g�Ƃ������i�ŁA200�����x�̐L�є\�͂����B
MOOM (Membrane Oom), 2011
Design & Construction: Kazuhiro Kojima Laboratory, Tokyo University of Science
Structure: Jun Sato
�ӏ��{�{�H�F�������ȑ�w������_������ �� ���l������wYGSA������_�������p��
���́F���z�H�Ɗ������
Membrane tensegric structure composed of membrane and aluminum pipes.
Membrane work as tension wires and pipes are working as compression particles.
Length 26 m, Span 8 m.
���Ɉ��k�ނ��\��t�����`�������e���Z�O���e�B�\���ł��B
���k�ނ̓r���ɂ��������͂����z���邱�Ƃɂ���ēƎ��̌`�Ԃ����܂�܂��B
�S�� 26m�C�� 8m���h�[���͔��Ɍy�ʂ����d�� 600kgf�A40�l���x�Ől�͂Ō��Ă��܂��B
�E�G���W�j�A�����O�̎�@
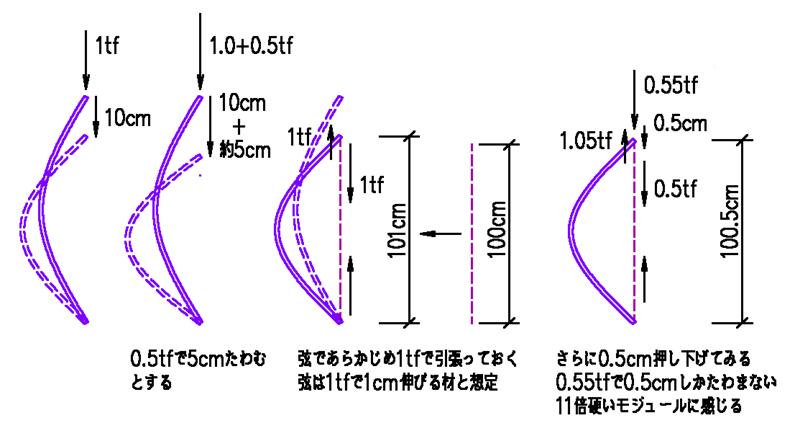
Structural calculations which I have provided were only these written on this paper at a meeting.
Omission is one of the techniques of engineering.
Engineering is a technique of omission. We still confront unidentified phenomena. We never have enough time to try all the calculations we desire. We try to suppose a simplified model with fewer calculations and figure out if the structure is feasible. This aspect has to be acknowledged by society.
�w���������l�������̍\������������ɂ������čs�Ȃ����v�Z�́A�ł����킹�̍ۂ��`�S���̎��̔����ɏ������������̃��������������B
�N���e�B�J���ȕ��������ɂ߁A�ɂ߂��P�������ꂽ���f�����v�������ׂ�A�ȑf�Ȍv�Z�ł��ꂪ�������邩�ǂ������ʂł���B

�S�̂����������`������߂�͔̂���`��͂ɂȂ邪�A�ŏI�`���z���ł�����̕K�v�͂Ȃ��B
�f�v�X�ɂ���Đ��܂�鍄����\�z���A�A�[�`�̍������x�Ŕ��f����B
�ȗ���^����Ɩ_�����������グ�Ē��͂�����B�������Đܔ\���ɋ߂��`��ɂȂ�f�v�X�����܂�A�A�[�`�̍����ɑς����鍄�������܂��B�v�Z������ǂ��Ă݂�B
�A�[�`�Ƃ��Ĕ������鎲�͂��v�Z����B
�d���� w = 5 kgf/m2 �Ƃ���B
�X�p�� L = 8m�A���� H = 3m �̃A�[�`�Ƃ���B
�_�̃s�b�`�� 1m �Ƃ���B
�A�[�`��������� y = ��x2 �Ƃ��� y = 3 �Ax = 8/2 = 4 ��������ƁA
��= 0.1875
�A�[�`�̑����ł̌X���� y�f= 2��x = 1.5 �Ȃ̂ŌX���� 1 : 1.5 ���ƕ�����B
�A�[�`�S�����12m�Ƃ��āA�����ł̉������͂́A
5 kgf/m2 �~ 1 m �~ 12 m �� 2 = 30 kgf
����Ǝ���N�́A
N =  �~ 30 kgf = 37 kgf
�~ 30 kgf = 37 kgf
����ɑ��A���x���v�Z����B
�؍ނ̊ۖ_��25mm�Ƃ���ƁA�f�ʐς́A
A = 4.91 cm2
���܂��f�v�X�� 100mm �Ƃ���B���̒f�ʐς͕s�������A�؍ނ̖_�Ɠ����Ƃ��Ă݂�ƁA�f�ʂQ�����[�����g�́A
I = 4.91 cm2 �~ 52 cm �~ 2 = 245 cm4
�����O���͍d���������x�� E = 20 tf/cm2 �Ƃ���B
�A�[�`�̍������� Lk = 0.4L�`0.5L ���x�ƒm���Ă����Ƃ悢�BLk = 0.5L = 0.5 �~ 800cm = 400cm �Ƃ���B�����d�́A
Pcr = ![]() =
= 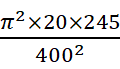 = 0.302 tf
= 0.302 tf
����� N = 37 kgf �ɑ��ĂW�{�̗]�T������̂Ŗ��Ȃ��ƕ�����B
���������ꂾ���̉���Ɛ����ɕ����ׁA�d����������̂�10����������Ȃ��B
�����������f�����̊��o��g�ɂ��A����ꂽ���ԂƔ�p�̒��Ō������ڂ����ɂ߁A�������\�ɂ����u�ȗ��̋Z�v�����u�G���W�j�A�����O�v�ł���A���̋Z���ӔC�������Nj���������Љ�̂��߂ɔ����ł��Ȃ��Ȃ��āA���E�I�Ɋ�@�ɂ��炳��Ă��邱�Ƃ��Љ��F������Ȃ���Ȃ�Ȃ��B




�ŏ��̓A���~�p�C�v���}�����ꂽ����n�ʂɕ~���B
���͂��F�ň͂݁A�[���̃A���~�p�C�v���N�����Ȃ���A�����������������グ��Ǝ��R�ɖc��ށB
���̃h�[���́A���k�n�������m���n�k�i�����{��k�Ёj�̔�Вn�ł̎x�������̏W��ȂǂɊ��p����Ă���B
�� Stained Glass Structure
�X�e���h�O���X�\��
Design & Research: Jun Sato Laboratory, University of Tokyo
Structure in Architecture is appearing diverse forms composed of diverse materials, constructed by diverse methods, and exposed to diverse impacts.
The stained glass panels are made by fixing glass in a slight metal frame, which represents the diverse forms in the manner mentioned below.
As we can see it is sufficiently complex composite to develop a dynamics operation, when completed, it can be adopted for many of other structures.
�ׂ��������g�ŃK���X���S�������X�e���h�O���X�\���̌����B���l�ȍޗ��ƌ��ۂ��\���̑�\�Ƃ��ĕ߂炦�A�͊w�`�Ԃ𑀂�v�@�̍\�z��ڎw���Ă��܂��B
Left, Middle : Stained Glass Structure test specimen
Right : Pop-up Stained Glass using brass frames, Workshop 2012, Jun Sato Lab
���R�Ȑ��͗l�ƙ^�͗l�̃X�e���h�O���X�\���̎�����
As the cushioning materials inserted between the glass and metal frame are required to be resistant to UV damage, we are using tin plates which we found to be effective.
���O�����Ȃ��ɏՍނƂ��āA�������ʓI�Ȃ��Ƃ��Ă��Ă��܂��B
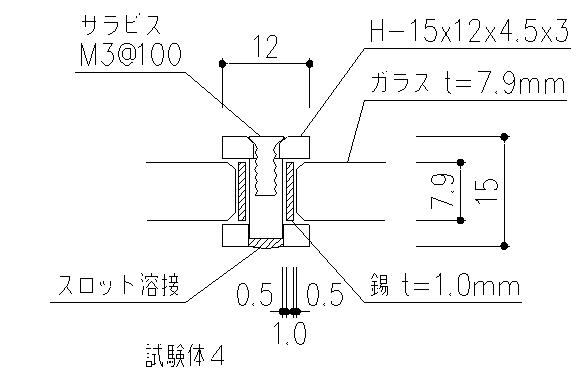
Joint Detail
���O�����Ȃ������ɏՍނɂ���[�܂�
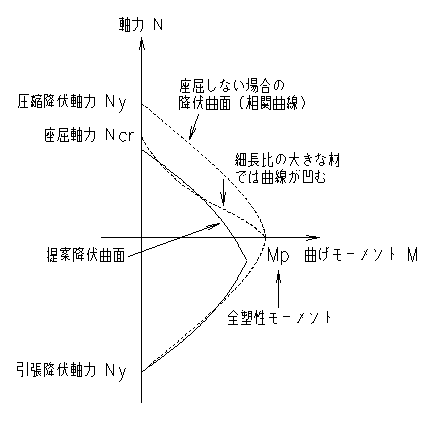
Perfectly Plastic Surface Diagram
Axial force – Bending moment interaction curve considering buckling phenomenon
�������l�������~���Ȗ�
�ނ��ׂ��Ȃ��āu�����v�̉e�����傫���Ȃ�ƁA���͂ƋȂ��Ȃlj��͂̑��Ȑ��u�~���Ȗʁv�����݂܂��B������u�Y���q���W�v�̋����ɍl������K�v������܂��B


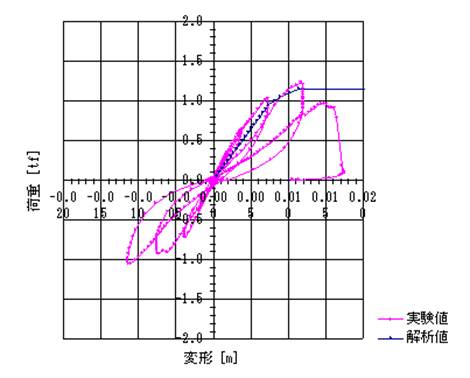
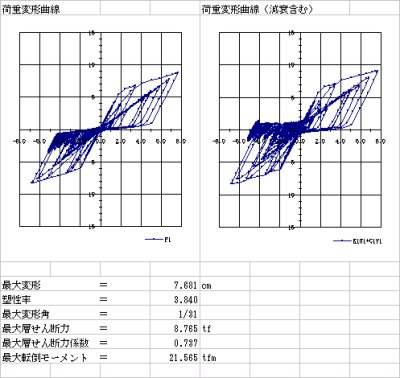
Tuning up the simplified model to fit to the loading test
���ރ��f���ɂ�闧�̍�����́C�����̉d�ό`�Ȑ��C��͂ɂ��d�ό`�Ȑ��̍Č�
�������ʂ��Č��ł����̓��f�����\�z���邱�Ƃ��A���̍\���𗝉����v�@���\�z���邱�ƂɂȂ���܂��B
�����̍ޗ��A���ނƖʍށA�ɏՍނ̃X�Y���Ԃ�Ă䂭�Ƃ����u���������v�A�K���X�ƍ��g�ނ̑��݂��u�����⍄���ʁv�A�Ƃ������\���̃��f�������Ă��܂��B



Apartment House in Wasedatsurumaki, 2015, Architect : Yoshio Sakurai
Published : Nikkei, 2017
����c�ߊ����̏W���Z�� (2015�N�C���z�ƁF�N��`�v) �Ŏ������܂����B
�� Glass Welding
�K���X�n��
�K���X��n�����A�唻�܂����V�[�����X�ȃh�[���⑽�ʑ��삷����@���Ă���B
�ڕW�́A�ڒn���݂̂�1200�����x�ɉ��M���ėn�����A���̂Ƃ����\�Z���`���ꂽ�������S�s�����퉷�ɗ��܂邱�ƁB
���ꂪ�����ł���A����ł̑唻�̗n�����\�ƂȂ�B
Published: JIA journal, 2015 & 2016

�K���X�[�����O���ɘI�o�������n�����u�C�n������������
�K���X�͗�p�����A�j�[�����O�ƌĂ��530�`550�����x�ł̉��x�ێ��Ŏc���Ђ��݂��ɘa����B
���̉��x�ł͕��q��������蓮����B
530�����x�ȉ��ł͕��q�̈ړ����قƂ�NjN���Ȃ��Ƃ������Ƃł�����B
2015�N�x�A�ڒn��1000���A���Ӊ��x 120 ��������30cm�̃K���X���m�̗n���ɐ��������B
�����530��������鉷�x�̈悪���ӂɑ��݂ł���\���������ꂽ�B
�����I�ɖڒn���𑖍�����u�n�����u�v���J������ꍇ�ɁA���u�̕���50cm���x�ł悢�\���������ꂽ�B
���݁A�傫�ȃK���X�ł̎����̂��߂ɑ�^�F���������B

���x����

���x���z�̌��z
�c���Ђ��݂��Č��ł����̓A���S���Y���̊J�����J�n�����B

�Z�i�������@�ɂ��c���Ђ��݂̉摜�Ɖ�͂̔�r
2013�N�x�A�����V�̑��Ɛ���ɂ����āA����̗n�������݂�ꂽ�B

����ɗn����������i�C�n�����u
���̑��Ɛ���uGLASS CUBE�v�ɂ��A�����V���u�����B���Y�܁v����܁B
2015�N�x�A�����S�Ƃ̑��Ɛ���ɂ����ċȖʃK���X�̗n���ɐ��������B


�X�����s���O�ɂ��Ȃ����K���X��n�������A�[�`�ƃh�[��
�� Glass Shop
�K���X�\��
Extreme Nature, Venice Biennale 2008
Architect: Junya Ishigami
Structure: Jun Sato
Four greenhouses were built around Japan Pavilion in Giardini della Biennale
using slight and rigid jointed steel frame covered with glass sheet.
���ׂ��������͍|���K���X�ō\������郉�[�����\���B
�A���̂��߂Ɍ��z�̑��݊��𔖂����邱�Ƃ�ڎw���܂��B


Greenhouse of 2 m height with 16x16mm columns and 8mm glass walls
Greenhouse of 6 m height with 32x32mm columns
To provide a transparent structure, we used ultra-high strength steel for the frame, and used glass walls as tension bracings.
The columns are located randomly, and a form optimization could contribute to detecting their location.
First model
Frame using super high-tensile steel
Heating process using gas burner
Screenshot of form optimization software
Development of Manual Form Optimization Software is contributing for optimised location of columns.
�u���Ԃ�v���g���ċɍׂɍi�����S�����[�����\���ƃK���X�\���̉���
�ї����钌�̈ʒu���œK�������u�蓮�`�ԉ�́v�̃\�t�g�E�F�A���쐬���܂����B

fix-fix fix-hinge hinge-hinge fix-fix+sway fix-hinge+sway
��=0.5 ��=0.708 ��=1.0 ��=1.0 ��=2.0
Buckling strength : Pcr = ![]()
E: Young�fs modulus [tf/cm2]
I: Moment inertia of section [cm4]
Lk: Buckling length Lk =��L [cm]
Deformation, Bending stress diagram of rahmen structure due to gravity
Heat cambering of the steel is an important process in the fabrication of the structure as it reduces deformation as well as bending stress.
�u���S�v�ƌĂ��u���Ԃ�v�̋Z�p���g���ĕό`�ƋȂ����[�����g���R���g���[�����邱�Ƃ��ł��܂��B
Process to reduce deformation and bending stress
Iz House, Shizuoka, Japan, 2005
Architect : Sou Fujimoto
Structure : Jun Sato
Stacked structure of glass walls and acrylic resin walls.
A simple structural analysis model was developed for seismic response analysis.



Exterior and interior under construction, Structural Test
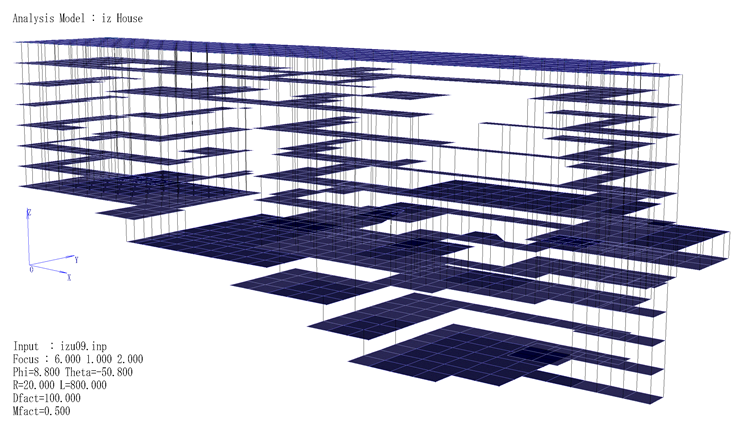
Structural Analysis Model

Section Drawing


Details



Load displacement curve, Seismic response analysis
�� Lattice3 = Lattice�~Lattice�~Lattice
3D Printed Lattice3 Model / Eoffel Tower (Photo : Jun Sato)
Eiffel Tower is a composition of Lattice2, that is lattice made of lattice.
Lattice3 with optimized form will become able to be manufactured in these years.
�p�`�|�ǂ̓��`�X�����邱�Ƃɂ���ēS���ʂ����炵�������ɂ��邱�Ƃ��ł��܂��B
�G�b�t�F�����̓��`�X�̌��ނƎނ�����Ƀ��`�X�ɂ��Ă��̓����x�����Ă��܂��B
���܂̃f�W�^���Z�p�Ɨn�ڋZ�p����g����Ƃ����P�i�K���`�X�����邱�Ƃ��ł������ł��B
�I�[�X�g�����A�̃v���W�F�N�g�Œ�Ē��B
Ponds des Art / Gare de Lyon (Photo : Jun Sato)
19���I�ɂ͓S���������������ߎ�Ԃ������Ăł����`�X�����邱�Ƃ��o�ϓI�ł����B
(No Photo) (No Photo)
Pennsylvania Station 1910 / Gare de Tours 1898
�� Concealed Structural Design
�B�������\���f�U�C��
��������p��w���p�فE�}����
2010�N�v�H
�ӏ��v�F���{�s��z�v������
�\���v�F�����~�\���v������
�K���@�@�F�n��Q�K�C�n���P�K
�\���@�@�F�S����
�{�I���Q������ɔz�u���ꂽ�}���فB
���ʖ{�I�̌��ԂɓS���̔����炢���[�����\����[�߂Ă���B
��b���}�C�����}
����������ɔz�u����Ă���B
�u�ו��g�`�|�v�łł��������炢�u���[�����\���v��{�I�̌��ԂɎd����
�p�`�|�ǂ̂��肵�����ɔ�ׂāA�w�������Đ��������Ă��A�S���ʂ͂��܂葝���������������ł���B
���[�����\���ƌ����Ă��o���G�[�V�����͐������l������B�Ȃ��ł��u���[�X���g��Ȃ������[�����\���͏_�炩�ȍ\���i�_�\���j�ƂȂ�B����𐬗������邽�߂ɂ͂ЂƍH�v���K�v�ɂȂ�A���ꂪ�`�Ԃݏo���B


�Ȃ����[�����g�}�𗧑̓I�Ɍ���B
�g�`�|�̒��͋��������ɍd���̂ŁA�e�����̒n�k�ɑ��ċ����������Ă�����̂������Ă���l�q��������B
�����炢���ނ��Ȃ��S����Ƃ��͉������ɒ��ӂ��K�v�ƂȂ�B
�g�`�|�̒��r���u�������r�v�Ƃ��A�n����̂ɖ��ߍ��܂�Ă���B
�����ɗї����钌�B���������̗l�q��������B
�\���̂قƂ�ǂ͖{�I�Ȃǂɂ���ĉB������Ă��܂����A�\�������z�̌`�Ԃݏo�����ʂ̈�[��S���Ă���B
�u�B��ĂȂ�������\���f�U�C���v�ƌ�����B
�� Metal Shop
�����\��
PENTA
Architect: Kotaro Imai Laboratory, University of Tokyo
Structure: Jun Sato Laboratory + Yoshihiro Fukushima
����3D�v�����g�Ŏ��R�p�x�̐ڍ�����������đ��ʑ̂��`�����܂��B



���̐ڍ����ɂ� Fuzzy Node �̋@�\���d���ނƑS�̌`�̃o���G�[�V���������������ł��B
Copper Shell in Earth: Material for Design by The National Museum of Emerging Science and Innovation, 2010
Design & Build: Jun Sato Laboratory, University of Tokyo
A copper shell structure with 8m long fabricated by only hammering from a flat plate, with 40 students.
Considering the total energy consumption for this structure to appear, we discovered the energy consumption in processing the copper shell by hammering was only 7 %, of the total energy, while 93 % for manufacturing copper plate.
Copper Shell in the Exhibition Earth: Material for Design, 2010
���l�����̐X���� Zoorasia ���X�g�������C�Q�[�g��
�ӏ��v�F�R�{�����v�H��
�\���v�F�����~�\���v������


���X�g������
�|�ɂ��V�F���\��
��⤏�̒��̓V�F���Ɉˑ����Ă��܂��B

�|��FB�i�t���b�g�o�[�j���u�~�D�ʁv���`�����܂��B


�t���b�g�o�[�̊i�q�ɔ��|���������ĂĒe���Ȃ������Ȃ���n�ڂ��܂��B

�Q�[�g��
�����̌`�ɂ��蔲���ꂽ�|���������x���ϐk�v�f�ɂ��Ȃ��Ă��܂��B
�O���͖ؑ��s�����ň͂��Ă��܂��B

�S���Ɩؒ��̎{�H�̗l�q
Research Building, Hakodate Future University, 2005
Architect: Riken Yamamoto
Structure: Jun Sato
Steel mesh structure composed of vertical and diagonal elements.
Mesh tectonics shows the craftsmanship is necessary to generate these structural, environmental elements.
�����͂����Ė�����w������
���z�ƁF�R�{ ����
�\���F���� �~
�c�ނƎނō\�������X�`�[�����b�V���\��
Welding tecnique and reforming tecnique is necessary to fabricate these mesh.
�u�n�ځv�Ɓu���Ԃ�ɂ�鋸���v�̋Z�p������Ȃ�����̂悤�ȃ��b�V���͍��Ȃ��B

���̍�����́A�\���������o�Ď������邱�Ƃ��ł����B
Tsuda Veterinary Clinic, 2003
Architect: Kazuhiro Kojima / CAt
Structure: Jun Sato
Shelf shaped sturcture with 6mm plates, without backboard by controlling 3 dimensional buckling.
�c�_�E�W���E�C�J
���z�ƁF���� ��_�^CAt
�\���F���� �~
6mm�̍|�ɂ��w�̂Ȃ��I��̍\���B




When flat bar columns are located in radial arrangement, buckling strength can be found 4 times bigger than parallel arrangement.
���̍�����͂ɂ��A�t���b�g�o�[�̗���ˏ�ɔz�u����Ɖ����̐����ړ����~�܂�A�����d�����s�z�u�̂S�{�ɑ������Ƃ����������B

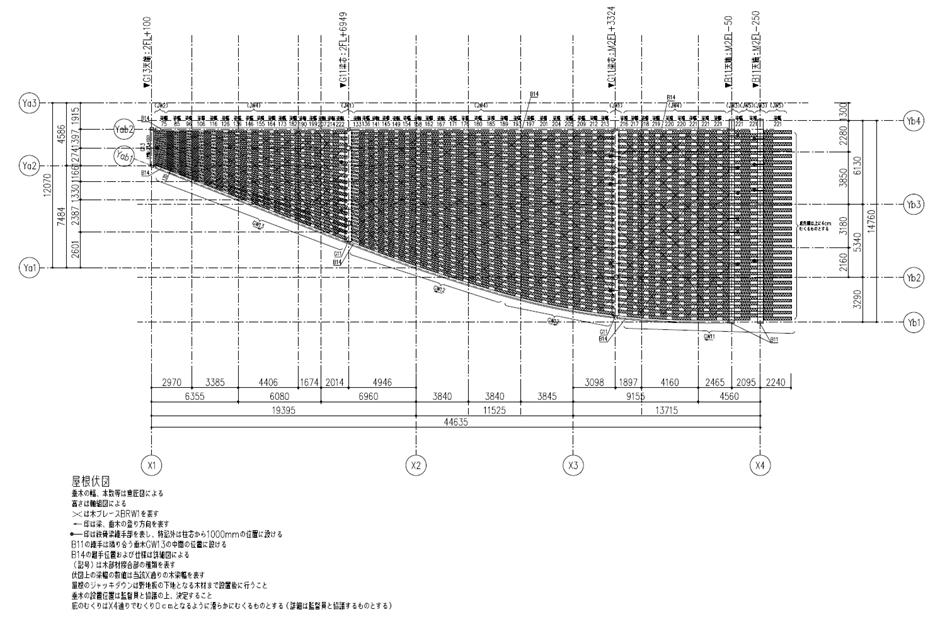
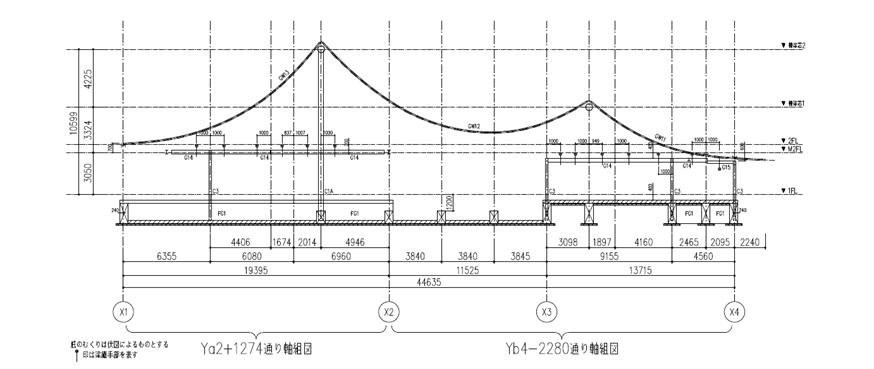
Buckling control of flat bar columns : Radial, Polygonal, Parallel
�̔z�u�ɂ������̐���F���ˏ�C���p�`�C���s

Elevation of each grid structure based on buckling phenomena
In some case, forms generated by the optimization of buckling appears not visualizing the stress flow.
���z�ƂƃA�C�f�A������Ƃ́A���z�Ƃ��ڂ���Ǝv���`���Ă��邱�Ƃ��u�������Ă�v���Ƃ̂悤�Ɋ�����B
������Ȃ��X�P�b�`�ł��ǂ�ǂ�`���Ă݂�B�������Ă��邤���ɍ��������݂����߂�\�����v�������B
�ŏ��͒��ꕷ���̂ł͂Ȃ��A�u�u���[�X�̂Ȃ����[�����\���Ƃ��Ă݂悤�v�u�ނ̃s�b�`�͂Q���Ƃ��Ă݂悤�v�Ə���ɑz�����Čv�Z���n�߂�B�������Ă������������ăC���[�W�����L���Ă���A�u�i�q�ǂɂ�����ǂ�ȃT�C�Y�ɂȂ邩�v�u�����ƍׂ����ނ���ׂ���v�Ə��X�ɑN���ɋ�����Ă䂭�B
�����ŁA�ł����킹�̂��̏�Ōv�Z�����@���𗧂B���G�Ȍ`��ł��P�������čl����قƂ�ǂ̍ނ̃T�C�Y�����̏�Ō��߂邱�Ƃ��ł���B���������݂��Ɏ~�߂����@�\��m���Ă����������łX�����łȂ��U�����łł���\��������ƕ��������B
�I���W�i���̃\�t�g�E�F�A�̊J�����𗧂��Ă���B�c��Ȑ��̎��Z�̌J��Ԃ��ŃA�C�f�A����邱�Ƃ��ł���B
���̏�ł̊ȑf�Ȍv�Z �� MOOM
Rest House in Zoorasia
Architect: Riken Yamamoto
When flat bar columns are located in radial arrangement, buckling strength can be found 4 times bigger than parallel arrangement.
���̍�����͂ɂ��A�t���b�g�o�[�̗���ˏ�ɔz�u����Ɖ����̐����ړ����~�܂�A�����d�����s�z�u�̂S�{�ɑ����B

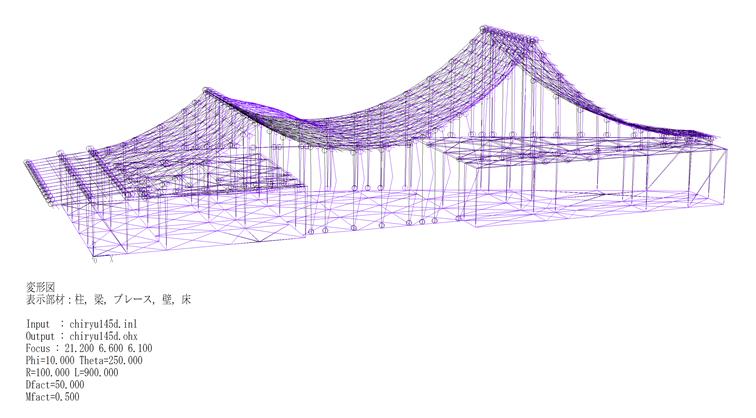
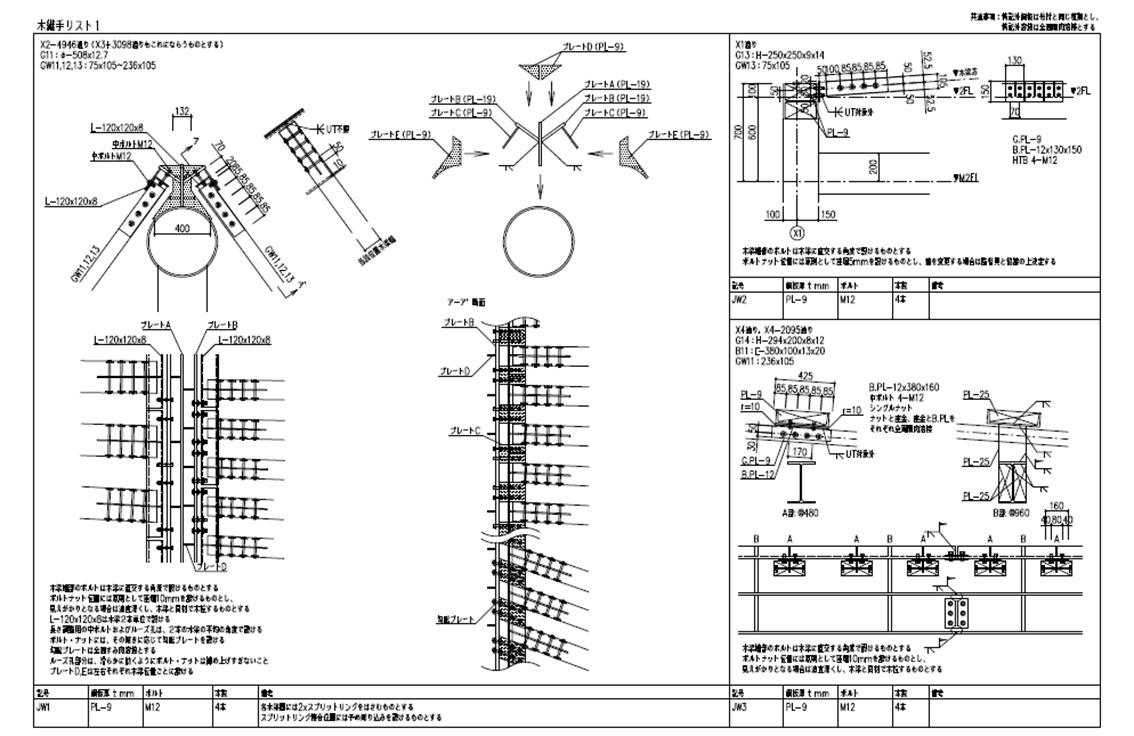
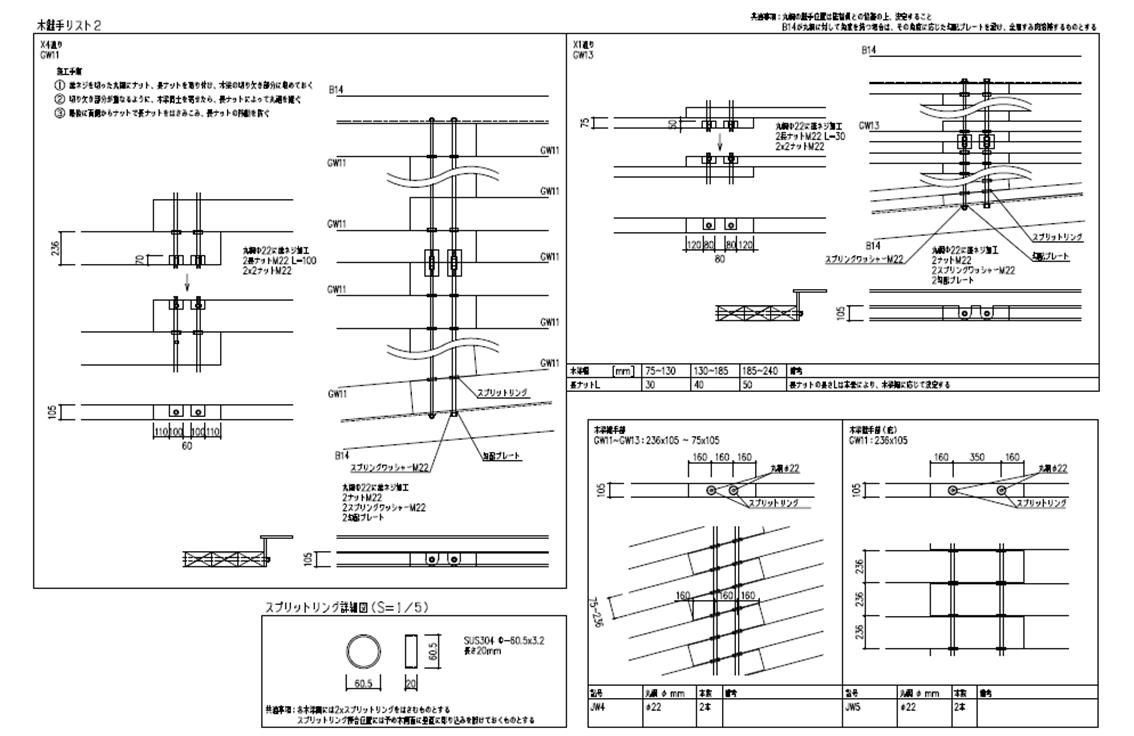
Buckling control of flat bar columns: Radial, Polygonal, Parallel
�̔z�u�ɂ������̐���F���ˏ�C���p�`�C���s
NYH, 2006
Architect: Makoto Yokomizo
Cylindrical steel plate wall structure stacked up to 4 strories.
�m�x�g : 2006�N�C���z�Ɓ����R�~�]�}�R�g
�e�K�قȂ�z�u���~�`�̕ǂ��A�S�K�ςݏグ��ꂽ�\���B
�~�`�ǂ́A9mm�̓S�Ƀ��u FB-16x38 @300mm �����č���Ă���B
Steel plate 9mm thick with reinforcing flat bar 16x38@300mm
��3.4m�C����2.9m�C����11m�̃����t���A���A�����łQ�������������̂Q�p�[�c�ōH�ꐻ��B����Őϑw����B
Buckling strength of thin plate comes bigger when the curvature got bigger.
���ǂ́A�ȗ���^���邱�Ƃɂ���č����ɋ����Ȃ�B�ȗ����a���p�����[�^�[�Ƃ��č������x�̃O���t��`���ƁA���a���������Ȃ�Ɣ���I�ɋ��x���オ�邱�Ƃ�������B
Structural analysis model, Buckling strength - Curvature diagram
��̓��f���S�̐}�C���S���̐F�\���}�C���u�t���Ȗʕǂ̍������
�� Timber Joinery Development
Prostho Museum Research Center, 2010
Architect: Kengo Kuma
Structure: Jun Sato
1st examination of Kigumi with Kengo Kuma
Timber 3D grid structure without metal fixings at joint.
Starbucks Coffee in Dazaifu, 2011
2nd examination of Kigumi with Kengo Kuma
3D diagonal grid acting as a hunched portal frame.




Library and Social Care Center, Yusuhara, 2018
������ �}���ف{�Љ���{��
�v��@�F2018�N
�v��n�F���m��
���z�ƁF�G���ጚ�z�s�s�v������
�\���@�F�����~�\���v������
�G����Ƃ̖ؑg�V���[�Y��4�e�B�_�炩�ȓS���̃��[�����\���ɁA4�{�����̖ؑg���܂Ƃ����܂��B
4th examination of Kigumi – timber joinery without metal fixings.



�ؑg�̕����n�k�ɂ̂������邱�Ƃɂ���ĔR����Ȃ��Ō����ɂł��܂��B
3D diagonal grid developed inside steel frames
Its geometry is not an imitated form of tree but showing some naturalness.
�X�іL���ȓ������ŁA���X�K�͂̑傫�����̌v��ŁA�ؑg����g�ł��Ȃ����ƒT���Ă݂܂����B
�ω��v�������v��Ŗ؍ނ�������ɂ́A�n�k�ɂ������������@������܂��B
�d�͂ɑ��ẮA�S���\�������������ƍl���܂��B
�n�k���ɂ��������Ȃ��Ă悢���̂Ƃ���Αω�v������Ȃ��̂ŔR������핢���K�v����܂���B
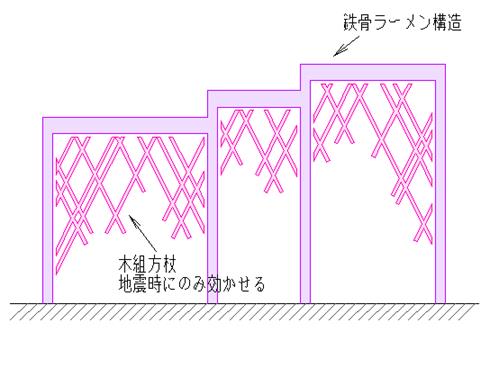
�؍ނ�ϐk�ɂ̂���������@�̂ЂƂA����`��
�S���̃��[�����\���̓����ɖؑg�̃{�����[����Y�킹�邱�Ƃɂ���āA�ؑ�����Ƃ��Č������܂��B
�ނ͑S��120mm�œ��ꂵ�Ă��܂����A�����ƒ����Ȃ��ނ���ނŁA����Ɍ�������ނ������⍄�ނ̖�����S���܂��B
The frame has to be made of steel because of fire regulation.
Haunching braces of timbers work only against seismic load and wind load.
When the timbers work only for earthquake, not for gravity, fire matter doesn�ft have to be considered.



4�{�̍ނ����S��1�_�Ō���闧�̕H�`�i�q
�i�q�ɑg�ނ��Ƃɂ���č������x�������A�ނ��i�邱�Ƃ��ł��A�ؑg�̓����x���グ�邱�Ƃ��ł��܂��B
Center axses of 4 timbers are crossed exactly at one point.
Parallelogram grid with random spacing can be composed with same angles.
3D grid can increase buckling strength so that the timbers can be slender to be 120mm.
A constant angle was selected but spacings are random to create tree pattern.
We can generate natural pattern of forest with this geometry, without using imitated shape of trees.
�����p�͂�⎩�R�Ɍ��߂��܂����A�p�x�͓��ꂷ�邱�ƂƂ��܂����B
�ނ̃s�b�`���o�[�R�[�h��ɕs�ψ�ɂ���ƌ������̍��ݕ��͑S�ē����Ȃ���A����̖͗l�ݏo�����Ƃ��ł��A�����ɂ��̍\���̎��R�x������Ă��܂��B
���`��^����̂ł͂Ȃ��w���Ɠ��̎���͗l�ݏo���܂��B
�� Timber Catenary
Gunma Agricultural Technology Center, 2012
�Q�n���_�ƋZ�p�Z���^�[
2012�N�v�H
�ӏ��v�FSALHAUS�i�������C�����i�C���V�����j
�\���v�F�����~�\���v������
�����ʐρF2400 m2
�K���F�n��Q�K


���b�V����i�i�q����j�̖ؑ��������e���g�̂悤�ɒ������\���B
���̍\���͎�ɁA
�E�R�{���S���x��
�E���̊Ԃɒ���ꂽ�ؑ�����
�E�[�������������e���V�������b�h
�ō\������Ă���B
�����́A�X�M�iE50�j90 x 75 mm @ 450 mm ���Q�i�ɕ��ׂĂ���B
�ؕ��ނ́A�d�ʂɂ���ď������ꂽ��ԂŒ�����B�Ȃ����x�����������x������ޗ��łł����A���d���ނł́A���͂�^����������i�ό`�j�����炵���ق����傫�ȉd�ɑς�����B
�܂��A���\���ɔ�ׁA�d���ނ��Ă��邱�Ƃ͕��ɂ��㉺���o�^���i�t���b�^�[�j��}������ʂ����҂ł���B

�P�����F�Ȃ����[�����g�݂̂���������B
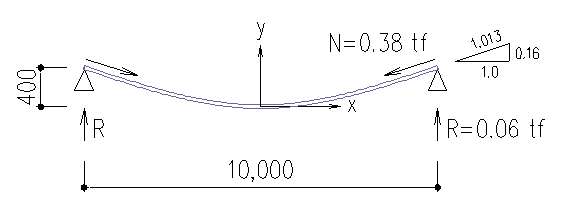
���͂�^����Ƃ���݂�����B
�Q�l�F�j���ɃI������݂邵�A�[�����X���C�h����悤�ɒu���Ă���Ɛ܂�Ă��܂����A���[���������莝���Ă���Ɛ܂ꂸ�ɂԂ牺����B
���ꂽ�`����w�I����`����ɂ�苁�߂Ă���B�iNewton-Raphson�@�j
�ڍׂȉ�̓��f��

�ؑ����N���[�v�����C���J�m�\�[�v�e�B�u�����ɂ��ό`�̐i�s�ɔz������K�v������B
�����ŁA1�N3�����ɓn��A4m�̍ނ��������葱���鎎�����s�����B
���������̗l�q�B4m�̍ނ�3tf�̒��͂�^���������B
�C���C���x�̕ϓ�
�؍ނ̊ܐ����̕ϓ��F
23�`8���ŕϓ������B
�؍ނ̐L��
�ؕ��ޒ[���ڍא}
�����ό`��̐L�т�2mm���x�ƂȂ����B
�N�Ԃ̐L�т͔N���o��ɏ]���ė��������Ă��邱�ƁA���͂����ۂɂ�1�`2tf�ł��邱�ƁA���l�����ĔN��1mm�ƍl����ƁA����20m�̍ނł͔N��5mm�A10�N��50mm�ƂȂ�B
�����ōޒ[��100mm�̃N���A�����X��݂���Ώ[���ƍl���A�����̒��ߒ������\�Ȕ[�܂�ɂ��Ă���B
�Ȃ��n�k���ɂ́A
���ԕ����ł̓e���V�������b�h�C�S�����[����
���s�����ł͓S�����[�����C�ؕ��ނɂ�鐅���u���[�X
������ϐk�v�f�ƂȂ��Ă���B

���͂�^���A�ނP�{��20m���˂��n�������̗l�q�B
���c�����w�Z
�v��@�@�F2017�N
�v��n�@�F��茧
�ӏ��v�FSALHAUS�i�������C�����i�C���V�����j
�\���v�F�����~�\���v������


�u���c�����w�Z�v�C���z�ƁFSALHAUS
�ϐ�d�Ԃ�����b�h�ł��炩���߈������낵�Ă����ƁA�ϐ�d�ł̂���݂��ɂ߂ď������B
�u�x���f�B���O�A�N�e�B�u�v�̐��������p���Ă���B
�m���̎��q��
�v�H�@�F2016�N
���z�ƁF���c�^�G�C���c�����^MOUNT FUJI ARCHITECTS STUDIO
�\���@�F�����~�\���v������
�����ʐρF744.70 m2


�m���̎��q��
https://www.fuji-studio.jp/
�؍ނ����݂ɕ��ׁA���{���g�ŖD���A������J��Ԃ��č���q�̂悤�ȏ_�炩�Ȍ����ʂ��`������B
����1500mm�C����105mm�œ��ꂵ�A����65.9�`220.6mm�ƕω������āA��`�ɕ����L�����Ă䂭���ʌ`�ɏ�ɓ���̐��̍ނ���ׂĂ���B

�������}

���g�}
�ؑ��������x�������͓̂S�����Ƃ��Ă���B���������̒��_���x�����钌�ɂ͗�������̒��͂��o�����X����̂ő傫�ȉ��͂͐����Ȃ��B�[���ŋ�������������̂ɑ��Ă̓u���[�X�t���̃��[�����Œ�R���Ă���B
�� ����`���
���ʂ��Ώ̌`�łȂ����߂ɁA���������`��͂�╡�G�ȋȖʂ�`���B����������X�^�[�g���āA����ė��������`������߂����`��͂��s�Ȃ����B��̓��f���}�ŁA�����̒����ʼn����̂ق�����O���������ł���B
�S���X�^�b�t�ɂ��쐬���ꂽ�ANewton Raphson�@�̈��ł���Co-rotation�@�����ǂ����A���S���Y���̔���`��̓v���O�����ɂ��B

����`��͌���
��̓��f���̉����́A�P���Ȋi�q�Ƀ��f�������Ă���
�� ������́C�n�k�����
��L�ŋ��߂��`��ɑ��Ē����d���������ꍇ�̔���`��͂��s�Ȃ��B
�����ł̓t�@�T�[�h�̃}���I���������k�ɑς�������̂Ƃ����̂ŁA�e���̉����̒�����������ށB
��������݂̍ő傪1.8cm���x�ƎZ�o���ꂽ�̂ŁA�N���[�v�A���J�m�\�[�v�e�B�u�A�ϐ�d���펞�͍ڂ�Ȃ����ƁA���l�����āA�؍ނ�u�����ׂ邽�߂̒�K�̍�����3cm�̂ނ����^�����B���ۂ̂���݂�4cm���x�������Ă��A�ڕW�ʒu����1cm�̉�����ł���Ζ��Ȃ��B����ŃW���b�L�_�E�������Ƃ���2cm���x������A�ϐႪ�Ȃ����Ƃ��l����Ɨ\�z����⑽�߂��������A�ُ�Ȃقǂł͂Ȃ��ƍl������B
�n�k���ɂ͊e���̉����̒��������c���悤�ɗh��邪�A�傫�ȕό`�ł͂Ȃ��B

������͌��ʁF�ό`�}�C50�{�\��

�n�k����͌��ʁF�ό`�}�C50�{�\��
�� �V�A�[�R�l�N�^�[���̃��f����
�؍ޓ��m�̐ڐG���ɂ͒��{���g���Q�{���ʂ��āA���̍E���ɃV�A�[�R�l�N�^�[�Ƃ��ăX�v���b�g�����O���d����ł���B
���S�ȃs���ڍ��łȂ��A�����������������A���ɑ���t���b�^�����O���N���ɂ����悤�ɂ��Ă���B
���̕������l�����āA�V�A�[�R�l�N�^�[�́u�A�\�r�v�Ɓu�߂荞�݁v���瓙�����������߂��Ƃ���A�؍ނ̒f�ʂQ�����[�����g��0.3�`0.5�{�ɂ��邱�Ƃɑ������邱�Ƃ����������B�����ŁA��̓��f���ł͑S��0.3�{�Ƃ��Ă���B
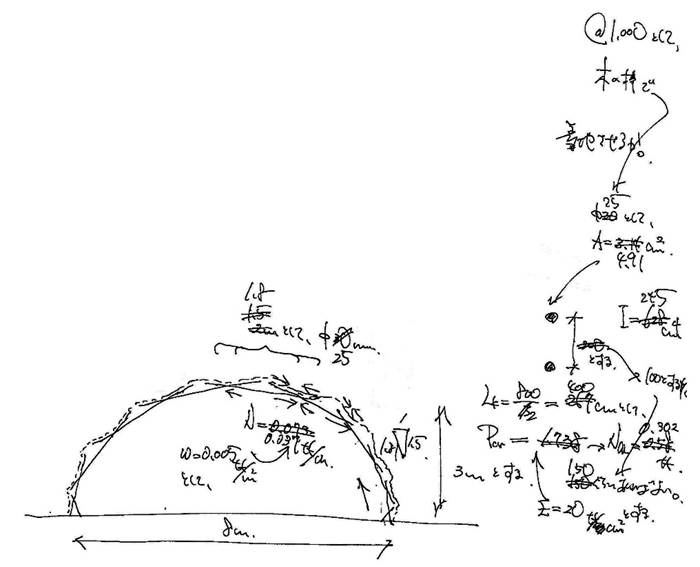
�� �؍ނ̉��H
��`�ɍL�����Ă䂭�؍ނ������`�ɕ��ԂƁA�p���Ŗʂ�����Ȃ����߁A���{���g�ł̒��ߕt���������Ȃ��Ȃ�B�ڐG�ʂ��m�ۂ��Ȃ���A�v���̑z��������H���₷���`�A�{�H�҂����Ă��Ă����������B
33�i�ڂ�31��̖؍ނ̌`��}
�ЂƂ̖؍ނ́A���̐}�ʼnE�ׂ̖؍ނƂ̊����i���F���j�����B
�S�Ă̖؍ނɂ��āA���̂悤�Ȑ}��`���Ă����������Ƃ��ł����B



�� Wood Shop
�؍\��
Yamaga Elementary School, 2013
�R�����w�Z
�v��@�F2013�N
�v��n�F�F�{��
���z�ƁF�H���a���{�x��O�^�V�[���J���X�j���g
�\���@�F�����~�\���v������
���a�ނŌ`�������u�싞���o�ʗ���v�̉ˍ\�ł��B
���a�ŒZ���ނ��A�p�x���������ς��Ȃ���A�˂Ă䂭�Ɠ싞���o�ʗ�����ɍL�����悤�ȉˍ\���`������܂��B
�S�̂��傫�ȃf�v�X�̃��`�X��ɂȂ�A���������炩�ȋȐ���`���Ă��ꂪ�A�[�`�Ƃ��Č����Ă��܂��B
�e���Ƃ���{�I�ɉ����ȊO�̍\���͂q�b�Ƃ��A�ؑ��������ڂ��A�����I�ɖؑ������̓r�����x�����邽�߂ɖؑ��ˍ\�̋r�����낵�Ă��܂��B



The large span structure composed of slender timbers to have a shape of Nanjing MusoTamasudare.
Timber configuration is optimized to generate arch effect as well as lattice effect.

Nanjing MusoTamasudare
�싞���o�ʗ�




Branching joinery / Tenon / Gymnasium
Specific mortise and tenon could be developed for this joinery.
�l�X�Ȋp�x�Őڍ�����ޒ[�ɂ͒E�����Ȃ��悤�ȓʌ^�̃z�]��݂��Ă���A���ꂪ�R���̑�H�ɂ��f���炵�����x�ō��܂�A�g���Ă��܂����B
��{�I��4m�ށA6m�ނō\������悤�ɂ��Ă��܂����A�n���̎R�ŃX�g�b�N���[�h�̐E�l�ɕ����ƁA�u�����p�i240mm�j�v���炢�Ȃ�10m���炢�̍ނ͓�Ȃ��̂���ƌ����܂��B������āA�̈�ق�240mm�p��7m���x�̍ނ��ӂ�ɗp���邱�Ƃ��ł��܂����B

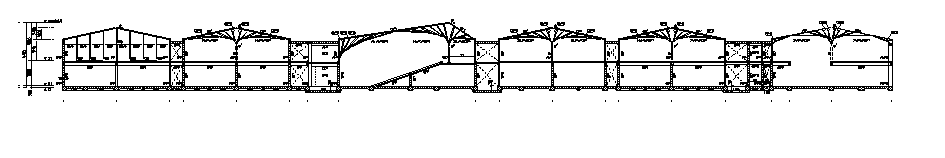


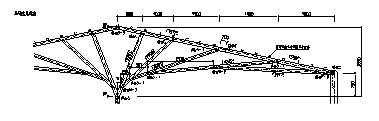
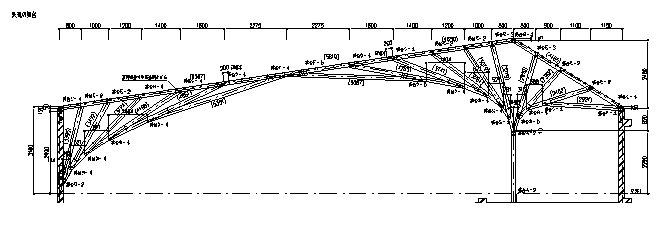

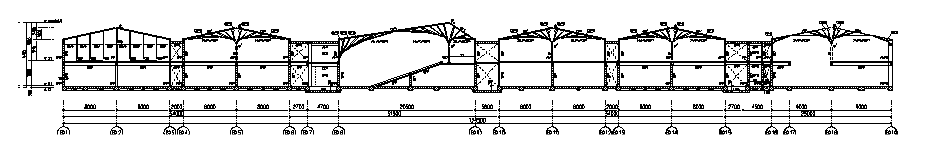

Tamasudare pattern could be adjusted to span, shape and boundary condition of each room.
Dimensions of the timber could be 105mm, 150mm, 240mm respectively.
�����A�}���فA�̈�قȂǁA�X�p���ɂ���ĕ��ނ̃T�C�Y��105mm�p�A150mm�p�A240mm�p�̐��ނƂ��Ďg�������A�܂��x���_�̈قȂ�����ɂ���ċʗ��̖͗l��K�������Ă��܂��B
�������`���Ȑ��ɂ���āA���܂������̎��͂�`�B�ł��邩�����܂�܂��B
�_�o���ɂȂ肷����K�v�͂Ȃ�
�u�{��X���w�Z�v���z�Z�p2017�N5�������
���ԂɂȂ�قǁA�����ؑ��ō\�����邱�ƂɐS�z����������������B�ؑ��̐v���́A�ǂꂪ�D���Ƃ����ߓ�悤�Ȃ̂ŁA��ɐ������̌��ۂ�z�肵�ċ��x���m�F���Ȃ���Ȃ�Ȃ��B���ꂾ���̌��_������Ƃ������Ƃł�����A�����ǂ�ł���Ɠr���ɕ���B
����ł�����d�˂閈�ɁA�����������_�͔���������̂ŕK������������Ȃ��Ƃ����Ȃ����̂ł��Ȃ��ƍl���Ă���A�����ߏ�ɐ_�o���ɂȂ���̂ł��Ȃ��ȂƊ�����B
(1) �����F�ނ̒����ɍގ������ɒf���I�ɑ��銄��͔������Ȃ����̂ƍl����B�h���t�g�s����}������ɓ��銄��͌������̂ŁA�{�H�̑����i�K�ʼn\�Ȃ���ւ��邪�A���������̓G�|�L�V�����𒍓�����B
(2) �ɂ��F���k���Đڍ������ɂނ��Ƃ͍l���Ă����B�{���g�͏������ߒ����A�h���t�g�s���͊ɂ݂ɂ������ɂ�ł��E�����Ȃ������ɂ��Ă����B���Ԃ��ł����疄��}������B
(3) ���H�F�ǂ�Ȃɕ��H������Ă�����Ȃ��O��ōl���Ă͂����Ȃ��B�����̍ނ̎��ւ����@���l���Ă����B�p�肪�h���t�g�s���ڍ��ł���V���v���ŁA����ꂽ�͈͂��ȒP�ɊO�����Ƃ��ł���B
(4) �N���[�v�F�ό`���傫���Ȃ镔���̓��N���Ă����B���N��Ȃ������Ƃ��ɝ��݂��傫���Ɩ��ɂȂ邪�A���N�肪����Ȃ��ă}�C�i�X�ɂȂ��Ă��\�z�͂��đĂ����Ƃ������Ƃł������ɂ͂Ȃ�Ȃ��B
(5) ���B�F�ǂ�Ȑ��@�̖؍ނ��ǂꂾ���̍ސώg����̂��A���̌���̐E�l���H�ȂǎR�ɋ߂��l�X�ɕ����Ǝg����ނ̖L�x����m��Ƃ�������B�ł����ꂪ�����ł͂Ȃ�����A����قǓ����I�ȍނ�����ł��Ȃ��Ƃ��́A�ނ��낻�̏�����ƕ߂炦��B
(6) ���͓`�B�F�ߍނ̉��͂��������ɓ`���Ă�����̉��͂ƒނ荇�킹��Ƃ������`�B�ɒ��ӂ���B��������̂���f�͂̓`�B�����G�ȏꍇ������B
���f����
�������Č��Ă݂�ƁA�Ō�́u���͓`�B�v�͐_�o���g�����̂́A���͂����������̂ł��Ȃ����Ƃ���������̂ł͂Ȃ����Ǝv���B��K�͖ؑ��̉\�����L����F���Ƃ��Ė𗧂ĂƎv���B
��D�n���h���Z�c����
�v��@�@�F2018�N
�v��n�@�F��茧
�ӏ��v�FSALHAUS�i�������C�����i�C���V�����j
�\���v�F�����~�\���v������
�ߑ�Ɍ����邮�炢�т̔w��傫�����A�������������ł����ށB�����ɂ����ѐڍ��̓��[�����ƃt�B�[�����f�B�[�����`�����邱�Ƃ��ł���B
�ѐڍ��͍ޗ������̗ǂ��ڍ��ł͂Ȃ����̂́A�������͂͐s���Ȃ��B���߂̒��ɋɒ[�ɍו��̗��A�Ƃ����Â��ǂ��v���|�[�V�������Ɠ��ł�����A�N�T�r�Œ��߂�ȑf�Ȏd�g�݂��f�p�Ɍ�����B�Z�I�I�Ȗؑg�`��̍��݂ł͂Ȃ����̂́A���H���x�̍����ƃN�T�r�ɂ���ăX���b�v�̏��Ȃ������ڍ������������B



�Q�i�C�S�i�̊т���g�����ю����[�����\��
�؍ނ́A�X�M�̐��ނł���r�I�������̂�����ł������Ƃ̏�������B���Ƃ���270mm�p�Ȃ琻�ނʼn\�A300mm�p���Ə��X���������W���ށi�X�M�W����E65-F225�j�Ȃ��Ȃ��g����A�Ƃ����������B�єw��300mm���炢�Ȃ琻�ނʼn\�A�W���ށi�J���}�c�W����E95-F270�j�Ȃ�360mm���g����Ƃ����B���ꂾ���������ނ��g����Ȃ琻�ނ����ł���Ă݂悤�Ǝ��Z���Ă݂��Ƃ���A�т͊T�˂Q�`�R�i�A�Ƃ���ǂ���U�i�łقƂ�Ǖǂ̂悤�ɂ���A�Ƃ�������ƕ��������B�v���|�[�U���i�K�ł͂��̂悤�ɐ��ނŒ�Ă��Ă������A���̒n��͏W���ނ����Y���Ă���̂ōŏI�I�ɂ͏W���ނ��g�p���čސ������炷���Ƃɂ����B
�т�360mm�ŊT�˂Q�`�S�i���x�ƂȂ�A�S�̓I�ȓ��������������ˍ\�ƂȂ����B



���Q�̐��x�Ŋт�}�����A�N�T�r�Œ��߁A�������U�{�ł�����
�N�T�r�ɂ̓P���L�A�����ɂ̓J�V���g���B
���k�ŃN�T�r���ɂ�ł����ݒ���
���̃��[�����\���������͂ɒ�R����Ƃ��A�т���]���Ē����тɂ߂荞�ށB�㉺�̂߂荞�݈ʒu������Ă���قNjȂ����[�����g���傫���̂ŁA�т̔w���傫���قNj����B�����ŋɒ[�ɔw�̑傫�Ȃ��̂��g���Ȃ����ƍl���A���肵�₷�����ő傫��360mm���g�����Ƃɂ����B
�����͑���30mm���U�{�ł����߂�B�������č����ɂ��Ă�����Ȃ��Ԃ�͕K�v�Ȓi�����d�˂�悢�B


���r����

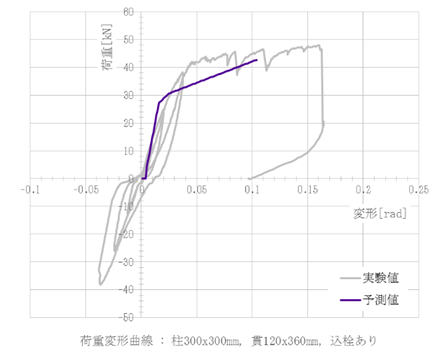
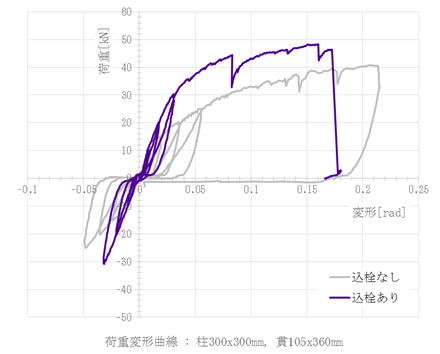
�d�����̋Ȃ������C�d�ό`�W
��=300x300�C��=120x360�C��������C�����^�\��
��=300x300�C��=105x360�C��������^�����Ȃ�
�тƍ����̍��Z���\�Ȃ��Ƃ��m�F
�u�ʂ��ѐڍ��v���A�u�����v���A���{���z�w��́u�؎��\���ڍ����v�}�j���A���v�ō����Ƒϗ͂̎�����Ă���Ă���̂ŁA������K�p����B�������A���Z�����@�͋L�ڂ���Ă��Ȃ��B�т��������ǂ�����@�ے�������ɂ߂荞�ޕό`�𗘗p���Ă��茘���̍������Ȃ��̂Ō����^�C�~���O�����Ă��邾�낤�Ǝv����̂ƁA�߂荞�݂̔����ʒu���قȂ�̂ŁA���܂�^��Ȃ����Z�ł���̂ł́A�Ǝv���A�m�F���Ă݂����Ǝv������������s�����Ƃɂ����B
����300mm�p�̃X�M�W���ނɓ��ꂵ�A�т�105mmx360mm��120x360mm�̃J���}�c�W���ނ̂Q��ނƂ����B�т�105x360mm�̂ق��ŁA�u��������v�Ɓu�����Ȃ��v�������Ă݂��B�Ȃ��A�����ł͍������p�_30x30mm�Ƃ��Ă��邪�A����ł͊ۖ_��30mm�Ƃ��Ă���B
�����ŁA�����̂����ꂼ��Q�̂��Ƃ��Ă���B�����̂��R�̂łȂ��Q�̂ł悢�Ɣ��f�ł��邱�Ƃ͎����̂��Ղ����i�i�ɍ��߂�B��L�̂悤�Ɏ����̂̎�ނ͂R��ނƂȂ������A����Ŏ����̐������v�U�̂ōςނ��A�X�̕K�v���͐�����ۂ��قȂ�B
�т��傫��120x360mm�ꍇ�̔j��ƁA�d�ό`�W������ƁA�j��͏\���ɕό`������ɁA���������̈ʒu�ŏc�ɗ�悤�ɔ����������Ƃ�������B105x360mm�̏ꍇ�ɂ͗��̂ق�����j�[�h�������̂ŁA���̌���������傤�ǔj�[�h���ς�鐡�@�������ƕ��������B������ɂ��Ă��\���ɕό`������̔j��Ȃ̂Ŗ��Ȃ����̂Ɣ��f�����B
�ʂ��ѐڍ��ɂ��~���ϗ͉͂d�Ɋ��Z����24.5kN�A�����̍~���ϗ͉͂d�Ɋ��Z����4.7kN�ƎZ�o�����B��]���������ɎZ�o����A�ʂ��ѐڍ��̍~������s����ƍl����ƃO���t�̑�Q�܂�_�܂ł����܂�B
�ő�ϗ͂́A�u�؎��\���ڍ����v�}�j���A���v�Œ�Ă���Ă���悤�ɁA�ʂ��ѐڍ��͍~����1.5�{�ɏ㏸����ƍl���A�����͏���������1/10�ɒቺ����ƍl�����B�����������ɂ��ϗ͂͏㏸���Ȃ����̂Ƃ��Ă݂��B
�ȏ�̌��ʕ`���ꂽ�O���t�́A�~�����̕ό`����⏭�Ȃ����̂́A�T�ˎ����l�̈��S����`���Ă���ƌ�����B�]���āA�u�ʂ��ѐڍ��v�Ɓu�����v�̑ϗ͂͒P���ɍ��Z���Ă悢���̂ƍl�����B
�Ȃ��A�u��������v�u�����Ȃ��v�̈Ⴂ�ɂ��ϗ͂̈Ⴂ�͎����Ŗ��m�Ɍ��邱�Ƃ��ł���B


�Ԍɂ̃t�B�[�����f�B�[���C���N�邽�߂ɃX�p���[���ɐ�����ڈႢ
�c�ނ̃s�b�`���ׂ������A�S�i�тɂ���ƃt�B�[�����f�B�[���ŃX�p��13.65m�̎Ԍɂ̉������˂��n�����Ƃ��ł���B�N���[�v��Œ�����4cm�ނ����Ă�������W���b�L�_�E���ł��傤��4cm����B���コ��ɕό`���i�s���Ă�6cm���x�ɗ��������Ƒz�����Ă���B
�u������K�v
���̌���̍Œ��A�h�C�c����C���^�[���V�b�v�ŗ��Ă����w�����A�h�C�c�ł͔��N�Ԃقnj���o��������C���^�[���V�b�v������Ƃ����B���{�ł͂ł��܂��ƕ����̂ŁA�ی��Ȃǂ̊W�œ���̂ł͂Ǝv�����̌���ɂ��肢���Ă݂���A�ӂ��Ԏ��ł����������������B���ǂQ���ԂقǓ��{�̑�H�d����̌����āA��ϊ��ŋA�����Ă������B����͗��w���Ɍ��炸�����Ǝ��݂���ƁA�w���ɂ�����ɂ��o���ɂ悢���ʂ�����悤�Ɋ������B
Ashikita Community Hall - Communication Center of Local-Resource-Utilization, 2010
Architect: Akiko Takahashi, Hiroshi Takahashi / Workstation
Structure: Jun Sato
Woven-like interlocked thin laminated timber structure inspired by bamboo baskets.
The timber bands can be woven in various directions and the members follow a geodesic line of surface.



Sashiki Elementary School in Ashikita, Kumamoto, 2006
���k�������~���w�Z
�v�H�@�F2006�N
���z�ƁF��R�v�{�Έ�Ђ�݁^Architerrace
�\���@�F�����~�\���v������
�X�M���u�ۑ��v���\���Ɏg�p����2�K���čZ�ɁB�Ǘ����̑��ړI�z�[���ɂ͖ؑ��h�[���������˂����Ă���B
����2m�s�b�`�ŗї����A���Ԃ̕����𗘗p�������`�X��̗��ƂƂ����u���[�����\���v���`������B
����ɂ���čZ���L���ɖʂ���ǖʂɃu���[�X��ǂȂǑϐk�v�f���K�v�Ȃ��A�J���I�ɂ��邱�Ƃ��ł���B
Cedar log columns and lattice beams are composed into rahmen structure.
�S�̖͌^�C���ρC���ړI�z�[������
�R�����A���ǂ��̌v��ł��uE70 �� 60 �` 80 tf/cm2�v���w�肵�Ă���B�a������ 350 mm���w�肵�A������K�����x�̍������ƂɌa���w�肵�Ă���B�ő� 10.5 m �Ƃ��������ނ��g�p���邱�Ƃ��ł����B
���́C��ނ��C���R�����C���Œ蒌�r�̎���
�؍ނ̒��B�͒��H�̔��N�ȏ�O�A�v�r������i�����n�߂Ȃ���Ȃ�Ȃ��B
�u���́v���u�t�͂炵�v���u��ނ��v���u�����v���u���݁v���u�����v
�̍H�����o��B
���̌v��ł́A�����\���Ȋ������Ԃ��m�ۂł��Ȃ��������̂́A�S�̍H���̓��D�����O�ɖ؍ނB���邱�Ƃ��ł����H�L�ȗ�ƂȂ����B
�����̋��E�̕ǂɂ��u���[�X��݂��A���a�����̑ϐk�v�f�Ƃ��Ă���B
�������̍\�����}�C���g�}�C�ڍ����ڍא}
�� Free Form Using Excavated Ground
Maison Owl – House & Restaurant, 2022
Architect: Junya Ishigami
Structure: Jun Sato
Excavated ground is useful for free-form formwork.
When the soil could be removed after the concrete could be hardened, the caved space could be revealed.


Maison Owl – House & Restaurant
https://www.pen-online.jp/article/011800.html



Excavated ground and rebar works
�� Structural Analysis Model Tune-up
Different Brick, Exhibition Real Size Competition 2013
Design & Construction: Yusuke Obuchi Lab, the University of Tokyo
Structural Adviser: Jun Sato Lab
Masonry structure composed of ellipse shaped bricks.
The bricks are cast using cone shaped moulds. The moulds were soft enough to be deformed, so different ellipses could be generated from the same mould.
Ellipse packing is a very complicated geometric problem which is solved by finding the solution of simultaneous quartic equations. These are developed using the conditions that the length of circumference must be identical and every adjacent 2 ellipses should have single intersection. Here we proposed an approximate solution.
�����ޗ��̉~����̌^�ō�����ȉ~�u���b�N��ϑw����B�����^�ł������ׂ���ɂ���ĈقȂ�ȉ~�u���b�N����邱�Ƃ��ł��A�������ׂċȖʂ��`������B�ڕW�̋Ȗʂ���邽�߂ɂǂ�ȑȉ~�����悢���m��K�v������B
���R�Ȗʂ��ȉ~�[�U����������B
�u�ȉ~�̎������v
�u�ȉ~���m�̌�_�̐����P�v
�̏����̂��ƁA�S�����������\�z����B�S���������̉��̌����ł͉����Ȃ����ɂȂ�A������ƕ����������A�ߎ������������Ƃ͂ł����B
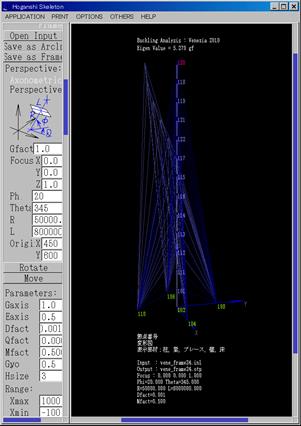

Not every global shape can be composed. This limitation should be considered as a characteristic of this system.
Another estimation other than those mentioned above will be the compression state, which necessitates that the final shape should be developed with no tension arising.
In this case a feedback operation is complicated to compose, but as we could develop an approximate algorithm of the relationship between the local state and the target curvature of the global shape, we could develop the growth , feedback and iteration processes.
DFL Pavilion 2013
Collaboration with Obuchi Lab (G30 Digital Fabrication Laboratory)
Tensegrity structure composed of X shaped components work as compression element and cables work as tension element.
�v��@�@�F������w�����S����� (G30 Program)
�\�����́F������w�����~������
�w�`�̃R���|�[�l���g�i���k�ށj���P�[�u���łȂ��A�h�[����ɖc��܂���ƌ݂��̃M���b�v�ɂ���ăf�v�X�����܂�A�P�[�u�����s���ƒ�����B���k�ޓ��m���ڐG���Ȃ��A�u�e���Z�O���e�B�v�̈��B


Geometric non-linear analysis is necessary for this structure because it stabilizes after large deformation such as 47 cm at its center.
�ȗ��������A���͂��\�����������܂ł̉ߒ�����ό`����ƂȂ�A�w�I����`������K�v�ƂȂ�B�����̕ό`�\�z��47cm�ƂȂ����B

�ɒ[�ɍ����̈قȂ镔�ނ����݂���ꍇ�̔���`��͎͂������ɂ����B���̉�͂ɂ��A��3mm�̃P�[�u�����s���ƒ���ƊT����6mm�̊ۍ|�Ɠ����̍d���̕��ނƉ��߂���Όv�Z���������₷���A���������Ȃ��ςނ��Ƃ����������B�R���|�[�l���g�ɔ������鈳�k�� 0.4 tf ���x�A�P�[�u���ɔ������钣�͂� 0.3 tf ���x�B


Compression test for a X shaped component, Tensile test for a cable joint
The X shaped component consists of 3 stainless steel plate of 0.3 + 0.8 + 0.3mm thick.
Ultimate compression strength of this component has found 0.41 tf.
�R���|�[�l���g�̈��k������ 200 tf �����@���g�p�A�P�[�u���̈��������̓e�R�����p���ăo�l���ōډׂ����B
���k�����œ���ꂽ�ő�d�͗Ⴆ�� 0.41 tf �ȂǁB
������Č��ł���悤��̓��f���̐ݒ������B���͎��ۂƓ��� 0.3 + 0.8 + 0.3 mm �Ƃ��A���ޕ��� 40 mm �Ɛݒ肷������d�� 0.29 tf �ƂȂ�A�T�ˍČ����Ȃ��班�����S���̎Z�肪�ł��邱�Ƃ����������B


Once it is found possible to use 40mm wide elements for these grid model, we can find the strength of other options only by the analysis without loading tests.
���f�����̕��j��������Α��̌`��̋��x��A���x�s���̃R���|�[�l���g�̑Ώ��@����݂͂̂Ō����邱�Ƃ��ł���B���� 0.5 + 1.2 + 0.5 mm �Ƃ�������d�� 0.758 tf �ƂȂ�\���Ȃ��ƂȂǂ�������B
�a���`�͍����ɗL���Ȍ`��ł���A�R���̌��������v�����P�����x�̍����������ł��邱�Ƃ����������B
���ꂾ���������ėՂ�ł��A����ł͐��X�̖�肪�N����B
�݂�グ�̍Œ��ɃR���|�[�l���g���Ȃ���A�R���|�[�l���g���c��݂����Ă��Ȃ����̂�������A�P�[�u���̒��肪���܂��̂�������B���̏�ɂ���ޗ��ō��ǂ�ȑΏ����\���A�u���̔��f���K�v�ƂȂ�B���������Ƃ��̐E�l�����͗��������B

Construction
������Ȃ����Ƃ��炯�̒��A����ꂽ���Ԃ̒��ʼn�����������ꂪ���ƕ����邩�A�ɂ߂ĒP���ȃ��f���ɕ����ׂĂ�������ɂ߂�A�\���G���W�j�A�̋Z�����s����ǂ��@��ƂȂ����B
���̌`�Ԃł́A�ȗ��ƃR���|�[�l���g�̑傫���ɂ���ăf�v�X���R���g���[�����邱�Ƃ��A�Ȗʂ̋��x���R���g���[�����邱�ƂɂȂ�B���̊W����ǂ̂悤�ȋȖʂ����ݏo���邩�Nj����邱�Ƃɂ����p�ł���B
Poured Sticks Structure in DFL Pavilion 2014
Obuchi Lab, G30 Digital Fabrication Laboratory
Sticks are connected in simple way just touching. But they have the parameter of 3D angle and connection point, which makes the geometry complicated.
To make the problem simple, poured sticks are interpreted as the porous volume material.
�v��@�@�F������w�����S����� (G30 Program)
�\�����́F������w�����~������




Zoom out from detail
Finally the way of calculation was found different from Sunny Hills, structure of complicated Kigumi joint.


Sunny Hills Japan, 2013, Architect : Kengo Kuma
Structural analysis model for Sunny Hills, composed with every timbers modeled into bar elements.
Compression Test
Left : Initial 3 specimens
Middle : Improved 2, provided 2 by Shimizu Co.
Specific Gravity ��= 0.06 tf/m3
Young�fs Modulus E = 1.0 kgf/cm2
Yield Stress ��y = 0.108 kgf/cm2

Bending Test
Specific Gravity ��= 0.04 tf/m3
Young�fs Modulus E = 0.6 kgf/cm2
Yield Stress ��y = 0.209 kgf/cm2
Representing values for structural analysis :
|
|
Specific Gravity |
Young�fs Modulus |
Yield Stress |
|
|
[tf/m3] |
[kgf/cm2] |
[kgf/cm2] |
|
Urethane Sponge |
0.300 |
0.4 |
- |
|
Poured sticks |
0.060 |
1 |
0.108 |
|
Styrene foam |
0.013 |
80 |
1 |
|
Balsa wood |
0.140 |
40000 |
160 |
|
Cedar wood |
0.380 |
80000 |
400 |
|
Steel |
7.800 |
2100000 |
5000 |
Even the material was found soft and weak, still we can use it for structure with thick volume.


Structural analysis for the mock up, gravity and wind speed 10 m/sec loaded.
Operated by Mika Araki, Jun Sato Lab
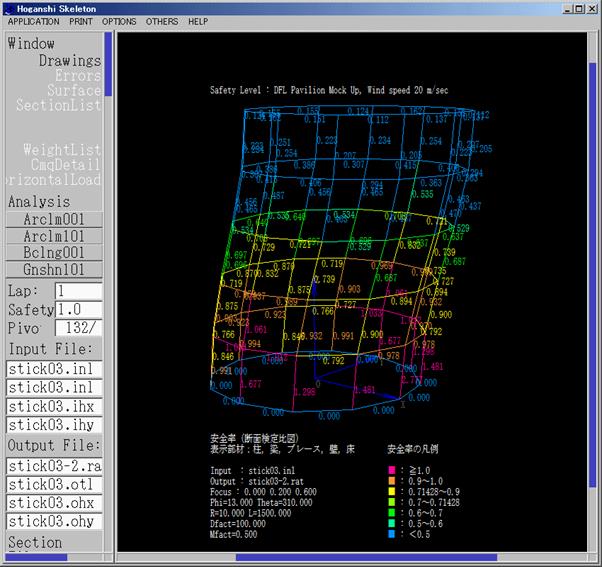

Structural analysis for the mock up, gravity and wind speed 20 m/sec loaded.
Red elements indicating the lack of strength.
Operated by Mika Araki, Jun Sato Lab

Structural analysis and Optimization progress for final shape.
Operated by Mika Araki

Interface for adjustment of Karamba+Grasshopper to Hogan
Developed by Masaaki Miki


Stainless cables inserted in the wall for extra safety.
They work as tension rings.
�� 3D Tensegrity / Pop-up Tectonics
3�����e���Z�O���e�B / ��яo���G�{
���k�ޓ��m���ڐG���Ȃ��\���B�L�`�ɂ͎��]�Ԃ̎ԗ֏�̂��̂��܂߂���B
���k�_�ƈ����̃P�[�u���ނō\������A���k�ޓ��m���ڐG���Ȃ��\���B�L�`�Ɉ��k�ޓ��m���ڐG���Ȃ��\���S�ʂ��w���A���]�Ԃ̎ԗւ̂悤�Ȃ��̂��܂܂��ꍇ������B
Kenneth Snelson�CBuckminster Fuller �ɂ��L�߂�ꂽ�B
�͌^����ł��獢��ɂ߂�B����������i���k�ނ�lj�����j��@���d�v�B
���k�ނ���莩�R�Ȍ`��Ƃ���A�����ނɂ���A�ȂǑ��l�ȃo���G�[�V�������l�Ăł���B
���莟���ɂ�镪�́A�Q�_��p�����Ώ̐��̕��́A�ڑ�������s��\�����邱�Ƃɂ���͖@�A���Ђ����ɒ��ڂ��Ĉ����Ԃ����߂��͖@�A�Ȃnj�������Ă���B



3D Tensegrity �� Experiments on Geometries and Dynamics : workshop at Stanford University
Experiments on Geometries and Dynamics: workshop at Stanford University, 2014
Lecturers
Beverly Choe, Architect, BACH architects / Stanford University
Jun Sato, Structural Engineer, Jun Sato Structural Engineers / University of Tokyo
Pop Up Structure and 3-dimensional Tensegrity Structure were studied in 2 days and constructed in 2 days.
�X�^���t�H�[�h��w�ł̃��[�N�V���b�v
2014�N�C�X�^���t�H�[�h��w�ł̃��[�N�V���b�v
�ޗ��ƃJ�e�S���[�������炩���Ă��A�Q���̂Q���ԂŌ`��̃X�^�f�B���s���A�T���̂Q���Ԃō\�z�����B
Category 1, Tensegrity Volume : Tensegrity to have 3D volume.


3D Tensegrity Volume composed of 18 galvanized bars and lengths of stainless cables (Photo by Nick Xu)
Tensegrity model, Pop-up Tectonics model
�e���Z�O���e�B���R�����̍L������������鎎��
��яo���G�{�̂悤�ɐ܂肽���߂�\���̎���
Dimensionality of Tensegrity
It is hard for a basic tensegrity to find a stable shape as a �g3 dimensional�h volume with not a modular system.
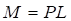
�e���Z�O���e�B�̋�ԓI�ȁu�����v
�P�����I�ȃ^���[��̂��́A�Q�����I�ȃh�[����̂��̂Ȃǒ�Ă���Ă���B
�R�����I�ȃ{�����[������������ɂ́A����ɍ���ɂ߂�B
Category 2, Pop-up Tectonics: Foldable structure like a pop-up book, composed of 22 panels made of washi, traditional Japanese paper, and timber frames.
Washi paper provided by Takeo Co., Ltd.
Echizen Washi production: Shimizu Washi Co., Ltd.




Raising process of Pop-Up Tectonics,
(Top right, bottom left: photo by Nick Xu)
(Top left, bottom right: photo by Jun Sato)
It is hard to find an exactly foldable shape when using thick plates.
Extensions of sides should cross at the same focus point.
Panels belonging to the same layer should not be overlapped when they are folded down and the total angle of the sets of panels, which coupled, should be same.

Geometrical conditions can be recognized by studying the model.
For example, from the top view, a ridge line or thalweg line should be seen to lie on a straight line.
When the loop is connected, panels have twisted shape like a Mobius loop and it is hard to find the focus point.



Pop-up Stained Glass using brass frames, Workshop 2012, Jun Sato Lab, The University of Tokyo
Rigid Origami: deployable geometry composed of rigid thick panels
�u��яo���G�{�v�\���̃X�e���h�O���X���̃C���X�^���[�V����
�� Developable Surface
�W�ʂ̃R���g���[���@
�ʂ̐L�k�Ȃ��W�J�ł���u�W�ʁv�̃R���g���[���@�̌������J�n�B
�܂��A�W�ʂ���ӂɌ��肷�邽�߂̃p�����[�^�[��T�����B






Venezia Biennale 2018 �̂��߂̑я�̃K���X�C���X�^���[�V�����̒��
����ނɌ��肵���`���A�˂ĕ��G�ȃ��{����̌`�Ԃ��\������
GA gallery �W����o�W�B
�� Reciprocal System
Rest house in the Forest
Seminar at Keio University, 2006
Free form shell structure composed of 19x140mm timbers. Each element is leaning on another element, mutally continuing. This system is called lamellendach or reciprocal system.
�X�̋x�e��, 2006�N, �c��`�m��w��w�@��
19�~140mm�̃c�[�o�C�ނ��A�݂��ɍڂ������Ȃ��玟�X�ƃo�����X���čL����A�傫�ȋ�Ԃ��˂��n���B
���Ƃ��������ˍ\(Lamellendach)�ƌĂ��V�X�e������̔��z�ł��������A�w�������ɂ���ėl�X�Ȋi�q�̃p�^�[�����N���̎��R�x���������ꂽ���ʁA���̂悤�ȎO�p�`�ƘZ�p�`���A�Ȃ�͗l�Ɏ������B
�ׂ��ȍނ�����Ԃ�������(Lamella, Lamellen)�ƌĂсA�Z���ނ����݂��Ɏx���������ƂŐ�������Ƃ����Ӑ}������A����̓������ˍ\�̈��ƌ�����B
�� Workshops & Exhibitions
���[�N�V���b�v�X�P�[���ł̎��H
���K�͍\�z����v�{�H���郏�[�N�V���b�v���u�v�@�v�̍\�z��Z���Ԃő̊�����ǂ��@��ƂȂ��Ă���B
����ꂽ���ԂƔ�p�̒��Ō��f���Ȃ���Ȃ�Ȃ��G���W�j�A�����O�̎�@���w�ԏ�Ƃ��Ȃ��Ă���B
�u���Ă����ȂȂ��\���v�̒Nj��ɂ��Ȃ��Ă���B
Creative Structures: art4d workshop in Bangkok, 2012
Using local materials, 4 teams constructed pavilions of 4 to 8 m spans, in 2 days.











French Braid Tectonics
State of the Community 2016 / CITIZENS, COMMUNITIES and MULTILAYERED IDENTITIES, Dhillon Marty Foundation
Jun Sato Lab, University of Tokyo
Marc Dilet and students, Ecole Nationale Superieure d�fArchitecture Paris - Val de Seine


French Braid Tectonic, Workshop in the State of Community 2016 conference
The copper wire, with a diameter of 3mm, was braided to form French Braid Hair.
Metal wires are useful to compose quite ductile structure. Even in the event of collapse, it won�ft fall down suddenly, rather shows a soft landing, because of the ductility.
Referring to a following Nebuta Tectonic project, when the slight wires are composed into 3-dimensional framework, the buckling length can be reduced and reinforced. In this case Washi paper works as tensile bracing.


Nebuta Tectonic: Steel wire + Washi paper, Jun Sato studio 2015 (Photo: Ying Xu)


Traditional Pampas grass braiding, metal wire braiding in Okinawa, Japan
Gecko and Cicada produced by wire artist Hironori Hashi
����ɓ`��鑐�҂�
�j���A�[�e�B�X�g���������̍�i
http://hashishiki.jugem.jp/?eid=1171
http://www.maruei.ne.jp/art-club/2010/08/post_220.html
Metal wires are also useful for developing fastener pieces. It is easy to develop the shape of interlocking, slotting, hooking or bouncing as following images.



Carbon Cable Pavilion for the Housevision Exhibition 2016, Architect: Kengo Kuma
Custom formed pad and fastener
French braid form tells how to compose bunches and how to cross at many points for metal wires to become structural elements.

French braid formed wires, Samples of braiding tecnique
Even though copper is weaker than steel, it is one of the materials which we can enjoy the aged surface turned into dark brown or green. Copper has enough ductility, which means ability to deform, and absorb energy due to the loads.



Overall form resulted in a ellipse dome composed of mingling arches.
Dimensions of the dome part of the pavilion : 3m long�~2m wide�~1.5m high
Water Pavilion, World Exhibition in Aichi 2005
Design & Build: Naomi Sakuragi + Postgraduates of the University of Tokyo
Booth composed of dimpled acrylic resin walls. Thin plate wall comes 4 to 6 times stronger when dimpled.
���m����2005�W���u�[�X : 2005�N�C���z�Ɓ��N�ؒ����{������w��w�@��
�����o�C�I�ŏ���c�̂̓W���u�[�X�B
����3mm�̃A�N�����������_�������ʏ��ɓʉ��������ǂ��\���Ƃ��Ă���B
�����A���ʏ�ɓʉ�������ƁA4�`6�{�̋��x������B
�A�N�����̗n�Z���x���Ⴂ���Ƃ𗘗p���āA�o�[�i�[�ʼn��M���A���i�x�������t����Ƃ������@�Ő��삵���B
�ǂ��������ɗ��Ă邱�Ƃɂ��A����ɋ��x�𑝂��Ă���B
Dimpled acrylic resin wall, Forming process using Chinese pan


Physical model made of aluminum, Buckling analysis
�A���~�ō�������x�����p�͌^�C�������
Moulded Pulp Installations: Seminar for master course of Tokyo University of Science
�p���v���[���h : �������ȑ�w��w�@�u�`, 2010�N
���𐅂ɗn���A���^�Ő��`����B�y�ʂŎ��R�Ȍ`�\�B
�@�ۓ��m�����f�����݂̂Őڍ�����Ă��邽�߁A���ɐZ���ƕ������A�ė��p�ł���B


����1.8m�́u�V�F���^�[�v, ���g�Ɏ��t������u��q�v
Komorebi Workshop in Paris, 2018
ENSA Paris Val de Seine �Ƃ̃��[�N�V���b�v



���E�Ŕ��{�Ōy�ʂ̕z�u�V���̉H�� Super Organza�v�̖������^���̍��g�œ˂������Ĉ��肳����\���̒��
�t�����X�̌��z����ł́A�I�[�K�j�b�N�ȑf�ނ��g�����Ƃ��d�����Ă���B


�������ȐF���܂�d�Ȃ镗�i
���ݏo�����i�`�����������u�Q�����X�y�N�g���v�ŕ��͂���\��
Digital Fabrication Laboratory Pavilion 2015
������w�����������iG30�j�ւ̋���



���̌`�Ԃ̌����̂��߂ɁA
Rhinoceros + Grasshopper �� Hogan (Released by Jun Sato)
�Ƃ������Ŏ������s�����`�ԉ�̓\�t�g�E�F�A�̃R���|�[�l���g���\�z�B
Student Summer Seminar 2015, ���{���z�w��
�J�[�{���P�[�u���iCFRP �P�[�u���j�ɂ�閚�̂悤�ȃh�[���\���̒�āB
�u�D�G�܁v����܁B

�u��炮�t���[���v, �l�āF�G ���� �{ �F�J �B�T�C���́F�����������w���ꓯ
�ʐ^�Fhttps://www.aij.or.jp/jpn/symposium/2015/sss2015photo.pdf
Real Size Competition �o�W��i�ւ̋���



GA Gallery �W����
���z�v�n�̎G���uGA JAPAN�v��Â̓W����B
Leoflex ���g�����K���X�\���̕������b�N�A�b�v�AVenezia Biennale 2016 �̂��߂̉W�ʂ̃X�^�f�B�͌^���o�W�B

Leoflex �ɂ��\�����b�N�A�b�v
�� Educational Keywords
�EMaterial properties �ޗ�����
��d density / unit weight (��)
���� stiffness (K)�C�����O�W���i�����O���C�e�����jYoung�fs modulus (E)
�|�A�\���� Poisson�fs ratio (��)
�e�� elastic�C�Y�� plastic�C�S�e�� viscoelastic
�~�� yield
���x�i�����C���k�C�Ȃ��C����f�jstrength (tensile, compression, bending, shear)
�I�Nj��x ultimate stress
���` linear�C����` nonlinear
�L�ѐ��\ ability of elongation�C���� ductility�C�Ր� toughness�C�Ɛ� brittleness
���c���W�� linear expansion coefficient
�EStress - Strain Curve ���͂Ђ��݊W
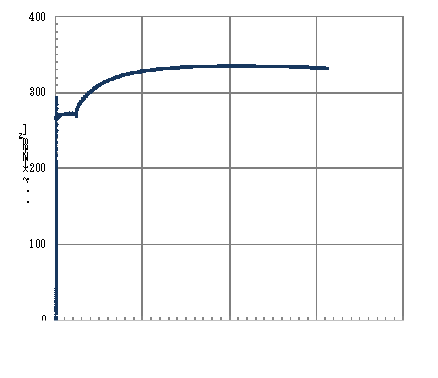
�|�� SS400 �̈�������
Tensile Test of Steel SS400
�ELoad - Displacement Curve �d�ό`�W

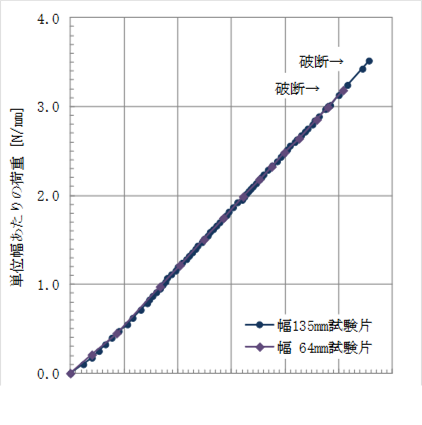
Typical Load-Displacement Curve �K���X�i���ʋ��x�j�̋Ȃ�����
�Y���悪�S�����݂��Ȃ�
Bending Test of Glass Plate
Glass has no ductility
�ESection Properties �f�ʐ��\
�f�ʐ� Area A [cm2]
�f�ʂQ�����[�����g Moment Inertia I [cm4]
�˂���萔 St. Venant�fs Torsion Factor J [cm4]
�f�ʌW�� Section Modulus Z [cm3]
Example of rectangular section B x D
A = BD
I = BD3/12
Z = BD2/6
�EStructural Calculation �\���v�Z
��{����
�������ʂɂ���āA�ޗ����x�A�����O���A���������f�ʐ��\�i�f�ʌW���y�C�f�ʂQ�����[�����g�h�Ȃǁj�A���������A�ȂǎZ�o����B
�����̎��́A�ˍ\��P�����������f�����C���[�W���Čv�Z����Ƃ��ɂ��𗧂B
Basic formulae
These formulae will be also used for simplified calculations, understanding structural test, etc.
�E�o�l�̉d�ƕό` Load – Displacement on spring
F = k x
where
F : �d force (load)
k : �o�l�萔 factor (stiffness) of spring
x : �ψ� displacement
�E�e���Ȃ����[�����g Bending moment in elastic level
M = �� Ze
where
M : �Ȃ����[�����g bending moment
�� : �����̉��͓x stress on edge
Ze : �f�ʌW�� elastic section modulus, for rectangle section Ze = BD2/6
�E�S�Y�����[�����g Bending moment in plastic hinge level (ultimate level)
Mp = ��y Zp
where
Mp : �S�Y�����[�����g plastic hinge bending moment
��y : �~�����͓x yield stress
Zp : �Y���f�ʌW�� plastic section modulus, for rectangle section Zp = BD2/4
�E�W���d����P���� Simple beam with concentrated load
bending moment ![]() displacement
displacement ![]()
�E�����z�d����P���� Simple beam with uniform distribution load
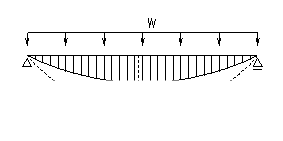
bending moment ![]() displacement
displacement ![]()
�E�����z�d���闼�[�Œ�� Fixed beam with uniform distribution load
bending moment ![]() displacement
displacement ![]()
�E�W���d����Ў��� Cantilever beam with concentrated load
bending moment ![]() displacement
displacement ![]()
�E�����z�d����Ў��� Cantilever beam with uniform distribution load

bending moment ![]() displacement
displacement ![]()
�E�I�C���[�����d Euler�fs Buckling Load

fix-fix fix-hinge hinge-hinge fix-fix+sway fix-hinge+sway
��=0.5 ��=0.708 ��=1.0 ��=1.0 ��=2.0
Buckling strength : Pcr = ![]()
E : Young�fs modulus [tf/cm2]
I : Moment inertia of section [cm4]
Lk : Buckling length Lk =��L
�E�A�[�`�̌`�� Arch Shape

�����z�d�ɑ��Ă͕�������`�� Parabola for uniform distribution load
![]()
���d�ɑ��Ă̓J�e�i���[��`�� Catenary for self-weight load
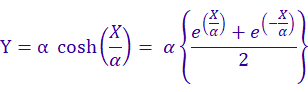
�E�A�[�`�̗��Z approximate calculation

Approximate Buckling Length Lk = 0.4 〜 0.5 L
������ ![]() �ŋߎ�����B
�ŋߎ�����B
���́A�X�p�� L �ƍ��� H �ɂ��A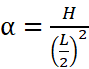 �ŋ��߂���
�ŋ��߂���
�X���͔������āA![]() �Ȃ̂ŁA�[���ł̌X���́A1.0 :
�Ȃ̂ŁA�[���ł̌X���́A1.0 : ![]() = 1.0 :
= 1.0 :  �ƂȂ�B
�ƂȂ�B
�����d�� w [�P�� tf/m �Ȃ�] �Ƃ���ƁA�x�_�̉������� ![]()
�[���̎��� N �́A�X�������Ȃ̂ŁA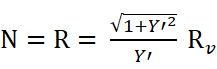
����ɑ��A�A�[�`�̍����d�́A���������� 0.4 L �ȂǂƂ��ăI�C���[�����̎��ɑ�����ċ��߂�B
�EBasic Process of Structural Analysis
�ˍ\�S�̂̍\����͂̊�{�菇
(1) �ޗ��ƌ`��̑z�� Assumption of Material Property, Shape of Members and Shape of Frame
(2) �d�̑z�� Decision of Loads as its Type and Level
Load Type: Gravity, Earthquake, Wind, Temperature
Load Level: Elastic / Ultimate
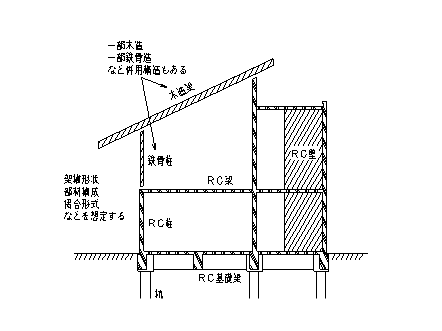
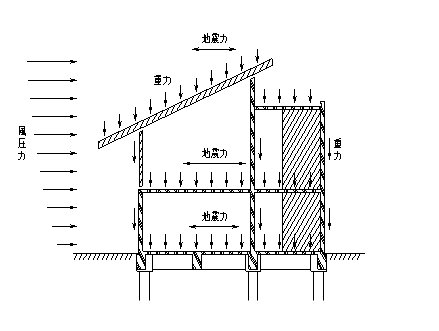

�����ɉ����l�X�ȉd�̗�
Diagram of diverse loads
�d Concerning Load
�d�� Gravity : �d�͉����x 1G = 980 cm/sec2
�Î~���Ă���l Stationary person = 80 kgf
�K�i���~���l Person descending stairs = 200 kgf
�n�k�d Seismic Load : �U����ÓI�d�Ɋ��Z������@������B���\�N�ɂP�x�̒n�k�ŏd�ʂ� 0.2 �{�ȂǁB
Seismic vibration load considering a couple of decades in Tokyo can be interpreted to 0.2 of factor of weight.
���d Wind load : �����̌����ł͐��\�N�ɂP�x�̕����i10�����ρj�Ƃ��� 34 m/sec ��z�肵�T�� 100 kgf/m2 �ƂȂ�B
�����͕͂�����2��ɔ�Ⴗ��B
�������ł� 20 �` 25 m/sec
���T�Ԃł� 10 �` 15 m/sec
�ȂǂƑz�肷��B
Wind pressure considering a couple of decades in Tokyo can be estimated to be 100kgf/m2 due to the wind speed 34m/sec (10min. average speed).
Wind pressure is proportional to the square of wind speed.
20 �` 25 m/sec for several months
10 �` 15 m/sec for several weeks
(3) ���f���� Modeling for Analysis
Finite Element Method (FEM)
Element Type: Bar element (Wireframe), Plate element, Solid element
Joint Type: Rigid, Hinge (Pin), Half rigid (Spring)
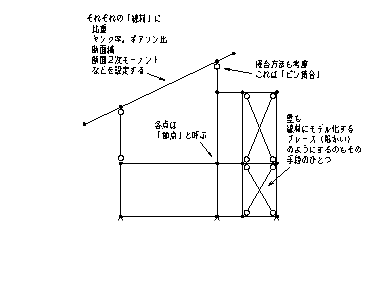

(4) �\����� Calculation
Results: Deformation, Stress

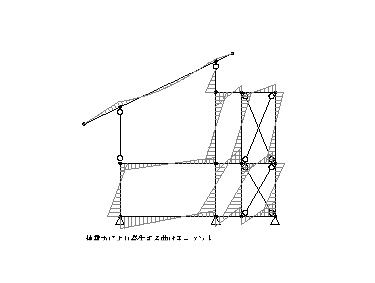

Bending Stress Diagram, Deformation Diagram
(5) ���x�̃`�F�b�N�i���ޒf�ʐv�j Analysis of Stress
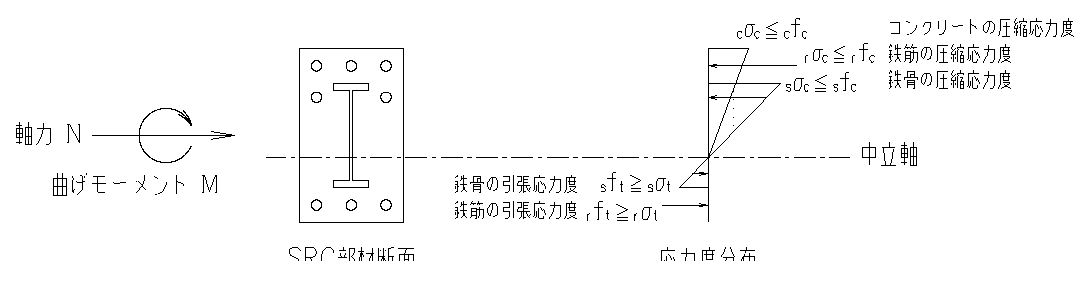
Basic Case
Safety Ratio = N/Na + M/Ma �� 1.0
N: axial force
M: bending moment
a: allowable
Structural analysis software HOGAN
Safety Ratio = M/Ma / (1.0 - N/Na) �� 1.0
�� Technical Key Words
�œK���^�`�ԉ�́^�`�ԑn��
�S�̍œK���^�Ǐ��œK��
���ړI�œK���^�����œK��
�����ŗL�l���̏k��
������с^2�����X�y�N�g�����
���R�`��^Fuzzy Node�^�ؑg
�p�����g���b�N�f�U�C���^�f�W�^���t�@�u���P�[�V����
��H�̋Z
�G���W�j�A�����O�͏ȗ��̋Z
���Ă����ȂȂ��\��
���ʊ�n�^�ΐ���n
�� International Seminar & Workshop
Seminar at Norwegian University of Science and Technology NTNU
Kigumi Workshop at Universität für angewandte Kunst Wien, Die Angewandte
Workshop with ENSA Paris Val de Seine
Seminar at Stanford University
Transparent Structure as Perceptual Filter: Seminar and Workshop at Stanford University, USA, 2015
Experiments on Geometries and Dynamics: Stanford University, USA, 2014
Workshop at Harvard GSD
Seminar at SCI-Arc
Seminar at RISD
Workshop in Paris
Community Week 2014: Dhillon Marty Foundation International Workshop in Amritsar, Punjab, India, 2014
Workshop in Bangkok
Creative Structures: art4d Workshop in Bangkok, Thailand, 2012
�� Lectures
November 4th + 5th 2019: Lecture on timber structure in Vietnam
March 21st + 22nd 2019: Lecture for Norwegian University of Science and Technology NTNU
October 16th 2018: Lecture for Universität für angewandte Kunst Wien, Die Angewandte
October 9th 2018: Lecture for ENSA Paris-Val de Seine
November 1st 2017: Lecture for SCI-Arc
October 27th, 2015: Lecture for Rhode Island School of Design RISD
October 26th, 2015: Lecture for Harvard GSD
April 30th, 2015: Archi-Neering Design AND in Nanjing
October 27th, 2014: Lecture for University of Oregon
September 19th, 2014: Keynote Speech for Smart Geometry in Hong Kong
�� Publications
MARK #54, Exacting Structures, Netherlands, 2015
Earth: Material for Design, National Museum of Emerging Science and Innovation, Japan, 2011
Items in Jun Sato Structural Engineers, LIXIL Publishing, Japan, 2010
GLASS BLOCK and ARCHITECTURE, Crystal Brick House, Alinea Editrice, Italy, 2010
Creating New Principles for 21st Century Architecture, LIXIL Publishing, Japan, 2009
Vivid Technology, Gakugei Publishing, Japan, 2007
Copyright © 2010 Jun Sato Laboratory